5 Ways to Master Triangle Angle Sum Theorem
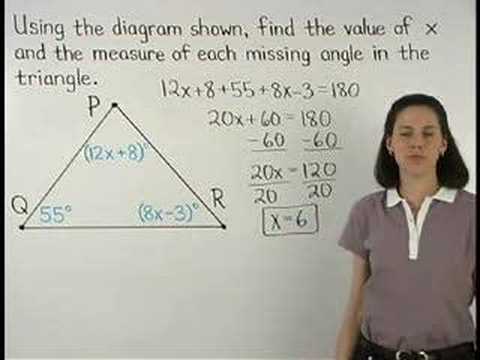
Unlocking the Secrets of the Triangle Angle Sum Theorem
The triangle angle sum theorem is a fundamental concept in geometry that states the sum of the interior angles of a triangle is always 180 degrees. This theorem has far-reaching implications in various fields, including architecture, engineering, and physics. Mastering the triangle angle sum theorem can help you solve complex problems and deepen your understanding of spatial relationships. In this article, we will explore five ways to master this theorem and unlock its secrets.
1. Understanding the Basics
Before diving into advanced applications, it’s essential to understand the basics of the triangle angle sum theorem. The theorem states that for any triangle, the sum of the three interior angles is always 180 degrees. This can be represented mathematically as:
α + β + γ = 180°
where α, β, and γ are the interior angles of the triangle.
To reinforce your understanding, try solving simple problems involving triangles with known angles. For example:
- Find the third angle of a triangle given that two angles are 60° and 80°.
- Determine the sum of the interior angles of an equilateral triangle.
Table: Examples of Triangle Angle Sums

| Triangle Type | Angle 1 | Angle 2 | Angle 3 | Sum of Angles |
|---|---|---|---|---|
| Equilateral Triangle | 60° | 60° | 60° | 180° |
| Isosceles Triangle | 70° | 70° | 40° | 180° |
| Right Triangle | 90° | 60° | 30° | 180° |
📝 Note: These examples illustrate the versatility of the triangle angle sum theorem in different types of triangles.
2. Visualizing Angles
Visualization is a powerful tool for mastering the triangle angle sum theorem. By drawing triangles and measuring their angles, you can develop a deeper understanding of the theorem’s implications.
- Draw a triangle with angles α, β, and γ.
- Measure each angle using a protractor or angle measurer.
- Calculate the sum of the angles to verify that it equals 180°.
To enhance your visualization skills, try using digital tools or software to create interactive triangles. This will allow you to experiment with different angles and explore the theorem’s properties in a more engaging way.
3. Applying the Theorem to Real-World Problems
The triangle angle sum theorem has numerous applications in real-world problems. By applying the theorem to practical scenarios, you can develop a deeper understanding of its significance.
- Design a bridge with a triangular support structure. Use the theorem to ensure that the angles are optimized for maximum stability.
- Calculate the angles of a triangular roof to determine the best placement for solar panels.
- Determine the interior angles of a triangular garden bed to optimize plant growth.
By applying the theorem to real-world problems, you can develop a deeper appreciation for its relevance and importance.
4. Exploring Angle Relationships
The triangle angle sum theorem is closely related to other angle relationships in geometry. By exploring these relationships, you can develop a deeper understanding of the theorem’s underlying principles.
- Investigate the relationships between angles in similar triangles.
- Explore the properties of complementary and supplementary angles.
- Analyze the relationships between interior and exterior angles of a triangle.
By examining these relationships, you can develop a more comprehensive understanding of the triangle angle sum theorem and its role in geometry.
5. Practicing with Word Problems
Word problems provide an excellent opportunity to practice applying the triangle angle sum theorem in different contexts. By solving word problems, you can develop your critical thinking skills and reinforce your understanding of the theorem.
- Tom is designing a triangular shelf for his bookcase. If two angles are 50° and 70°, what is the third angle?
- A triangular prism has angles of 30°, 60°, and x. What is the value of x?
- A surveyor is measuring the angles of a triangular plot of land. If two angles are 80° and 40°, what is the third angle?
By practicing with word problems, you can develop your problem-solving skills and master the triangle angle sum theorem.
The triangle angle sum theorem is a fundamental concept in geometry that has far-reaching implications in various fields. By mastering this theorem, you can deepen your understanding of spatial relationships and solve complex problems. By following these five steps – understanding the basics, visualizing angles, applying the theorem to real-world problems, exploring angle relationships, and practicing with word problems – you can unlock the secrets of the triangle angle sum theorem and become a master of geometry.
What is the triangle angle sum theorem?
+The triangle angle sum theorem states that the sum of the interior angles of a triangle is always 180 degrees.
How can I apply the triangle angle sum theorem to real-world problems?
+The theorem has numerous applications in real-world problems, such as designing bridges, calculating roof angles, and determining garden bed angles. By applying the theorem to practical scenarios, you can develop a deeper understanding of its significance.
What are some common angle relationships related to the triangle angle sum theorem?
+Some common angle relationships include similar triangles, complementary and supplementary angles, and interior and exterior angles of a triangle. By examining these relationships, you can develop a more comprehensive understanding of the theorem.
Related Terms:
- Triangle Sum Theorem Worksheet PDF
- Triangle exterior angle Theorem Worksheet
- Isosceles triangle Theorem Worksheet