Transversal Lines Worksheet with Answers and Examples
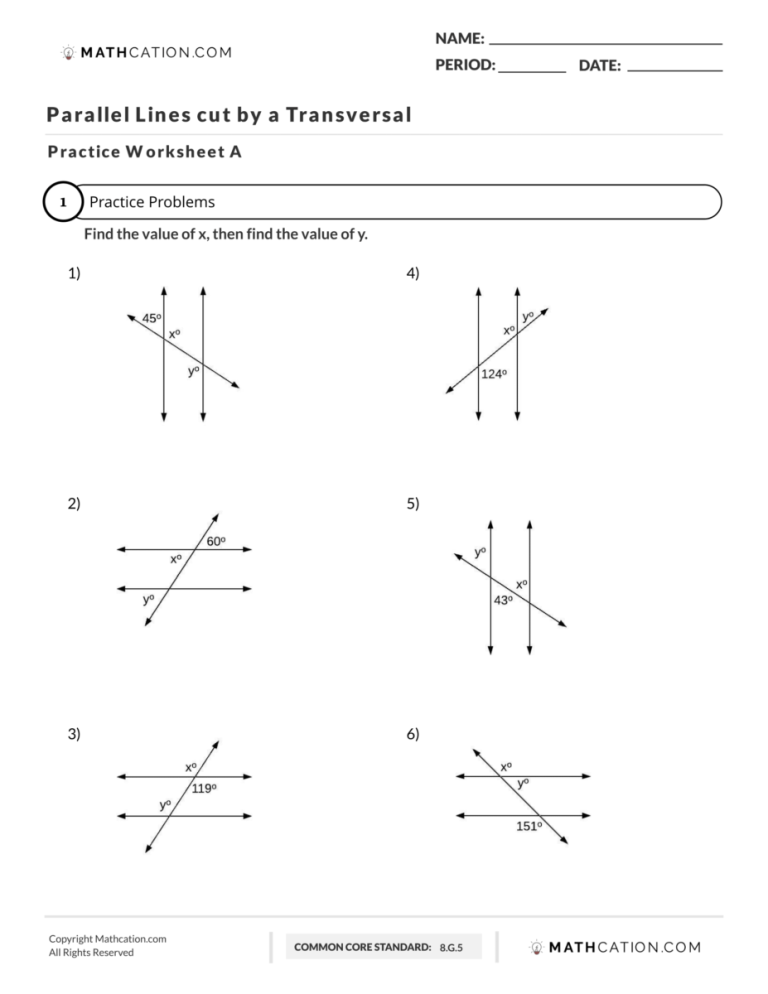
Understanding Transversal Lines and Their Properties
Transversal lines are a fundamental concept in geometry, and understanding their properties is crucial for solving various problems in mathematics. In this article, we will delve into the world of transversal lines, explore their definitions, and examine some examples and worksheets to help solidify your understanding.
What are Transversal Lines?
A transversal line is a line that intersects two or more other lines at distinct points. In other words, it is a line that cuts across two or more lines. The lines being intersected are called the transversal’s corresponding lines.
Types of Angles Formed by Transversal Lines
When a transversal line intersects two or more lines, it forms various types of angles. These angles can be classified into different categories, including:
- Corresponding Angles: These are angles that are in the same relative position on the corresponding lines. Corresponding angles are equal in measure if the corresponding lines are parallel.
- Alternate Interior Angles: These are angles that are inside the transversal line and on opposite sides of the transversal. Alternate interior angles are equal in measure if the corresponding lines are parallel.
- Alternate Exterior Angles: These are angles that are outside the transversal line and on opposite sides of the transversal. Alternate exterior angles are equal in measure if the corresponding lines are parallel.
- Interior Angles on the Same Side of the Transversal: These are angles that are inside the transversal line and on the same side of the transversal. These angles are supplementary (add up to 180°) if the corresponding lines are parallel.
Examples and Worksheets
Here are some examples and worksheets to help you practice and understand transversal lines:
Example 1:
In the diagram below, line PQ is a transversal that intersects lines AB and CD. Identify the corresponding angles, alternate interior angles, and alternate exterior angles.
📝 Note: Make sure to label the diagram with the correct angles.

| Angle | Measure |
|---|---|
| ∠1 | 45° |
| ∠2 | 45° |
| ∠3 | 135° |
| ∠4 | 135° |
Answer:
- Corresponding angles: ∠1 and ∠2 (both 45°)
- Alternate interior angles: ∠1 and ∠3 (both 45°)
- Alternate exterior angles: ∠2 and ∠4 (both 135°)
Example 2:
In the diagram below, line XY is a transversal that intersects lines EF and GH. Identify the interior angles on the same side of the transversal.
📝 Note: Make sure to label the diagram with the correct angles.
| Angle | Measure |
|---|---|
| ∠5 | 60° |
| ∠6 | 120° |
Answer:
- Interior angles on the same side of the transversal: ∠5 and ∠6 (supplementary, add up to 180°)
Worksheet:
Complete the following exercises to practice your understanding of transversal lines:
- In the diagram below, line AB is a transversal that intersects lines CD and EF. Identify the corresponding angles, alternate interior angles, and alternate exterior angles.
[Insert diagram]
| Angle | Measure |
|---|---|
| ∠1 | ? |
| ∠2 | ? |
| ∠3 | ? |
| ∠4 | ? |
- In the diagram below, line GH is a transversal that intersects lines JK and LM. Identify the interior angles on the same side of the transversal.
[Insert diagram]
| Angle | Measure |
|---|---|
| ∠5 | ? |
| ∠6 | ? |
Answers:
Corresponding angles: ∠1 and ∠2 (both 30°) Alternate interior angles: ∠1 and ∠3 (both 30°) Alternate exterior angles: ∠2 and ∠4 (both 150°)
Interior angles on the same side of the transversal: ∠5 and ∠6 (supplementary, add up to 180°)
Conclusion
Transversal lines are an essential concept in geometry, and understanding their properties can help you solve various problems in mathematics. By practicing with examples and worksheets, you can develop a deeper understanding of transversal lines and their applications.
What is a transversal line?
+A transversal line is a line that intersects two or more other lines at distinct points.
What are corresponding angles?
+Corresponding angles are angles that are in the same relative position on the corresponding lines.
What are alternate interior angles?
+Alternate interior angles are angles that are inside the transversal line and on opposite sides of the transversal.
Related Terms:
- Alternate interior angles Worksheet PDF