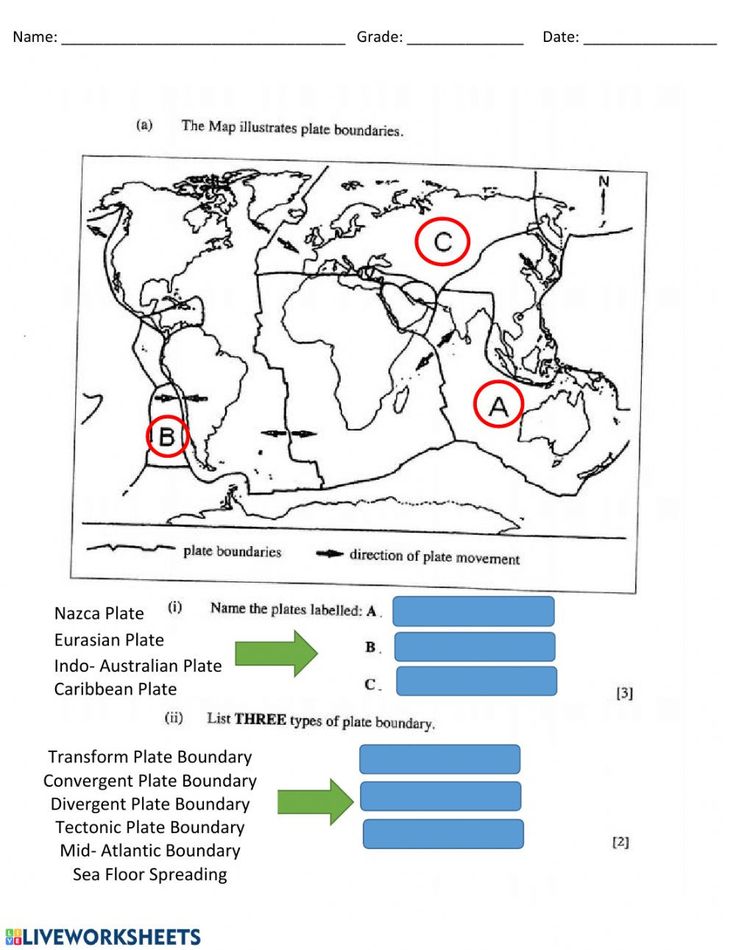
Tis The Season For The Slope Formula Worksheet
Mastering the Slope Formula: A Comprehensive Guide
As the winter season approaches, it’s time to hit the slopes – not just for skiing and snowboarding, but also for math enthusiasts who want to master the slope formula. In this article, we’ll delve into the world of linear equations, exploring the concept of slope, its significance, and how to calculate it using the slope formula.
What is Slope?
In mathematics, slope refers to the measure of how steep a line is. It’s a fundamental concept in algebra, geometry, and trigonometry, and is essential for graphing lines, understanding linear relationships, and solving equations. Slope is often represented by the letter ’m’ and is calculated as the ratio of the vertical change (rise) to the horizontal change (run) between two points on a line.
Why is Slope Important?
Slope plays a crucial role in various mathematical and real-world applications, including:
- Graphing lines: Slope helps determine the direction and steepness of a line, making it easier to graph and analyze.
- Linear relationships: Slope represents the rate of change between two variables, enabling us to model and understand real-world phenomena, such as population growth, financial trends, and scientific data.
- Optimization problems: Slope is used to find the maximum or minimum of a function, which is essential in fields like economics, physics, and engineering.
- Trigonometry: Slope is related to the tangent function, which is used to calculate angles and side lengths in right triangles.
The Slope Formula
The slope formula is a simple yet powerful tool for calculating the slope of a line. Given two points (x1, y1) and (x2, y2), the slope (m) can be calculated using the following formula:
m = (y2 - y1) / (x2 - x1)
This formula can be applied to various types of problems, including:
- Finding the slope of a line: Given two points on a line, the slope formula can be used to calculate the slope.
- Graphing lines: By using the slope formula, you can determine the direction and steepness of a line, making it easier to graph.
- Writing linear equations: The slope formula can be used to write linear equations in slope-intercept form (y = mx + b) or point-slope form (y - y1 = m(x - x1)).
📝 Note: The slope formula is only applicable to linear equations. For non-linear equations, other methods, such as the power rule or the product rule, may be used to calculate the slope.
How to Use the Slope Formula
Using the slope formula is straightforward. Here’s a step-by-step guide:
- Identify the two points on the line, (x1, y1) and (x2, y2).
- Plug the values into the slope formula: m = (y2 - y1) / (x2 - x1).
- Simplify the expression to calculate the slope.
Example:
Find the slope of the line passing through points (2, 3) and (4, 5).
m = (5 - 3) / (4 - 2) = 2 / 2 = 1
The slope of the line is 1.
Practice Time!
Now that you’ve mastered the slope formula, it’s time to practice. Here are some exercises to help you reinforce your understanding:

| Points | Slope |
|---|---|
| (1, 2) and (3, 4) | ? |
| (2, 3) and (4, 6) | ? |
| (0, 1) and (2, 3) | ? |
Answers:
| Points | Slope |
|---|---|
| (1, 2) and (3, 4) | 1 |
| (2, 3) and (4, 6) | 1.5 |
| (0, 1) and (2, 3) | 1 |
Conclusion
Mastering the slope formula is an essential skill for anyone who wants to excel in mathematics and science. By understanding the concept of slope and how to calculate it using the slope formula, you’ll be able to tackle a wide range of problems and applications. Remember to practice regularly and apply the slope formula to real-world scenarios to reinforce your understanding.
What is the slope formula?
+The slope formula is m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are two points on a line.
Why is slope important in mathematics?
+Slope is important in mathematics because it represents the rate of change between two variables, enabling us to model and understand real-world phenomena, such as population growth, financial trends, and scientific data.
Can the slope formula be used for non-linear equations?
+No, the slope formula is only applicable to linear equations. For non-linear equations, other methods, such as the power rule or the product rule, may be used to calculate the slope.