Solving Systems Word Problems Made Easy

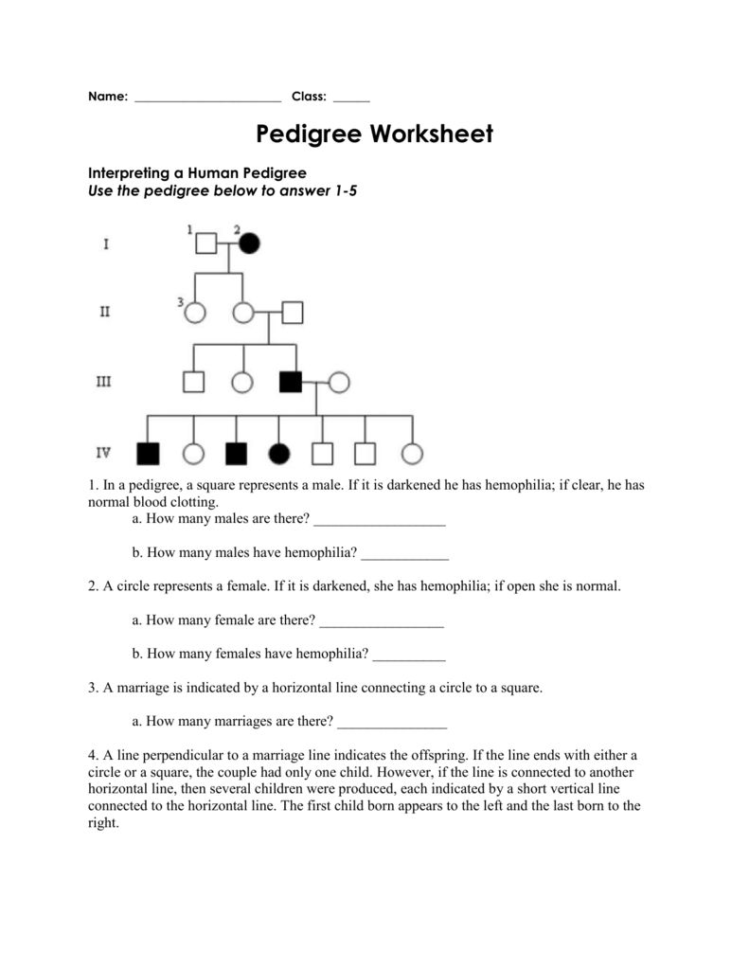
Understanding Systems of Equations

Systems of equations are a fundamental concept in algebra, and they can be used to solve a wide range of problems in fields such as physics, engineering, economics, and computer science. A system of equations is a set of two or more equations that contain two or more variables. In this blog post, we will explore how to solve systems of equations word problems with ease.

Real-World Applications of Systems of Equations

Systems of equations have numerous real-world applications. For instance, they can be used to:

• Determine the maximum profit and minimum cost in a business
• Calculate the distance and time it takes to travel between two cities
• Model population growth and decline
• Solve problems in physics, such as the motion of objects and the forces acting upon them
• Analyze and interpret data in economics, social sciences, and medicine

How to Read and Interpret Word Problems

When reading and interpreting word problems, it is essential to:

• Identify the variables and constants in the problem
• Determine the relationships between the variables
• Translate the problem into mathematical equations
• Use algebraic methods to solve the system of equations

Methods for Solving Systems of Equations

There are several methods for solving systems of equations, including:

• Substitution method: This involves solving one equation for one variable and substituting that expression into the other equation.
• Elimination method: This involves adding or subtracting the equations to eliminate one variable.
• Graphical method: This involves graphing the equations on a coordinate plane and finding the point of intersection.

Solving Systems of Equations Word Problems

Here are some examples of systems of equations word problems and how to solve them:

Example 1:

Maria and John are traveling to a concert. Maria’s car travels at an average speed of 60 miles per hour, while John’s car travels at an average speed of 40 miles per hour. If they both leave at the same time and Maria arrives at the concert 30 minutes before John, how far is the concert from their starting point?

Solution:

Let x be the distance from the starting point to the concert. Since Maria travels at an average speed of 60 miles per hour, the time it takes her to travel to the concert is x/60. Similarly, the time it takes John to travel to the concert is x/40.

Since Maria arrives 30 minutes before John, we can set up the following equation:

x/60 = x/40 - ¹⁄₂

Simplifying the equation, we get:

x = 120 miles

Therefore, the concert is 120 miles from their starting point.

Example 2:

A bakery sells two types of bread: whole wheat and white bread. The whole wheat bread costs 2 per loaf, and the white bread costs 1.50 per loaf. If the bakery sells 250 loaves of bread per day and makes a total of $425 per day, how many loaves of whole wheat bread and white bread does the bakery sell per day?

Solution:

Let x be the number of loaves of whole wheat bread sold per day, and let y be the number of loaves of white bread sold per day.

Since the bakery sells 250 loaves of bread per day, we can set up the following equation:

x + y = 250

Since the whole wheat bread costs 2 per loaf and the white bread costs 1.50 per loaf, we can set up the following equation:

2x + 1.5y = 425

Solving the system of equations using the substitution method, we get:

x = 100

y = 150

Therefore, the bakery sells 100 loaves of whole wheat bread and 150 loaves of white bread per day.

📝 Note: When solving systems of equations word problems, it is essential to read the problem carefully and identify the variables and constants. Use algebraic methods to solve the system of equations, and check your solutions to ensure they are reasonable and make sense in the context of the problem.

Common Mistakes to Avoid

When solving systems of equations word problems, there are several common mistakes to avoid:

• Failing to read the problem carefully and identify the variables and constants
• Using incorrect algebraic methods to solve the system of equations
• Failing to check solutions to ensure they are reasonable and make sense in the context of the problem

Conclusion

Solving systems of equations word problems can be challenging, but with practice and patience, it can become easier. By reading and interpreting word problems carefully, using algebraic methods to solve the system of equations, and checking solutions to ensure they are reasonable and make sense in the context of the problem, you can become proficient in solving systems of equations word problems.

Related Terms:

• System of equations word problems