Solve 3 Variable Systems of Equations Easily
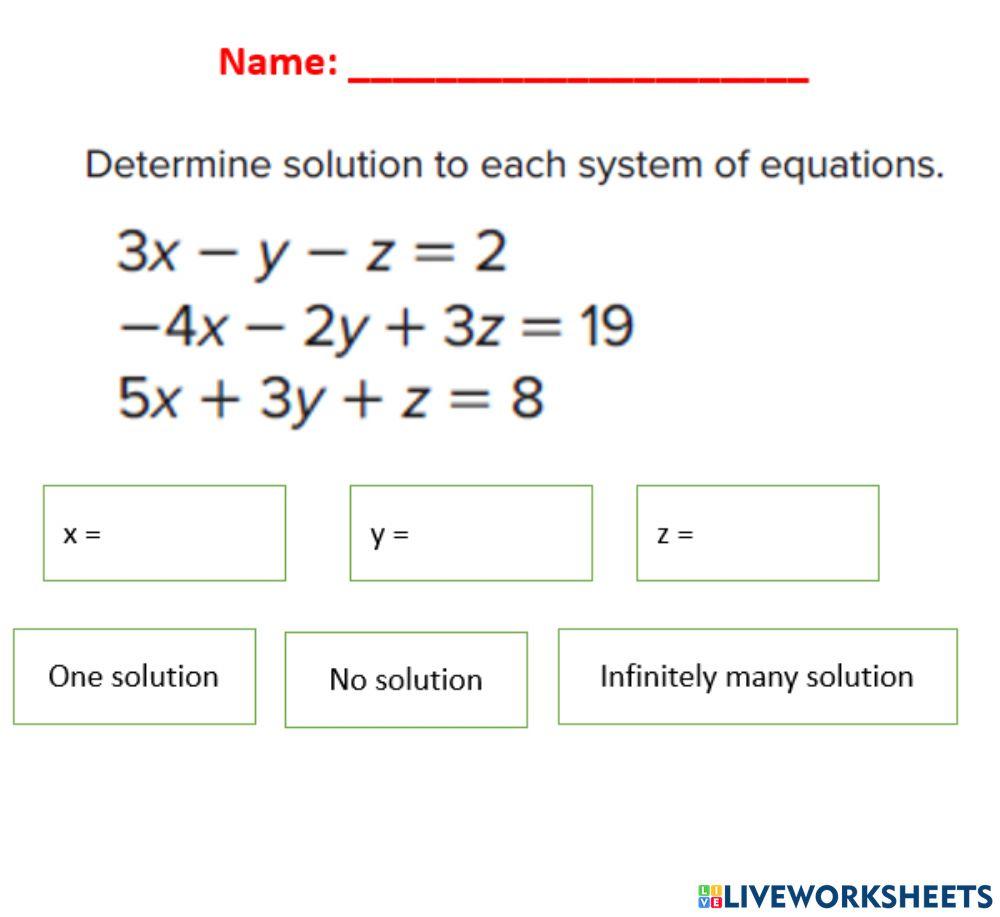
Understanding 3 Variable Systems of Equations
Solving systems of equations with three variables can seem daunting, but with the right approach, it can be easily managed. These systems involve three equations with three unknowns (usually x, y, and z). The goal is to find the values of x, y, and z that satisfy all three equations simultaneously.
Methods for Solving 3 Variable Systems of Equations
There are several methods for solving systems of equations with three variables, including:
- Substitution Method: This method involves solving one equation for one variable and then substituting that expression into the other two equations.
- Elimination Method: This method involves adding or subtracting the equations in such a way that one variable is eliminated, making it easier to solve for the remaining variables.
- Matrices Method: This method involves representing the system of equations as an augmented matrix and using row operations to transform the matrix into reduced row echelon form.
Step-by-Step Guide to Solving 3 Variable Systems of Equations
Here is a step-by-step guide to solving systems of equations with three variables using the elimination method:
Step 1: Write Down the Equations
Start by writing down the three equations. For example:
2x + 3y - z = 7 x - 2y + 3z = 11 4x + 2y + z = 15
Step 2: Eliminate One Variable
Choose one variable to eliminate. Let’s eliminate z. Multiply the first equation by 3 and the second equation by 1, and then add them together:
6x + 9y - 3z = 21
x - 2y + 3z = 11
7x + 7y = 32
Step 3: Eliminate the Same Variable from Another Pair of Equations
Choose another pair of equations and eliminate the same variable. Let’s eliminate z from the second and third equations. Multiply the second equation by 1 and the third equation by -3, and then add them together:
x - 2y + 3z = 11
-12x - 6y - 3z = -45
-11x - 8y = -34
Step 4: Solve for One Variable
Now we have two equations with two variables. We can solve for one variable. Let’s solve for x. Multiply the first equation by 11 and the second equation by 7, and then add them together:
77x + 77y = 352
-77x - 56y = -238
21y = 114
y = 114 / 21 y = 5.43
Step 5: Substitute the Value Back into One of the Original Equations
Now that we have the value of y, we can substitute it back into one of the original equations to solve for x. Let’s use the first equation:
2x + 3(5.43) - z = 7 2x + 16.29 - z = 7
Step 6: Solve for the Remaining Variables
Now we can solve for x and z. Let’s solve for x:
2x = 7 - 16.29 + z 2x = -9.29 + z x = (-9.29 + z) / 2
Now substitute the value of x back into one of the original equations to solve for z. Let’s use the second equation:
(-9.29 + z) / 2 - 2(5.43) + 3z = 11 -9.29 + z - 10.86 + 6z = 22 7z = 42.15 z = 6.02
🤔 Note: The values of x, y, and z are approximate, as the calculations involve decimals.
Step 7: Check Your Solution
Finally, plug the values of x, y, and z back into all three original equations to ensure that they satisfy all three equations.
Using Matrices to Solve 3 Variable Systems of Equations
Another method for solving systems of equations with three variables is to use matrices. This method involves representing the system of equations as an augmented matrix and using row operations to transform the matrix into reduced row echelon form.

| x | y | z | b |
|---|---|---|---|
| 2 | 3 | -1 | 7 |
| 1 | -2 | 3 | 11 |
| 4 | 2 | 1 | 15 |
To solve the system, we can use row operations to transform the matrix into reduced row echelon form.
What are the different methods for solving systems of equations with three variables?
+The three main methods for solving systems of equations with three variables are the substitution method, elimination method, and matrices method.
How do I know which method to use for solving a system of equations with three variables?
+The choice of method depends on the specific system of equations and the values of the coefficients. The elimination method is often the most straightforward, while the matrices method can be more efficient for larger systems.
Can I use a calculator to solve systems of equations with three variables?
+Yes, many calculators have built-in functions for solving systems of equations. However, it's still important to understand the underlying methods and principles.
In conclusion, solving systems of equations with three variables requires patience, attention to detail, and a solid understanding of algebraic methods. By following the steps outlined above, you can master the techniques and become proficient in solving these types of systems.
Related Terms:
- Systems of equations worksheet
- Systems of 3 equations