Surface Area of Triangular Prisms Worksheet and Solutions
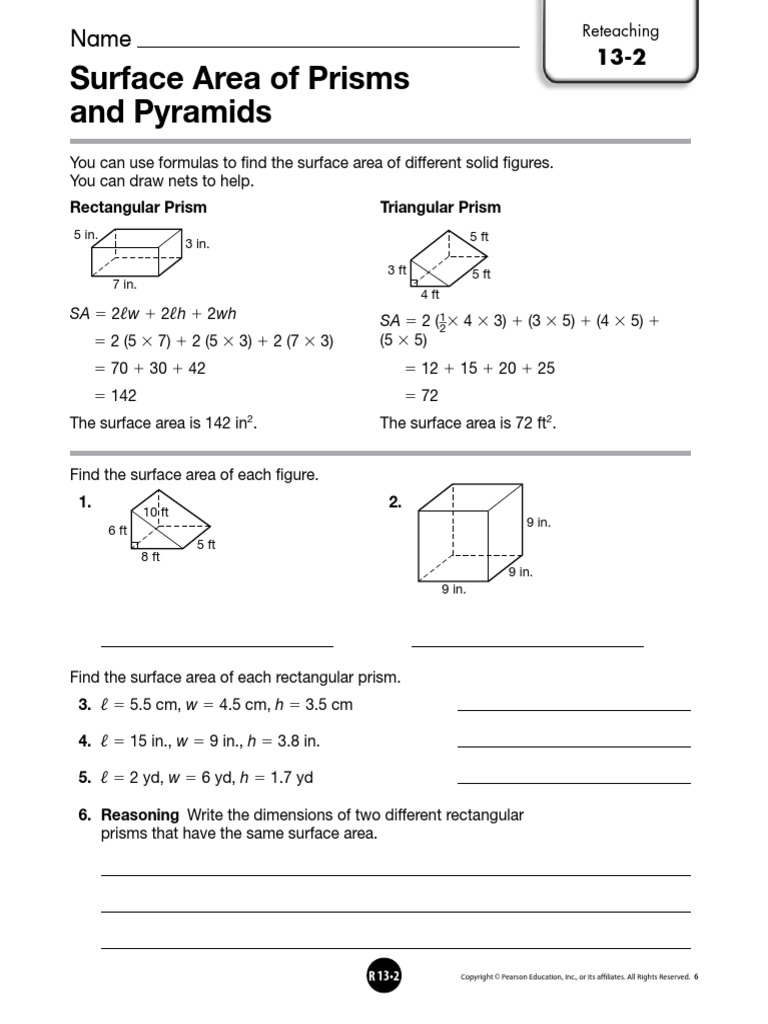
Understanding the Surface Area of Triangular Prisms
A triangular prism is a three-dimensional shape with two identical faces that are triangles, and three rectangular faces that connect the triangular faces. The surface area of a triangular prism is the total area of all its faces. In this article, we will delve into the concept of surface area of triangular prisms, explore the formula, and provide a worksheet with solutions.
The Formula for the Surface Area of a Triangular Prism
The surface area of a triangular prism can be calculated using the following formula:
Surface Area = 2 × (Area of the triangular base) + (Perimeter of the base × Height)
Where:
- Area of the triangular base is the area of one of the triangular faces, which can be calculated using the formula for the area of a triangle: (Base × Height) / 2
- Perimeter of the base is the perimeter of the triangular base, which is the sum of the lengths of all its sides
- Height is the height of the prism, which is the distance between the two triangular bases
Worksheet: Calculating the Surface Area of Triangular Prisms
Here are five problems to help you practice calculating the surface area of triangular prisms:
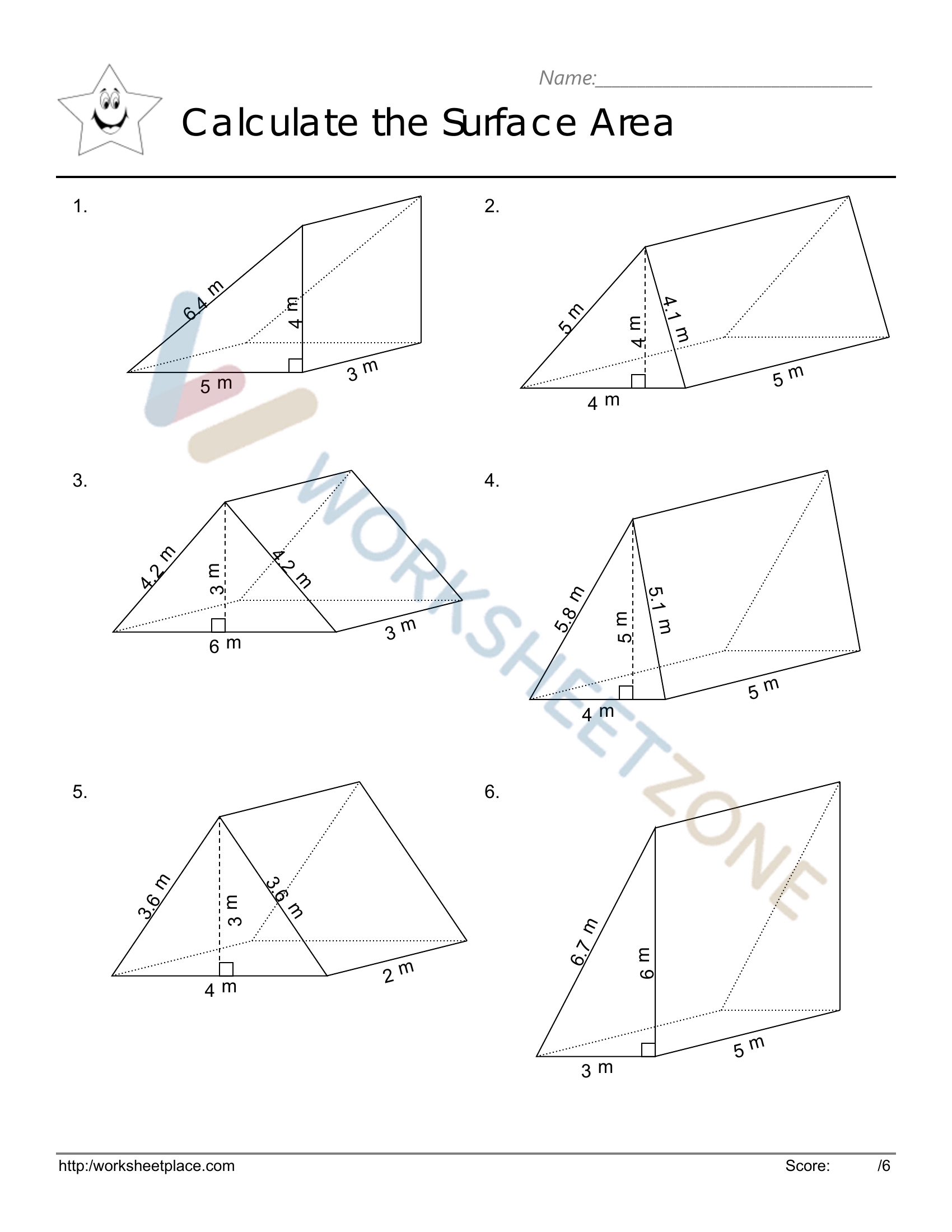
| Problem # | Triangular Base Dimensions | Height | Perimeter of Base |
|---|---|---|---|
| 1 | Base: 5cm, Height: 6cm | 8cm | 16cm |
| 2 | Base: 3cm, Height: 4cm | 6cm | 12cm |
| 3 | Base: 7cm, Height: 8cm | 10cm | 22cm |
| 4 | Base: 9cm, Height: 12cm | 8cm | 24cm |
| 5 | Base: 6cm, Height: 8cm | 9cm | 20cm |
Calculate the surface area of each triangular prism and check your answers with the solutions provided below.
🤔 Note: Make sure to use the correct units (e.g., square centimeters) when calculating the surface area.
Solutions to the Worksheet
| Problem # | Solution |
|---|---|
| 1 | Surface Area = 2 × (5 × 6 / 2) + (16 × 8) = 60 + 128 = 188 cm² |
| 2 | Surface Area = 2 × (3 × 4 / 2) + (12 × 6) = 24 + 72 = 96 cm² |
| 3 | Surface Area = 2 × (7 × 8 / 2) + (22 × 10) = 112 + 220 = 332 cm² |
| 4 | Surface Area = 2 × (9 × 12 / 2) + (24 × 8) = 216 + 192 = 408 cm² |
| 5 | Surface Area = 2 × (6 × 8 / 2) + (20 × 9) = 96 + 180 = 276 cm² |
Real-World Applications of Surface Area of Triangular Prisms
Understanding the surface area of triangular prisms is crucial in various real-world applications, such as:
- Architecture: Triangular prisms are used in building design, and calculating their surface area helps architects determine the amount of materials needed for construction.
- Engineering: Triangular prisms are used in bridge construction, and calculating their surface area helps engineers determine the structural integrity of the bridge.
- Product Design: Triangular prisms are used in product design, and calculating their surface area helps designers determine the cost of production.
As we have seen, calculating the surface area of triangular prisms is a straightforward process that involves using the formula and plugging in the values of the base dimensions and height. By practicing with the worksheet provided, you can improve your skills in calculating the surface area of triangular prisms.
What is the formula for the surface area of a triangular prism?
+The formula for the surface area of a triangular prism is: Surface Area = 2 × (Area of the triangular base) + (Perimeter of the base × Height)
How do I calculate the area of the triangular base?
+The area of the triangular base can be calculated using the formula: (Base × Height) / 2
What are some real-world applications of calculating the surface area of triangular prisms?
+Calculating the surface area of triangular prisms is crucial in various real-world applications, such as architecture, engineering, and product design.



