Special Right Triangles Worksheet Answers
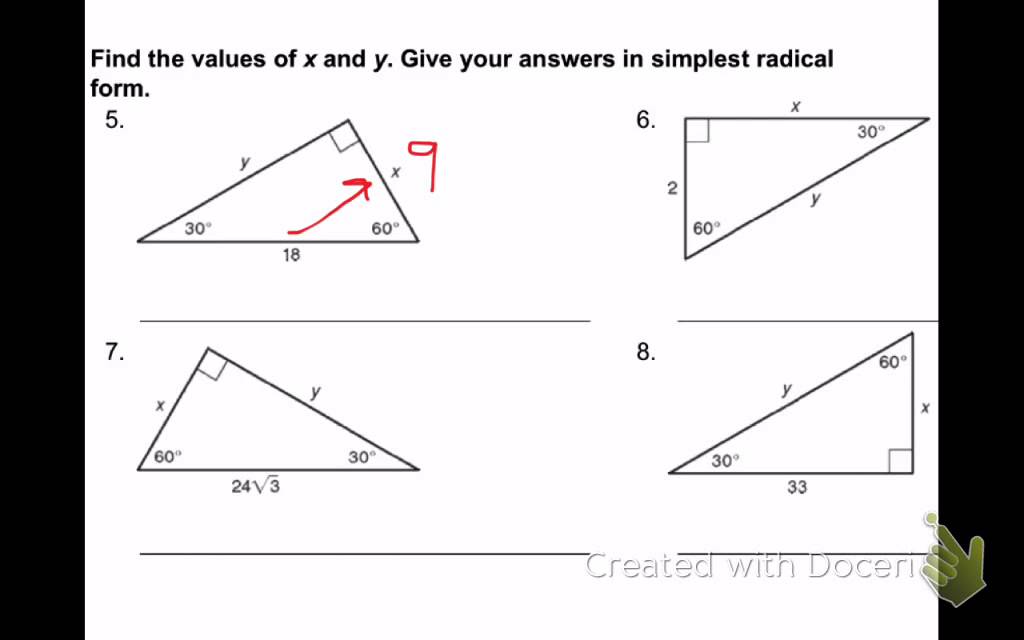
As a student, you’re likely to encounter special right triangles in your geometry or trigonometry class. These triangles have unique properties that make them useful for solving problems. In this post, we’ll review the key concepts and provide a worksheet with answers to help you practice.
Understanding Special Right Triangles
Special right triangles are right triangles with specific angle measurements that make them useful for solving problems. There are two main types of special right triangles: 30-60-90 triangles and 45-45-90 triangles.
30-60-90 Triangles
A 30-60-90 triangle has angle measurements of 30°, 60°, and 90°. The side opposite the 30° angle is half the length of the hypotenuse, while the side opposite the 60° angle is √3 times the length of the shorter side.
45-45-90 Triangles
A 45-45-90 triangle has two 45° angles and one 90° angle. The two legs are equal in length, and the hypotenuse is √2 times the length of each leg.
Special Right Triangles Worksheet
Here’s a worksheet with 10 problems to help you practice working with special right triangles. Use the concepts above to solve each problem.
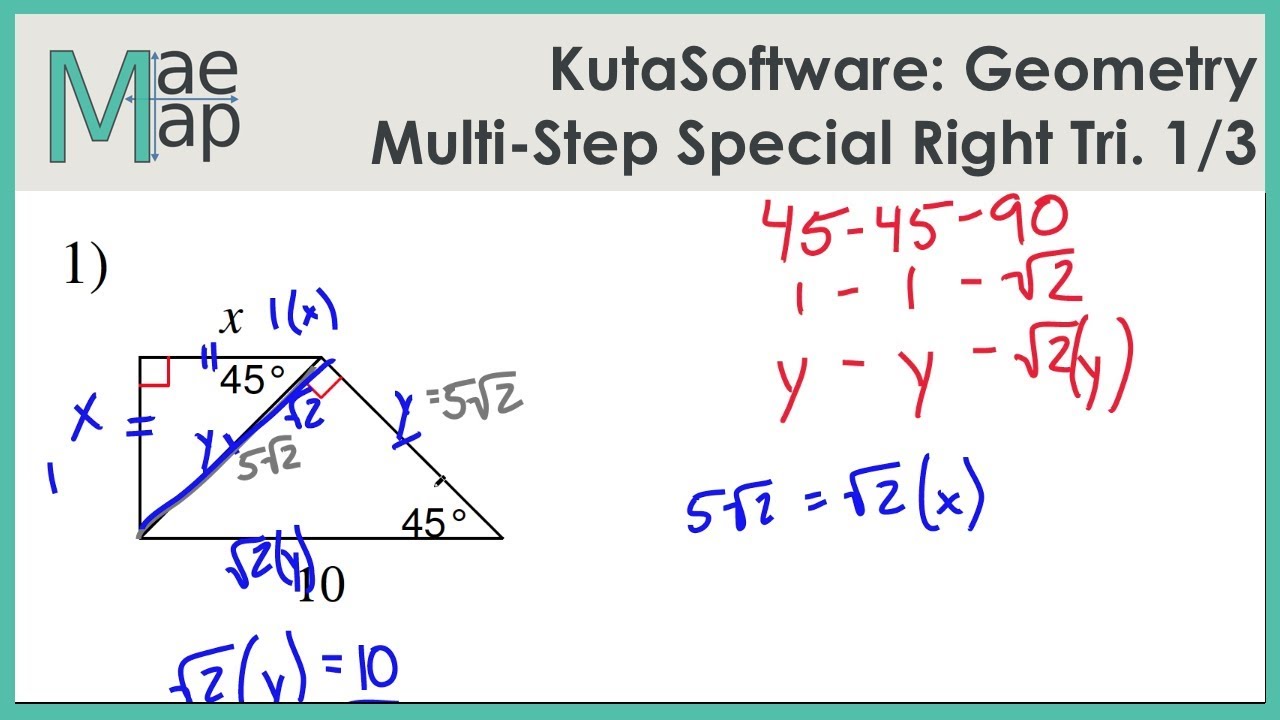
| Problem | Answer |
|---|---|
| In a 30-60-90 triangle, the length of the shorter side is 6 inches. Find the length of the hypotenuse. | 12 inches |
| In a 45-45-90 triangle, the length of each leg is 4 inches. Find the length of the hypotenuse. | 4√2 inches |
| In a 30-60-90 triangle, the length of the hypotenuse is 10 inches. Find the length of the shorter side. | 5 inches |
| In a 45-45-90 triangle, the length of the hypotenuse is 8 inches. Find the length of each leg. | 4√2 inches |
| In a 30-60-90 triangle, the length of the side opposite the 60° angle is 3√3 inches. Find the length of the shorter side. | 3 inches |
| In a 45-45-90 triangle, the length of each leg is x inches. If the length of the hypotenuse is 2x inches, find the value of x. | x = 2√2 inches |
| In a 30-60-90 triangle, the length of the shorter side is 2x inches. If the length of the hypotenuse is 4x inches, find the value of x. | x = 2 inches |
| In a 45-45-90 triangle, the length of the hypotenuse is 4x inches. If the length of each leg is x√2 inches, find the value of x. | x = 4 inches |
| In a 30-60-90 triangle, the length of the side opposite the 60° angle is 2√3 inches. Find the length of the shorter side. | 2 inches |
| In a 45-45-90 triangle, the length of each leg is 2x inches. If the length of the hypotenuse is x√2 inches, find the value of x. | x = 2 inches |
Final Thoughts
Special right triangles are an essential part of geometry and trigonometry. By understanding the unique properties of these triangles, you can solve problems more efficiently and effectively. Remember to practice regularly and apply these concepts to real-world problems.
What is the difference between a 30-60-90 triangle and a 45-45-90 triangle?
+A 30-60-90 triangle has angle measurements of 30°, 60°, and 90°, while a 45-45-90 triangle has two 45° angles and one 90° angle. The side lengths of these triangles also differ.
How do I identify a special right triangle?
+You can identify a special right triangle by looking at the angle measurements. If the triangle has a 30°, 60°, or 45° angle, it may be a special right triangle.
Why are special right triangles important in geometry and trigonometry?
+Special right triangles are important because they have unique properties that make them useful for solving problems. They can be used to find missing side lengths, angle measurements, and other properties of triangles.
Related Terms:
- Special Right Triangles Worksheet pdf
- Special Triangles Worksheet with answers
- Special right triangles Notes