5 Ways to Solve Linear Inequalities Easily
Understanding Linear Inequalities
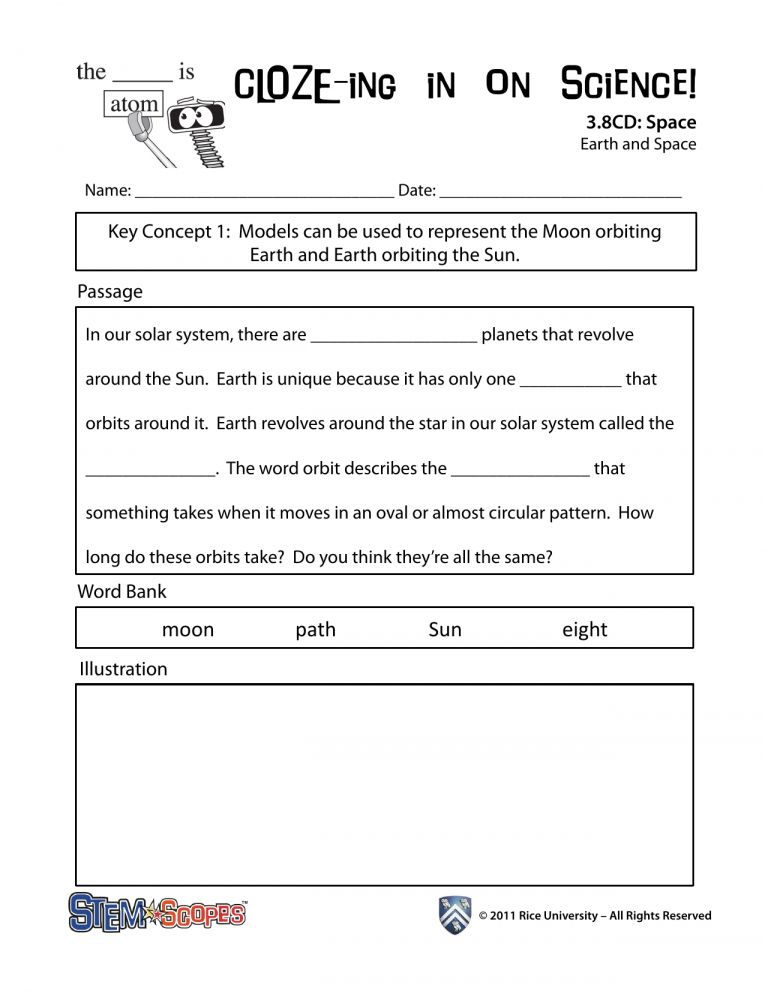
Linear inequalities are statements that compare two algebraic expressions using inequality symbols such as <, >, ≤, or ≥. They are used to represent relationships between variables and constants in mathematics, physics, engineering, and other fields. Solving linear inequalities is a crucial skill in algebra, and it can be challenging for some students. However, with the right strategies and techniques, solving linear inequalities can become easier.
1. Add or Subtract the Same Value to Both Sides
One of the simplest ways to solve linear inequalities is to add or subtract the same value to both sides of the inequality. This method is based on the property of inequality that states if a > b, then a + c > b + c, and if a < b, then a + c < b + c.
For example, consider the inequality 2x + 5 > 11. To solve this inequality, we can subtract 5 from both sides:
2x + 5 - 5 > 11 - 5
This simplifies to:
2x > 6
Next, we can divide both sides by 2:
x > 3
Therefore, the solution to the inequality 2x + 5 > 11 is x > 3.
2. Multiply or Divide Both Sides by a Positive Number
Another way to solve linear inequalities is to multiply or divide both sides by a positive number. This method is based on the property of inequality that states if a > b, then ac > bc, and if a < b, then ac < bc.
For example, consider the inequality 4x < 20. To solve this inequality, we can divide both sides by 4:
4x/4 < 20⁄4
This simplifies to:
x < 5
Therefore, the solution to the inequality 4x < 20 is x < 5.
3. Use the Inverse Operation to Isolate the Variable
Using the inverse operation to isolate the variable is another effective way to solve linear inequalities. The inverse operation is the opposite operation of the given operation. For example, the inverse operation of addition is subtraction, and the inverse operation of multiplication is division.
For example, consider the inequality 3x - 2 > 7. To solve this inequality, we can add 2 to both sides:
3x - 2 + 2 > 7 + 2
This simplifies to:
3x > 9
Next, we can divide both sides by 3:
x > 3
Therefore, the solution to the inequality 3x - 2 > 7 is x > 3.
4. Solve Inequalities with Fractions
Solving inequalities with fractions can be challenging, but it can be done using the same techniques as solving linear inequalities. To solve inequalities with fractions, we can start by multiplying both sides by the least common multiple (LCM) of the denominators.
For example, consider the inequality 1/2x + 1⁄4 > 3⁄4. To solve this inequality, we can multiply both sides by 4:
2x + 1 > 3
This simplifies to:
2x > 2
Next, we can divide both sides by 2:
x > 1
Therefore, the solution to the inequality 1/2x + 1⁄4 > 3⁄4 is x > 1.
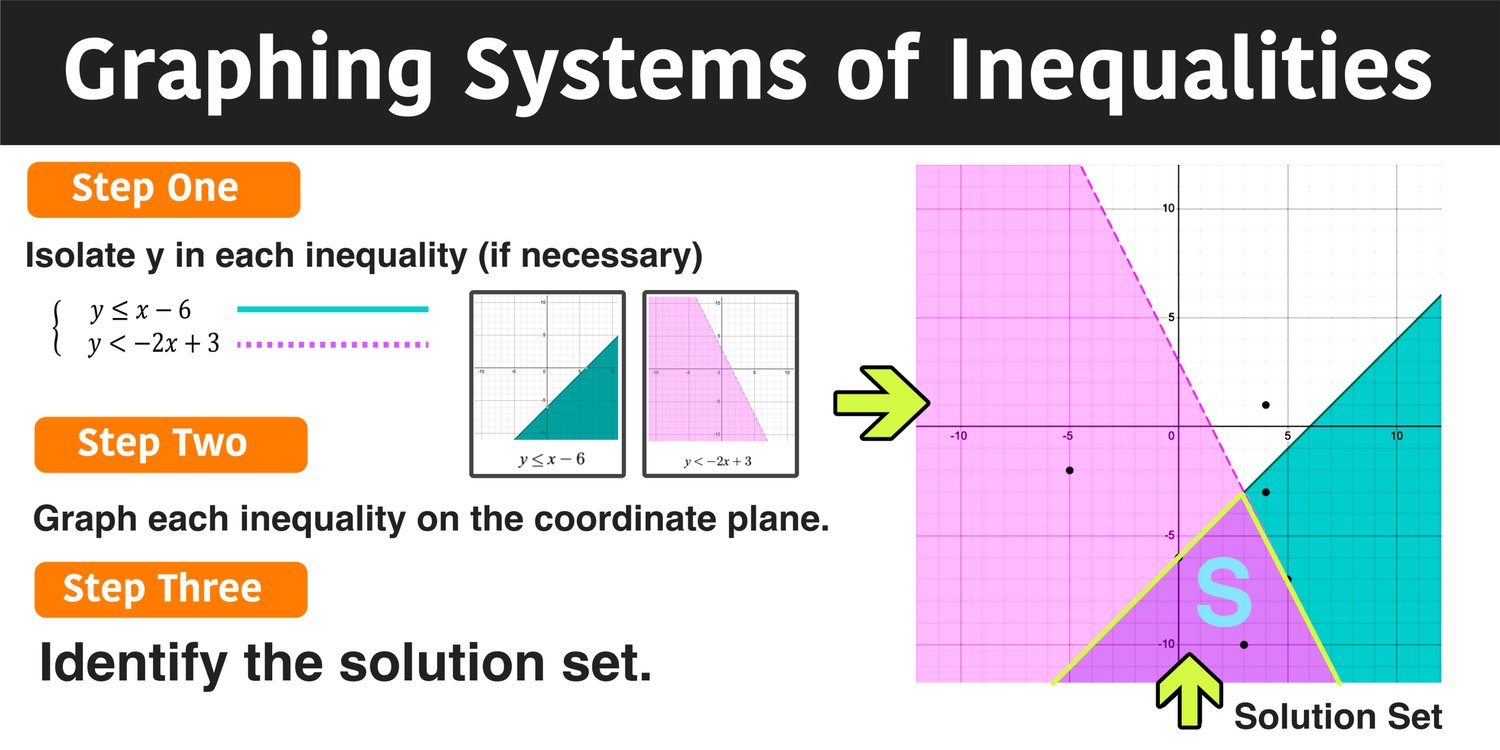
5. Use a Number Line to Visualize the Solution
Using a number line to visualize the solution is a great way to understand the concept of linear inequalities. A number line is a visual representation of the real numbers on a line. It can be used to show the relationship between the variable and the constant in an inequality.
For example, consider the inequality x > 2. We can represent this inequality on a number line as follows:
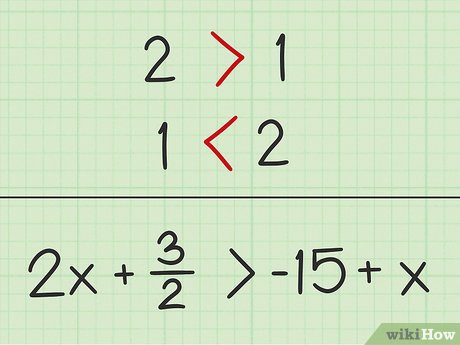
| ---> | 2 |
The arrow points to the right, indicating that the solution set includes all numbers greater than 2.
📝 Note: When solving linear inequalities, it's essential to consider the direction of the inequality symbol and the sign of the coefficient of the variable.
📝 Note: Inequalities can have infinitely many solutions, so it's essential to represent the solution set using interval notation or a number line.
What is a linear inequality?
+A linear inequality is a statement that compares two algebraic expressions using inequality symbols such as <, >, ≤, or ≥.
How do I solve linear inequalities?
+There are several ways to solve linear inequalities, including adding or subtracting the same value to both sides, multiplying or dividing both sides by a positive number, using the inverse operation to isolate the variable, solving inequalities with fractions, and using a number line to visualize the solution.
What is the difference between a linear inequality and a linear equation?
+A linear inequality is a statement that compares two algebraic expressions using inequality symbols, while a linear equation is a statement that sets two algebraic expressions equal to each other.
In conclusion, solving linear inequalities requires a deep understanding of algebraic concepts and techniques. By using the strategies outlined in this article, you can become proficient in solving linear inequalities and improve your overall math skills. Remember to always consider the direction of the inequality symbol and the sign of the coefficient of the variable when solving linear inequalities.
Related Terms:
- Linear Inequalities pdf
- Systems of linear inequalities worksheet
- Solving Linear Inequalities Worksheet PDF
- Solving linear inequalities answer key
- Linear Inequalities Grade 9 worksheets