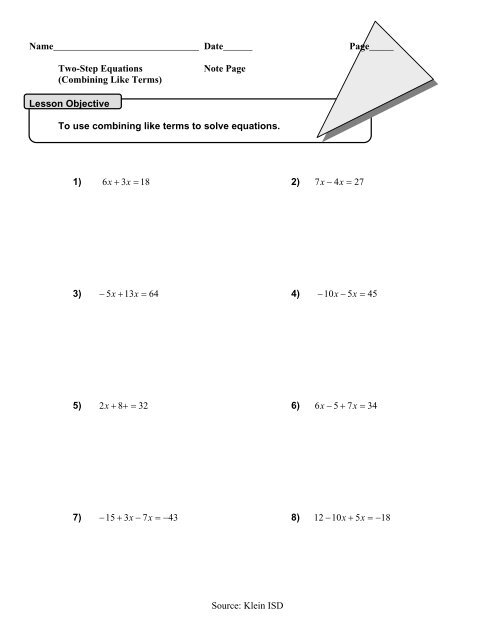
7 Ways to Solve Equations Using Like Terms
Understanding Like Terms in Equations
When dealing with algebraic equations, one of the fundamental concepts to grasp is the idea of like terms. Like terms are terms that have the same variable(s) raised to the same power. In other words, terms that have the same variable part can be combined or simplified by adding or subtracting their coefficients. This concept is crucial for simplifying and solving equations.
Why Are Like Terms Important in Solving Equations?
Like terms are essential in solving equations because they allow us to simplify complex expressions by combining similar terms. This simplification process can help us isolate the variable and solve for its value. By combining like terms, we can also eliminate unnecessary complexity in equations, making it easier to solve them.
7 Ways to Solve Equations Using Like Terms
1. Identify Like Terms
Before we can solve equations using like terms, we need to identify them. This involves looking at each term in the equation and determining which terms have the same variable(s) raised to the same power.
Example: 2x + 3x - 4y
Like terms: 2x and 3x (both have the variable x)
2. Combine Like Terms
Once we’ve identified the like terms, we can combine them by adding or subtracting their coefficients.
Example: 2x + 3x - 4y = (2+3)x - 4y = 5x - 4y
3. Use the Commutative Property
The commutative property states that we can rearrange the order of the terms in an equation without changing its value. This property can be useful when combining like terms.
Example: x + 2y + 3x = 3x + x + 2y = (3+1)x + 2y = 4x + 2y
4. Use the Associative Property
The associative property states that we can regroup the terms in an equation without changing its value. This property can be useful when combining like terms.
Example: (2x + 3y) + (4x + 5y) = 2x + (3y + 4x) + 5y = 2x + 4x + 3y + 5y = (2+4)x + (3+5)y = 6x + 8y
5. Use the Distributive Property
The distributive property states that we can distribute a single term to multiple terms inside parentheses. This property can be useful when combining like terms.
Example: 2(x + 3y) = 2x + 6y
6. Eliminate Unnecessary Complexity
Combining like terms can help eliminate unnecessary complexity in equations.
Example: 2x + 3x - 2x + 5y = (2+3-2)x + 5y = 3x + 5y
7. Check Your Work
Finally, it’s essential to check your work when solving equations using like terms.
Example: Check if 2x + 3x = 5x (yes)
📝 Note: Always follow the order of operations (PEMDAS) when solving equations with like terms.
Conclusion
In this article, we’ve discussed the importance of like terms in solving equations and provided 7 ways to solve equations using like terms. By identifying like terms, combining them, and using properties such as the commutative, associative, and distributive properties, we can simplify complex equations and solve for the value of the variable. Remember to always check your work and follow the order of operations when solving equations with like terms.
What are like terms in algebra?
+Like terms are terms that have the same variable(s) raised to the same power.
Why are like terms important in solving equations?
+Like terms are essential in solving equations because they allow us to simplify complex expressions by combining similar terms.
How do we combine like terms?
+We combine like terms by adding or subtracting their coefficients.
Related Terms:
- Solving Equations combining like terms
- Solving multi step Equations Worksheet