Solving Absolute Value Equations Worksheet
Solving Absolute Value Equations: A Comprehensive Guide
Absolute value equations are a fundamental concept in mathematics, and solving them can be a challenging task for many students. In this article, we will provide a comprehensive guide on how to solve absolute value equations, including the rules, examples, and practice exercises.
What is an Absolute Value Equation?
An absolute value equation is an equation that contains an absolute value expression, which is a value that is always non-negative. The absolute value of a number is its distance from zero on the number line. For example, the absolute value of 5 is 5, and the absolute value of -5 is also 5.
Types of Absolute Value Equations
There are two types of absolute value equations:
- Simple Absolute Value Equations: These are equations where the absolute value expression is isolated on one side of the equation. For example: |x| = 5
- Compound Absolute Value Equations: These are equations where the absolute value expression is part of a larger equation. For example: |x + 3| = 5
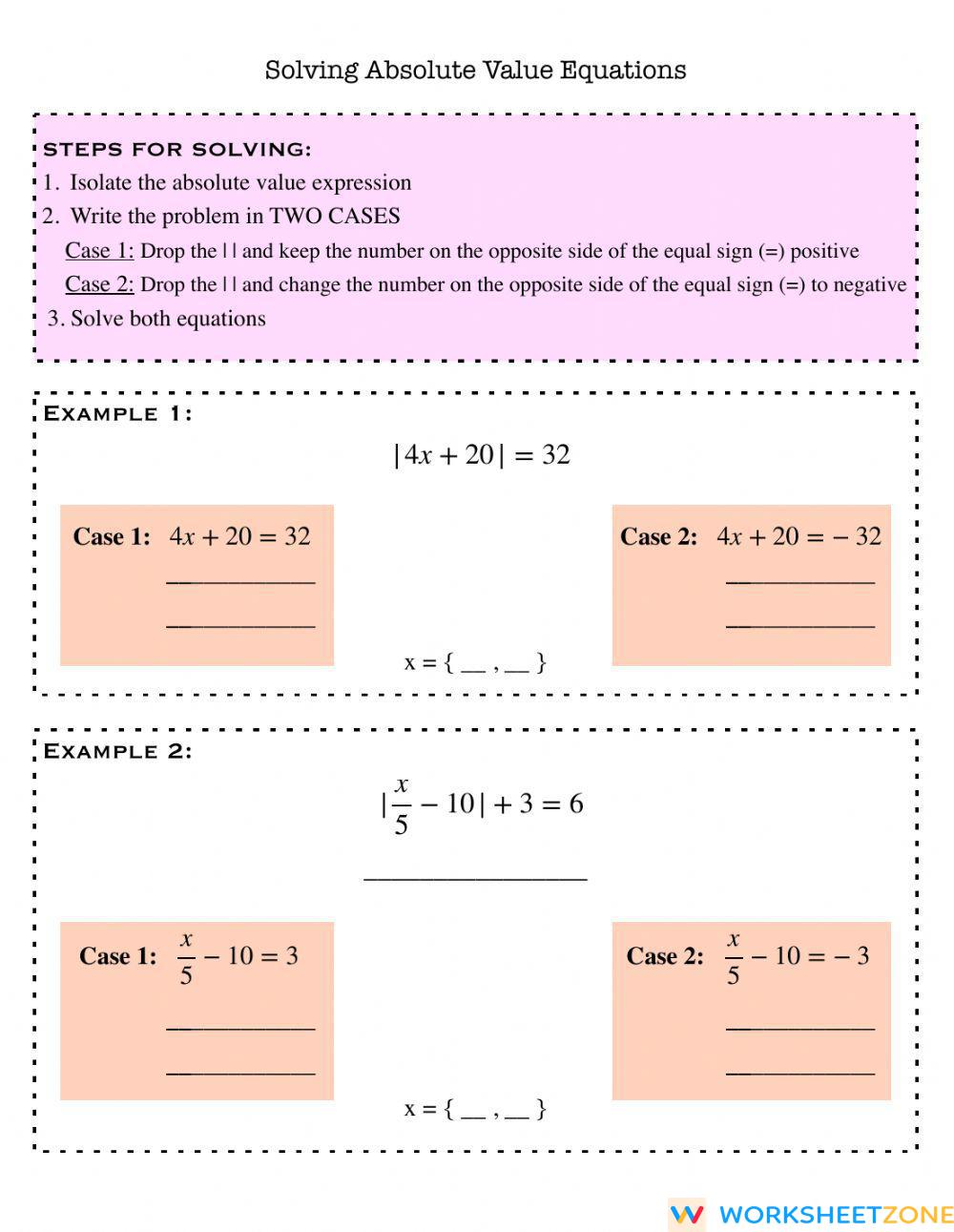
Rules for Solving Absolute Value Equations
To solve absolute value equations, we need to follow these rules:
- Rule 1: If the absolute value expression is isolated on one side of the equation, we can solve it by splitting it into two separate equations. For example: |x| = 5 can be split into x = 5 and x = -5.
- Rule 2: If the absolute value expression is part of a larger equation, we need to isolate it first. For example: |x + 3| = 5 can be isolated by subtracting 3 from both sides: |x| = 2.
- Rule 3: If the absolute value expression is equal to a negative number, there is no solution. For example: |x| = -5 has no solution.
Examples of Solving Absolute Value Equations
Here are some examples of solving absolute value equations:
- Example 1: |x| = 5 Solution: x = 5 and x = -5
- Example 2: |x + 3| = 5 Solution: x + 3 = 5 or x + 3 = -5 x = 2 or x = -8
- Example 3: |x - 2| = 3 Solution: x - 2 = 3 or x - 2 = -3 x = 5 or x = -1
📝 Note: When solving absolute value equations, it's essential to check your solutions to ensure they satisfy the original equation.
Practice Exercises
Here are some practice exercises to help you master solving absolute value equations:

| Equation | Solution |
|---|---|
| x | |
| x + 2 | |
| x - 1 | |
| x | |
| x + 1 |
Conclusion
Solving absolute value equations requires attention to detail and a clear understanding of the rules. By following the rules outlined in this article and practicing with the provided exercises, you can become proficient in solving absolute value equations.
What is the purpose of absolute value equations?
+Absolute value equations are used to model real-world situations where the magnitude of a quantity is important, but the direction is not.
How do I know which rule to apply when solving an absolute value equation?
+First, check if the absolute value expression is isolated on one side of the equation. If it is, apply Rule 1. If it’s part of a larger equation, apply Rule 2.
What if I get a negative solution when solving an absolute value equation?
+If you get a negative solution, it means the original equation has no solution.