6 Ways to Solve 2-Step Inequalities Easily
Understanding the Basics of 2-Step Inequalities
Solving 2-step inequalities is an essential skill in algebra, and it’s used to solve equations that involve two operations. A 2-step inequality is an inequality that requires two steps to solve. The goal is to isolate the variable, usually represented by x, on one side of the inequality sign.
What are 2-step inequalities?
Two-step inequalities are inequalities that require two operations to solve. They are used to represent relationships between variables and constants. For example, 2x + 3 > 5 is a 2-step inequality because it requires two operations: subtracting 3 and then dividing by 2.
Method 1: Solving 2-Step Inequalities by Adding or Subtracting
To solve 2-step inequalities, start by isolating the term with the variable. This is done by adding or subtracting the same value to both sides of the inequality.
Example 1:
Solve the inequality: 2x + 5 > 11
Step 1: Subtract 5 from both sides 2x + 5 - 5 > 11 - 5 2x > 6
Step 2: Divide both sides by 2 2x / 2 > 6 / 2 x > 3
📝 Note: When dividing or multiplying both sides of an inequality by a negative number, flip the direction of the inequality sign.
Method 2: Solving 2-Step Inequalities by Multiplying or Dividing
If the inequality involves multiplication or division, start by isolating the term with the variable. This is done by multiplying or dividing both sides of the inequality by the same value.
Example 2:
Solve the inequality: 3x / 2 < 9
Step 1: Multiply both sides by 2 (3x / 2) * 2 < 9 * 2 3x < 18
Step 2: Divide both sides by 3 3x / 3 < 18 / 3 x < 6
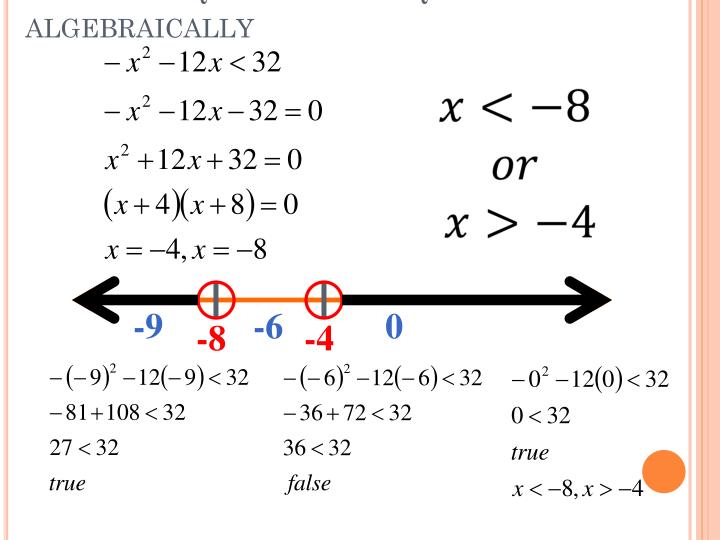
Method 3: Solving 2-Step Inequalities with Negative Numbers
When solving 2-step inequalities involving negative numbers, be careful when dividing or multiplying both sides by a negative number. Flip the direction of the inequality sign.
Example 3:
Solve the inequality: -2x + 3 > 7
Step 1: Subtract 3 from both sides -2x + 3 - 3 > 7 - 3 -2x > 4
Step 2: Divide both sides by -2 (flip the inequality sign) -2x / -2 < 4 / -2 x < -2
Method 4: Solving 2-Step Inequalities with Fractions
When solving 2-step inequalities involving fractions, start by finding the least common multiple (LCM) of the denominators.
Example 4:
Solve the inequality: 1/2x + 3⁄4 > 2
Step 1: Multiply both sides by the LCM (4) (1/2x + 3⁄4) * 4 > 2 * 4 2x + 3 > 8
Step 2: Subtract 3 from both sides 2x + 3 - 3 > 8 - 3 2x > 5
Step 3: Divide both sides by 2 2x / 2 > 5 / 2 x > 2.5
Method 5: Solving 2-Step Inequalities with Decimals
When solving 2-step inequalities involving decimals, start by converting the decimals to fractions.
Example 5:
Solve the inequality: 0.5x + 2 > 3
Step 1: Convert the decimal to a fraction (1⁄2)x + 2 > 3
Step 2: Subtract 2 from both sides (1⁄2)x + 2 - 2 > 3 - 2 (1⁄2)x > 1
Step 3: Multiply both sides by 2 (1⁄2)x * 2 > 1 * 2 x > 2
Method 6: Using Inverse Operations to Solve 2-Step Inequalities
To solve 2-step inequalities, use inverse operations to isolate the variable.
Example 6:
Solve the inequality: x / 3 + 2 > 5
Step 1: Subtract 2 from both sides x / 3 + 2 - 2 > 5 - 2 x / 3 > 3
Step 2: Multiply both sides by 3 (x / 3) * 3 > 3 * 3 x > 9
Now that you’ve learned the 6 ways to solve 2-step inequalities easily, practice makes perfect! Try solving more inequalities to become proficient in solving them.
What is a 2-step inequality?
+A 2-step inequality is an inequality that requires two operations to solve, such as adding or subtracting, and then multiplying or dividing.
How do I solve a 2-step inequality with negative numbers?
+When solving 2-step inequalities involving negative numbers, be careful when dividing or multiplying both sides by a negative number. Flip the direction of the inequality sign.
Can I use a calculator to solve 2-step inequalities?
+While calculators can be helpful in solving inequalities, it’s essential to understand the steps and concepts behind solving 2-step inequalities. Practice solving them manually to become proficient.