Slope Formula Worksheet
Understanding the Slope Formula: A Comprehensive Guide
The slope formula is a fundamental concept in mathematics, particularly in algebra and geometry. It is used to calculate the steepness of a line, which is essential in various real-world applications, such as physics, engineering, and economics. In this article, we will delve into the world of slope formulas, exploring what they are, how to use them, and providing a slope formula worksheet to help you practice.
What is the Slope Formula?
The slope formula, also known as the gradient formula, is a mathematical formula used to calculate the slope of a line. The slope of a line is a measure of how steep it is, and it is calculated by dividing the vertical change (rise) by the horizontal change (run). The slope formula is:
Slope (m) = Rise (y2 - y1) / Run (x2 - x1)
where:
- m is the slope of the line
- (x1, y1) and (x2, y2) are two points on the line
How to Use the Slope Formula
Using the slope formula is straightforward. Follow these steps:
- Identify two points on the line, (x1, y1) and (x2, y2).
- Calculate the rise (y2 - y1).
- Calculate the run (x2 - x1).
- Divide the rise by the run to get the slope (m).
For example, let’s say we have two points on a line: (2, 3) and (4, 5). To calculate the slope, we would:
- Identify the points: (x1, y1) = (2, 3) and (x2, y2) = (4, 5).
- Calculate the rise: y2 - y1 = 5 - 3 = 2.
- Calculate the run: x2 - x1 = 4 - 2 = 2.
- Calculate the slope: m = rise / run = 2 / 2 = 1.
Therefore, the slope of the line is 1.
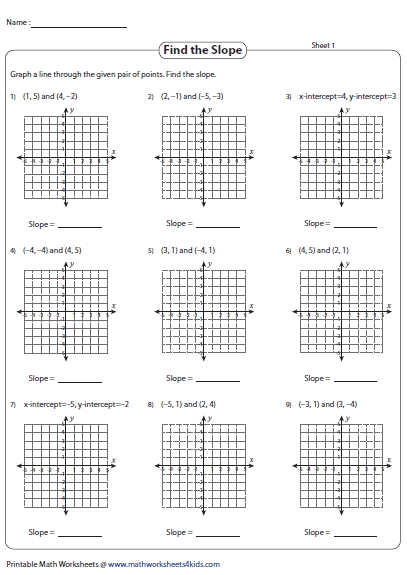
Slope Formula Worksheet
Now, it’s time to practice using the slope formula. Here’s a worksheet with 10 problems to help you get started:
| Problem | Points | Slope |
|---|---|---|
| 1 | (1, 2) and (3, 4) | ______ |
| 2 | (2, 3) and (4, 5) | ______ |
| 3 | (0, 1) and (2, 3) | ______ |
| 4 | (1, 2) and (2, 4) | ______ |
| 5 | (3, 4) and (5, 6) | ______ |
| 6 | (2, 1) and (4, 3) | ______ |
| 7 | (1, 3) and (3, 5) | ______ |
| 8 | (0, 2) and (2, 4) | ______ |
| 9 | (3, 2) and (5, 4) | ______ |
| 10 | (2, 3) and (4, 6) | ______ |
📝 Note: Fill in the slopes for each problem using the slope formula.
Tips and Variations
- The slope formula can be used to calculate the slope of a line given two points, or to find the equation of a line given the slope and one point.
- The slope formula can also be used to calculate the slope of a line perpendicular to a given line.
- In some cases, the slope formula may result in a negative slope. This indicates that the line slopes downward from left to right.
📝 Note: When working with negative slopes, make sure to keep the negative sign when dividing the rise by the run.
In conclusion, the slope formula is a powerful tool for calculating the steepness of a line. With practice and patience, you can master the slope formula and apply it to a wide range of real-world problems. Remember to fill in the slopes for each problem in the worksheet above to reinforce your understanding.
What is the slope formula?
+The slope formula is a mathematical formula used to calculate the slope of a line. It is calculated by dividing the vertical change (rise) by the horizontal change (run).
How do I use the slope formula?
+To use the slope formula, identify two points on the line, calculate the rise (y2 - y1) and run (x2 - x1), and divide the rise by the run to get the slope (m).
What is the slope of a line perpendicular to a given line?
+The slope of a line perpendicular to a given line is the negative reciprocal of the slope of the given line.
Related Terms:
- Slope formula worksheet answer key
- Slope formula Worksheet pdf
- Slope worksheet
- Point-slope form worksheet



