Simplify Trigonometry: Master Trig Expressions Made Easy
Unlocking the Secrets of Trigonometry: A Comprehensive Guide
Trigonometry, a branch of mathematics that deals with the relationships between the sides and angles of triangles, can be a daunting subject for many students. However, with the right approach and techniques, trigonometry can be simplified and made easy to understand. In this article, we will explore the world of trigonometry and provide you with a comprehensive guide on how to master trig expressions.
Understanding the Basics of Trigonometry
Before we dive into the world of trig expressions, it’s essential to understand the basics of trigonometry. Trigonometry is based on the relationships between the angles and side lengths of triangles. The most common trigonometric relationships are:
- Sine (sin): The ratio of the length of the side opposite the angle to the length of the hypotenuse.
- Cosine (cos): The ratio of the length of the side adjacent to the angle to the length of the hypotenuse.
- Tangent (tan): The ratio of the length of the side opposite the angle to the length of the side adjacent to the angle.
These relationships can be represented using the following formulas:
- sin(A) = opposite side / hypotenuse
- cos(A) = adjacent side / hypotenuse
- tan(A) = opposite side / adjacent side
Mastering Trig Expressions
Now that we have a basic understanding of trigonometry, let’s move on to mastering trig expressions. Trig expressions are mathematical expressions that involve trigonometric functions, such as sin, cos, and tan. Here are some tips to help you simplify and master trig expressions:
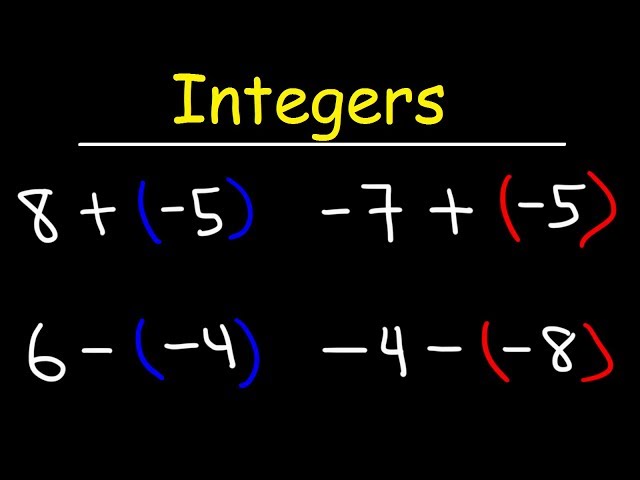
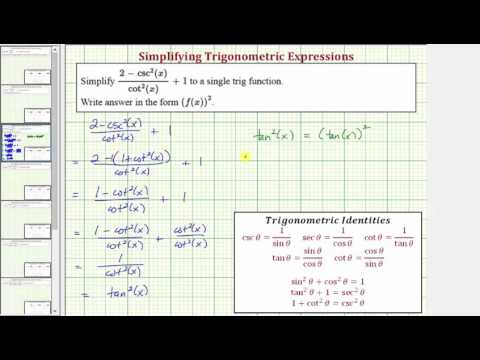
- Use the Pythagorean Identity: The Pythagorean identity states that sin^2(A) + cos^2(A) = 1. This identity can be used to simplify trig expressions by substituting sin^2(A) or cos^2(A) with 1 - cos^2(A) or 1 - sin^2(A), respectively.
- Use Trig Tables: Trig tables are tables that list the values of sin, cos, and tan for common angles. Using trig tables can help you quickly look up the values of trig functions and simplify trig expressions.
- Use the Sum and Difference Formulas: The sum and difference formulas are used to simplify trig expressions involving the sum or difference of two angles. The formulas are:
- sin(A + B) = sin(A)cos(B) + cos(A)sin(B)
- sin(A - B) = sin(A)cos(B) - cos(A)sin(B)
- cos(A + B) = cos(A)cos(B) - sin(A)sin(B)
- cos(A - B) = cos(A)cos(B) + sin(A)sin(B)
Examples of Simplifying Trig Expressions
Let’s take a look at some examples of simplifying trig expressions using the techniques we’ve learned so far.
- Example 1: Simplify the expression sin^2(A) + cos^2(A)
- Using the Pythagorean identity, we can substitute sin^2(A) + cos^2(A) with 1.
- Therefore, the simplified expression is 1.
- Example 2: Simplify the expression sin(A + 30°)
- Using the sum formula, we can rewrite sin(A + 30°) as sin(A)cos(30°) + cos(A)sin(30°).
- Using trig tables, we can look up the values of sin(30°) and cos(30°) and substitute them into the expression.
- Therefore, the simplified expression is sin(A)(√3/2) + cos(A)(1⁄2).
Practical Applications of Trigonometry
Trigonometry has many practical applications in various fields, including physics, engineering, and computer science. Here are some examples of how trigonometry is used in real-world applications:
- Physics: Trigonometry is used to describe the motion of objects in terms of displacement, velocity, and acceleration.
- Engineering: Trigonometry is used to calculate the stress and strain on buildings and bridges.
- Computer Science: Trigonometry is used in computer graphics to create 3D models and animations.
Conclusion
Trigonometry can be a challenging subject, but with the right approach and techniques, it can be simplified and made easy to understand. By mastering trig expressions and understanding the practical applications of trigonometry, you can unlock the secrets of this powerful branch of mathematics.
What is the Pythagorean identity?
+The Pythagorean identity states that sin^2(A) + cos^2(A) = 1.
What are the sum and difference formulas?
+The sum and difference formulas are used to simplify trig expressions involving the sum or difference of two angles. The formulas are: sin(A + B) = sin(A)cos(B) + cos(A)sin(B) sin(A - B) = sin(A)cos(B) - cos(A)sin(B) cos(A + B) = cos(A)cos(B) - sin(A)sin(B) cos(A - B) = cos(A)cos(B) + sin(A)sin(B)
What are some practical applications of trigonometry?
+Trigonometry has many practical applications in various fields, including physics, engineering, and computer science. Some examples include: Physics: Trigonometry is used to describe the motion of objects in terms of displacement, velocity, and acceleration. Engineering: Trigonometry is used to calculate the stress and strain on buildings and bridges. Computer Science: Trigonometry is used in computer graphics to create 3D models and animations.
Related Terms:
- Simplifying trigonometric expressions worksheet pdf
- Simplifying trig expressions worksheet Kuta
- Simplify Trig expressions