5 Ways to Simplify Radicals
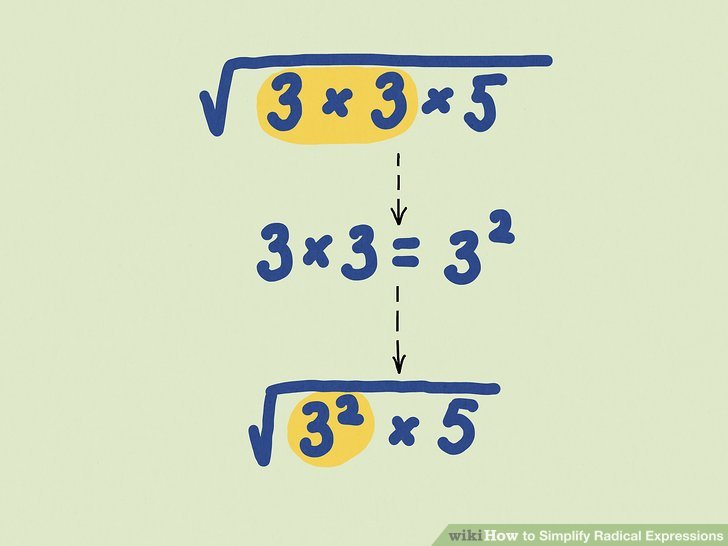
Understanding Radicals and Their Simplification
Radicals, also known as roots, are a way to express a number that, when multiplied by itself, gives a specified value. The process of simplifying radicals is essential in mathematics, especially in algebra and calculus, as it makes solving equations and performing calculations easier. In this article, we will explore five ways to simplify radicals, enhancing your understanding and proficiency in handling these mathematical expressions.
Method 1: Breaking Down Radicals into Prime Factors
One of the most effective ways to simplify radicals is by breaking down the number under the radical sign into its prime factors. This method is especially useful for radicals that are not perfect squares or cubes.
🤔 Note: Understanding prime factorization is key to simplifying radicals effectively.
To illustrate this method, consider the expression √48. Instead of leaving it in this form, we can break down 48 into its prime factors:
48 = 2 × 2 × 2 × 2 × 3
Now, we can rewrite the expression as:
√(2 × 2 × 2 × 2 × 3)
Since we have pairs of 2, we can simplify this further:
√(2^2 × 2^2 × 3) = √(4 × 4 × 3) = 4√3
This method simplifies the radical expression by pulling out any perfect squares or cubes from under the radical sign.
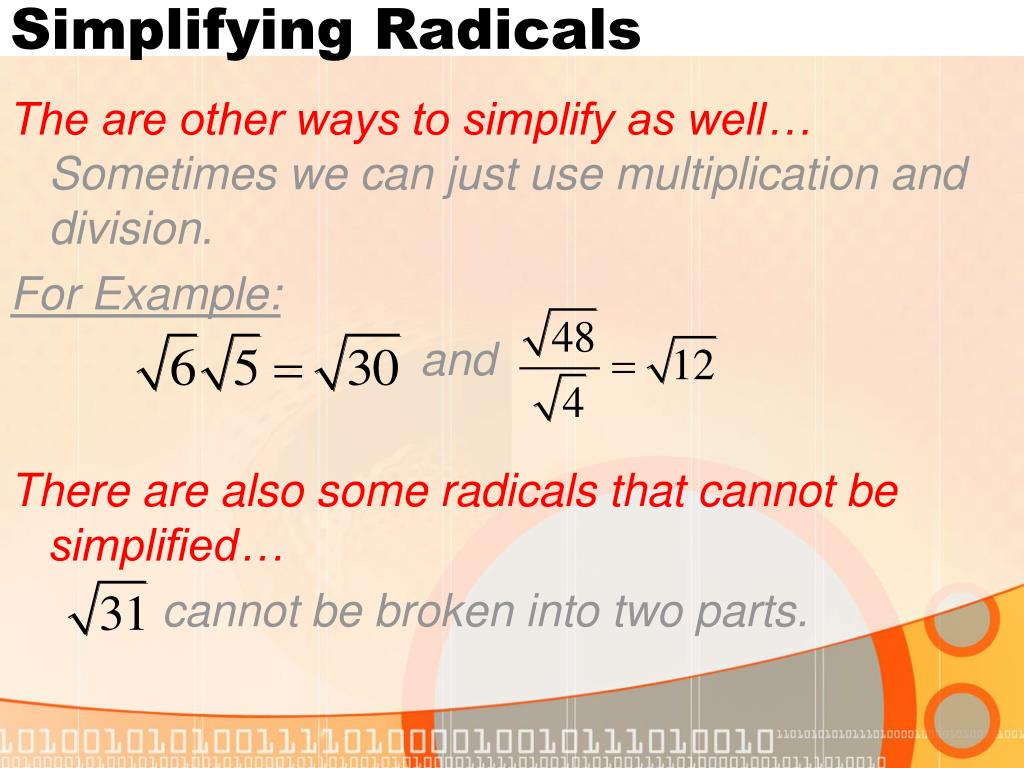
Method 2: Using the Product Rule for Radicals
The product rule for radicals states that the square root of a product is the same as the product of the square roots. Mathematically, this is represented as:
√(ab) = √a × √b
This rule can be used to simplify radicals by breaking down the product under the radical sign into separate square roots.
For example, consider the expression √(12 × 3). Using the product rule, we can rewrite this as:
√12 × √3
Now, we can simplify the expression further by finding the square root of 12:
√12 = √(4 × 3) = √4 × √3 = 2√3
So, the simplified expression is:
2√3 × √3 = 2 × 3 = 6
This method is particularly useful when dealing with radicals that involve products of numbers.
Method 3: Using the Quotient Rule for Radicals
Similar to the product rule, the quotient rule for radicals states that the square root of a quotient is the same as the quotient of the square roots. Mathematically, this is represented as:
√(a/b) = √a / √b
This rule can be used to simplify radicals by breaking down the quotient under the radical sign into separate square roots.
For example, consider the expression √(16⁄4). Using the quotient rule, we can rewrite this as:
√16 / √4
Now, we can simplify the expression further by finding the square root of 16 and 4:
√16 = 4 √4 = 2
So, the simplified expression is:
4⁄2 = 2
This method is particularly useful when dealing with radicals that involve quotients of numbers.
Method 4: Simplifying Radicals with Coefficients
Sometimes, radicals may have coefficients in front of them. In these cases, we can simplify the radical by first simplifying the coefficient, and then simplifying the radical expression.
For example, consider the expression 3√12. We can start by simplifying the coefficient 3, which in this case is already in its simplest form. Then, we can simplify the radical expression:
√12 = √(4 × 3) = √4 × √3 = 2√3
So, the simplified expression is:
3 × 2√3 = 6√3
This method is particularly useful when dealing with radicals that have coefficients.
Method 5: Rationalizing the Denominator
When working with radicals, it’s often necessary to rationalize the denominator, which means removing any radicals from the denominator.
For example, consider the expression 1/√2. To rationalize the denominator, we can multiply both the numerator and denominator by √2:
1/√2 = (1 × √2) / (√2 × √2) = √2 / 2
This method is particularly useful when dealing with radicals that have denominators.
| Method | Description |
|---|---|
| Breaking Down Radicals into Prime Factors | Break down the number under the radical sign into its prime factors to simplify the radical expression. |
| Using the Product Rule for Radicals | Use the product rule to break down the product under the radical sign into separate square roots. |
| Using the Quotient Rule for Radicals | Use the quotient rule to break down the quotient under the radical sign into separate square roots. |
| Simplifying Radicals with Coefficients | Simplify the coefficient, and then simplify the radical expression. |
| Rationalizing the Denominator | Remove any radicals from the denominator by multiplying both the numerator and denominator by the radical. |
In conclusion, simplifying radicals is an essential skill in mathematics, and there are several methods to achieve this. By understanding how to break down radicals into prime factors, using the product and quotient rules, simplifying radicals with coefficients, and rationalizing the denominator, you can simplify even the most complex radical expressions.
What is the purpose of simplifying radicals?
+Simplifying radicals makes it easier to solve equations and perform calculations in mathematics.
What is the difference between the product rule and quotient rule for radicals?
+The product rule states that the square root of a product is the same as the product of the square roots, while the quotient rule states that the square root of a quotient is the same as the quotient of the square roots.
How do I rationalize the denominator of a radical expression?
+To rationalize the denominator, multiply both the numerator and denominator by the radical in the denominator.
Related Terms:
- Simplify Radicals with variables Worksheet
- Simplifying Radicals Worksheet pdf
- Multiplying radicals worksheet
- Dividing Radicals worksheet