6 Ways to Master Similar Triangles Worksheet Answer Key
Unlocking the Secrets of Similar Triangles: A Comprehensive Guide
Similar triangles are a fundamental concept in geometry, and mastering them can be a game-changer for students and professionals alike. In this article, we’ll delve into the world of similar triangles, exploring what they are, how to identify them, and providing a step-by-step guide to solving problems. By the end of this journey, you’ll be equipped with the skills to tackle even the most challenging similar triangles worksheet with confidence.
What are Similar Triangles?
Similar triangles are triangles that have the same shape, but not necessarily the same size. This means that corresponding angles are equal, and the corresponding sides are in proportion. The concept of similar triangles is based on the idea that if two triangles have the same angles, their sides will be proportional, regardless of their size.
Key Properties of Similar Triangles
Before we dive into the world of similar triangles, it’s essential to understand the key properties that define them. These properties include:
- Angle-Angle (AA) Similarity: If two triangles have two pairs of congruent angles, then the triangles are similar.
- Side-Side-Side (SSS) Similarity: If two triangles have three pairs of congruent sides, then the triangles are similar.
- Side-Angle-Side (SAS) Similarity: If two triangles have two pairs of congruent sides and the included angle is congruent, then the triangles are similar.
How to Identify Similar Triangles
Identifying similar triangles can be a daunting task, but with practice and patience, you’ll become a pro in no time. Here are some tips to help you identify similar triangles:
- Look for Congruent Angles: If two triangles have congruent angles, it’s likely that they are similar.
- Check for Proportional Sides: If the corresponding sides of two triangles are in proportion, it’s a strong indication that they are similar.
- Use the AA, SSS, and SAS Postulates: Apply the postulates to determine if two triangles are similar.
Solving Similar Triangles Problems: A Step-by-Step Guide
Now that we’ve covered the basics, let’s dive into the world of solving similar triangles problems. Here’s a step-by-step guide to help you master similar triangles:
- Step 1: Read the Problem Carefully: Take your time to read the problem and understand what’s being asked.
- Step 2: Identify the Similar Triangles: Use the tips mentioned earlier to identify the similar triangles.
- Step 3: Write the Proportion: Write the proportion using the corresponding sides of the similar triangles.
- Step 4: Solve the Proportion: Solve the proportion to find the unknown side or angle.
- Step 5: Check Your Work: Verify your answer by plugging it back into the original equation.
Example Problems and Solutions
Let’s put our knowledge to the test with some example problems and solutions.
Problem 1:
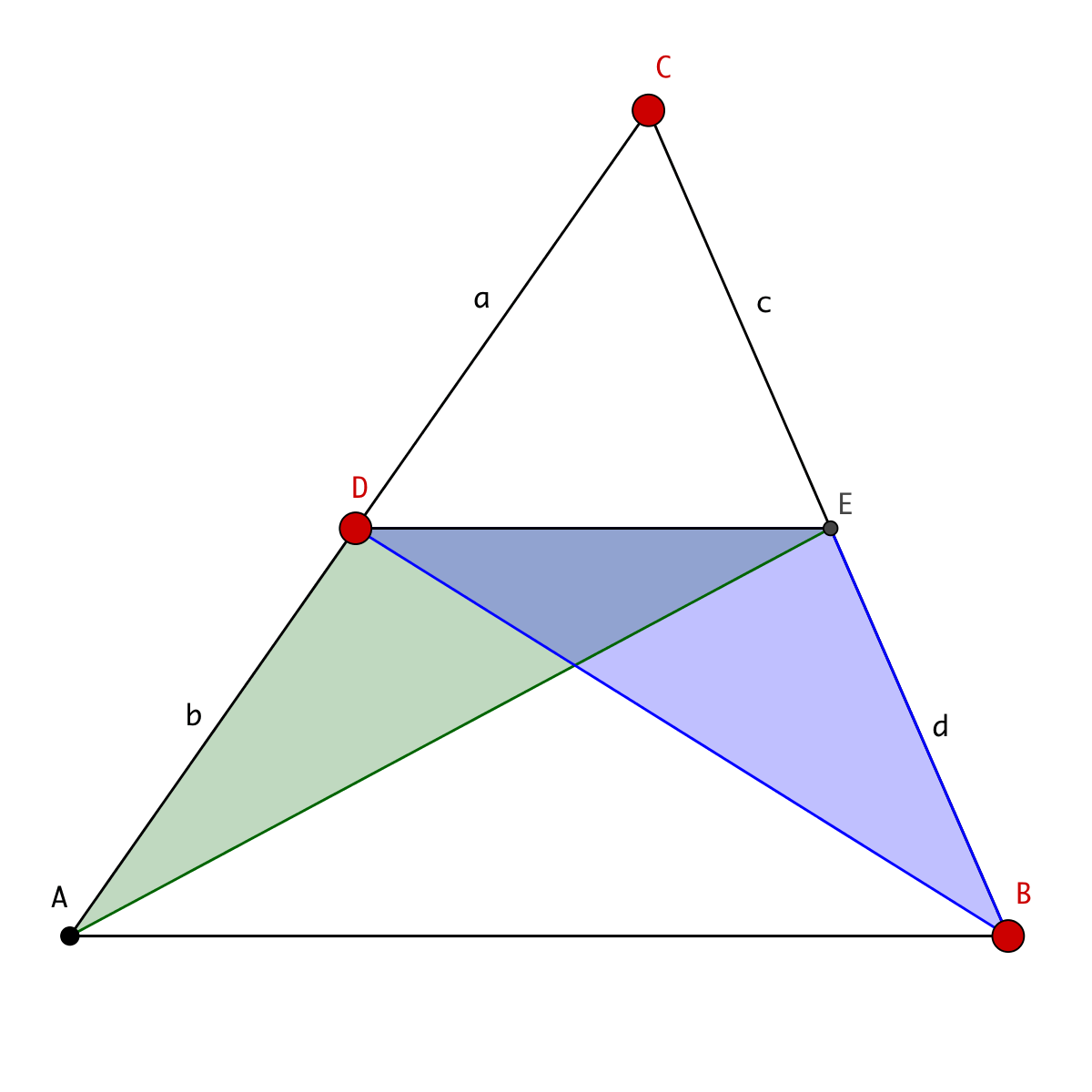
In the diagram below, ΔABC and ΔDEF are similar triangles. Find the length of side DE.
| ΔABC | ΔDEF |
| AB = 6 | DE = x |
| BC = 8 | EF = 4 |
| AC = 10 | DF = 5 |
Solution:
Since ΔABC and ΔDEF are similar triangles, we can write the proportion:
AB/DE = BC/EF = AC/DF
6/x = 8⁄4 = 10⁄5
Solving the proportion, we get:
x = 3
Therefore, the length of side DE is 3.
Problem 2:
In the diagram below, ΔGHI and ΔJKL are similar triangles. Find the measure of angle J.
| ΔGHI | ΔJKL |
| ∠G = 60° | ∠J = x |
| ∠H = 80° | ∠K = 80° |
| ∠I = 40° | ∠L = 40° |
Solution:
Since ΔGHI and ΔJKL are similar triangles, we can use the AA postulate to conclude that ∠J = ∠G = 60°.
Therefore, the measure of angle J is 60°.
📝 Note: Remember to always check your work by plugging your answer back into the original equation.
Conclusion
Mastering similar triangles is a skill that takes practice, patience, and persistence. By following the steps outlined in this guide, you’ll be well on your way to becoming a similar triangles expert. Remember to always read the problem carefully, identify the similar triangles, write the proportion, solve the proportion, and check your work.
What is the difference between congruent and similar triangles?
+Congruent triangles are triangles that have the same size and shape. Similar triangles, on the other hand, are triangles that have the same shape, but not necessarily the same size.
How do I know if two triangles are similar?
+You can use the AA, SSS, and SAS postulates to determine if two triangles are similar.
What is the purpose of writing a proportion in similar triangles?
+Writing a proportion allows you to set up an equation that relates the corresponding sides of the similar triangles, making it easier to solve for the unknown side or angle.
Related Terms:
- Similar triangles Worksheet PDF
- Parts of similar triangles Worksheet