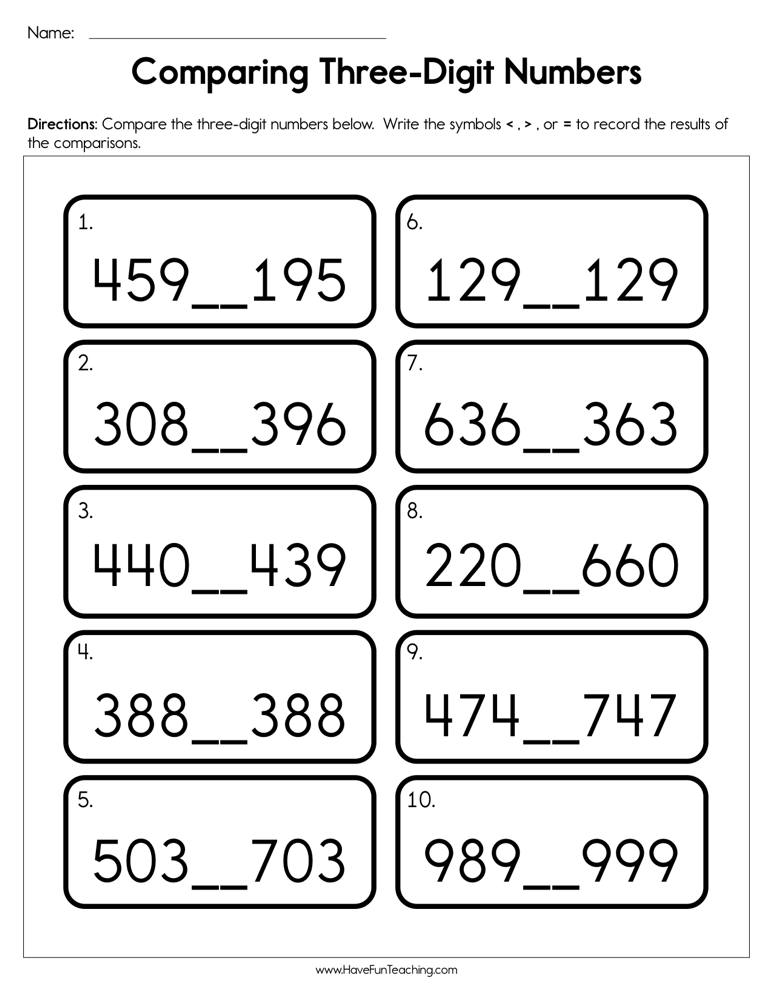
Similar Triangles Worksheet for Easy Geometry Practice
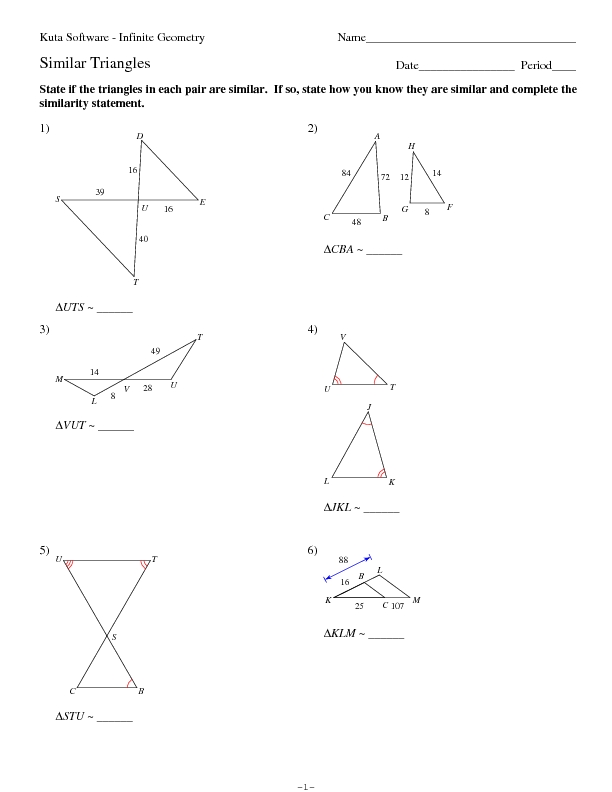
Similar Triangles: Unlocking the Secrets of Geometry
Geometry can be a fascinating and rewarding subject, but it can also be intimidating, especially when it comes to similar triangles. However, with the right tools and practice, anyone can master the concepts of similar triangles. In this article, we will explore the world of similar triangles, and provide you with a comprehensive worksheet to help you practice and improve your skills.
What are Similar Triangles?
Similar triangles are triangles that have the same shape, but not necessarily the same size. This means that corresponding angles are equal and the corresponding sides are in proportion. Similar triangles are used to solve problems involving scaling, proportions, and geometry.
Types of Similar Triangles
There are several types of similar triangles, including:
- AA (Angle-Angle) Similarity: If two triangles have two pairs of congruent angles, then the triangles are similar.
- SAS (Side-Angle-Side) Similarity: If two triangles have two pairs of congruent sides and the included angle is congruent, then the triangles are similar.
- SSS (Side-Side-Side) Similarity: If two triangles have three pairs of congruent sides, then the triangles are similar.
Properties of Similar Triangles
Similar triangles have several important properties, including:
- Corresponding Angles are Equal: If two triangles are similar, then corresponding angles are equal.
- Corresponding Sides are Proportional: If two triangles are similar, then corresponding sides are proportional.
- Perimeters are Proportional: If two triangles are similar, then their perimeters are proportional.
Worksheet: Similar Triangles Practice
Now that we have explored the basics of similar triangles, it’s time to put your knowledge to the test with our comprehensive worksheet. This worksheet includes 20 questions to help you practice and improve your skills in similar triangles.
Section 1: Multiple Choice
Which of the following is a characteristic of similar triangles? a) Corresponding angles are equal b) Corresponding sides are equal c) Perimeters are equal d) All of the above
If two triangles have two pairs of congruent angles, what can be concluded about the triangles? a) They are congruent b) They are similar c) They are right triangles d) They are isosceles triangles
If two triangles have three pairs of congruent sides, what can be concluded about the triangles? a) They are congruent b) They are similar c) They are right triangles d) They are isosceles triangles
Section 2: Short Answer
What is the difference between AA similarity and SAS similarity?
If two triangles are similar, what can be concluded about their perimeters?
Section 3: Word Problems
In a blueprint, a triangle has a perimeter of 12 inches. If the blueprint is scaled down to 75% of its original size, what is the perimeter of the new triangle?
A model of a building has a height of 10 inches and a base of 5 inches. If the model is scaled up to 150% of its original size, what is the height and base of the new model?
Section 4: Proofs
Prove that if two triangles have two pairs of congruent angles, then the triangles are similar.
Prove that if two triangles have three pairs of congruent sides, then the triangles are similar.
Section 5: Applications
A photographer is taking a picture of a building. If the camera is 100 feet away from the building, and the angle of elevation is 60 degrees, what is the height of the building?
A surveyor is measuring the distance between two points on a map. If the map is scaled at 1:1000, and the distance on the map is 10 cm, what is the actual distance between the two points?
📝 Note: Make sure to show all work and explain your reasoning for each problem.
Answer Key
Section 1: Multiple Choice
- a) Corresponding angles are equal
- b) They are similar
- b) They are similar
Section 2: Short Answer
- AA similarity requires two pairs of congruent angles, while SAS similarity requires two pairs of congruent sides and the included angle is congruent.
- If two triangles are similar, their perimeters are proportional.
Section 3: Word Problems
- 9 inches
- Height: 15 inches, Base: 7.5 inches
Section 4: Proofs
- Use the AA similarity theorem to prove that the triangles are similar.
- Use the SSS similarity theorem to prove that the triangles are similar.
Section 5: Applications
- 50 feet
- 100 meters
What is the difference between similar triangles and congruent triangles?
+Satisfying one similar triangle theorem is enough to establish similarity between triangles. On the other hand, satisfying one congruence theorem is not enough to establish congruence between triangles. One must satisfy three congruence theorems, known as Side-Side-Side, Side-Angle-Side, and Angle-Side-Angle.
What are some real-world applications of similar triangles?
+Similar triangles are used in many real-world applications, including architecture, engineering, physics, and computer graphics. For example, architects use similar triangles to design buildings and bridges, while engineers use them to calculate stresses and loads on structures. Physicists use similar triangles to describe the motion of objects, and computer graphics artists use them to create 3D models and animations.
How can I use similar triangles to solve problems?
+Similar triangles can be used to solve problems involving scaling, proportions, and geometry. To use similar triangles, identify the corresponding angles and sides of the triangles, and set up a proportion to relate the sides. Then, use the proportion to solve for the unknown side or angle.
In conclusion, similar triangles are a fundamental concept in geometry, and mastering them can help you solve a wide range of problems. With practice and patience, you can become proficient in using similar triangles to solve problems involving scaling, proportions, and geometry.
Related Terms:
- Similar triangles Worksheet answer Key
- Math monks Similar Triangles worksheet