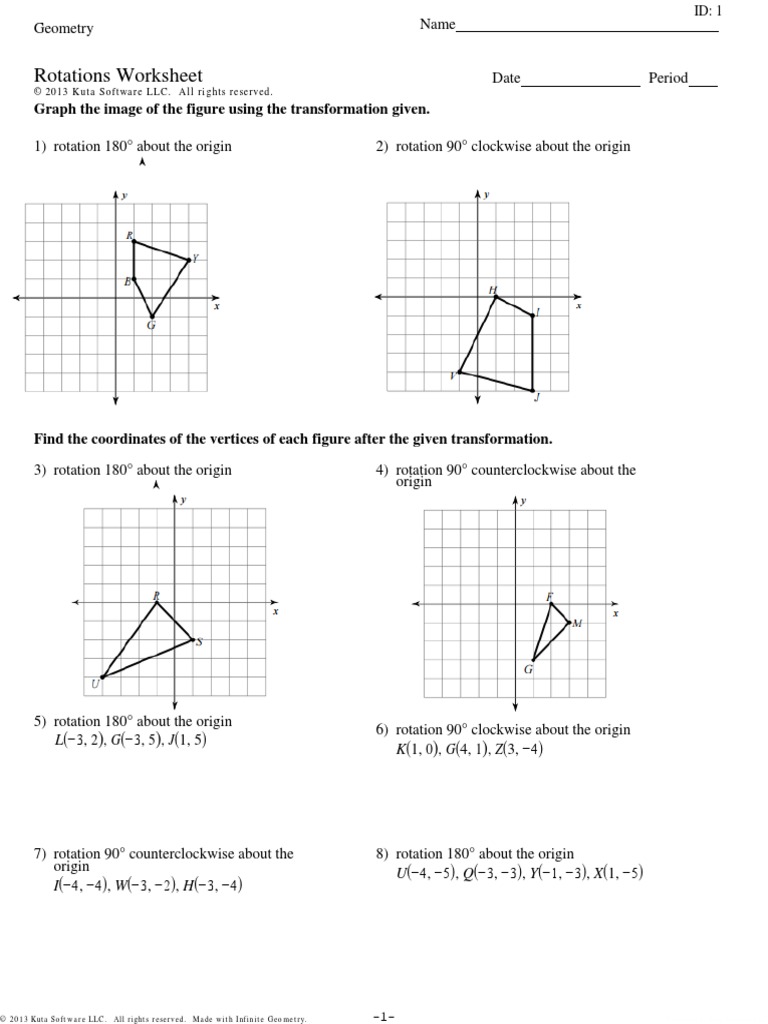
Mastering Rotations with Practice Worksheet Exercises

Unlocking the Power of Rotations: A Comprehensive Guide

Rotations are a fundamental concept in mathematics, particularly in geometry and trigonometry. Mastering rotations is essential for problem-solving in various fields, including physics, engineering, and computer science. In this article, we will delve into the world of rotations, exploring the basics, types, and applications. We will also provide a set of practice worksheet exercises to help you reinforce your understanding of rotations.

What are Rotations?

A rotation is a transformation that turns a figure around a fixed point, called the center of rotation. The amount of rotation is measured in degrees, with 360 degrees representing a full rotation. Rotations can be clockwise or counterclockwise, and they can be represented using various notation systems, including degrees, radians, and matrices.

Types of Rotations

There are several types of rotations, including:

• 90-degree rotation: A rotation of 90 degrees, also known as a quarter turn.
• 180-degree rotation: A rotation of 180 degrees, also known as a half turn.
• 360-degree rotation: A rotation of 360 degrees, also known as a full turn.
• Rotational symmetry: A rotation that leaves a figure unchanged, often used in geometry and art.

Applications of Rotations

Rotations have numerous applications in various fields, including:

• Physics: Rotations are used to describe the motion of objects in space, including the rotation of planets, stars, and galaxies.
• Engineering: Rotations are used in the design of mechanical systems, including gears, pulleys, and levers.
• Computer Science: Rotations are used in computer graphics, game development, and robotics.

Practice Worksheet Exercises

Now that we have explored the basics of rotations, it’s time to practice! Here are some exercises to help you reinforce your understanding of rotations:

Exercise 1: 90-degree Rotation

Rotate the following figure 90 degrees clockwise:

  *
 / \
/___\

Exercise 2: 180-degree Rotation

Rotate the following figure 180 degrees counterclockwise:

______
|       |
|  O   |
|       |
______

Exercise 3: Rotational Symmetry

Identify the rotational symmetry of the following figure:

  *
 / \
/___\
  | |
  | |

Exercise 4: Matrix Rotation

Use the following rotation matrix to rotate the point (2, 3) 45 degrees clockwise:

[cos(45) -sin(45)]
[sin(45)  cos(45)]

Exercise 5: Real-World Application

Describe a real-world application of rotations in physics, engineering, or computer science.

📝 Note: Remember to show your work and explain your reasoning for each exercise.

Rotations in Mathematics

Rotations are a fundamental concept in mathematics, particularly in geometry and trigonometry. In geometry, rotations are used to describe the transformation of figures, while in trigonometry, rotations are used to solve problems involving triangles and circles.

Conclusion

Mastering rotations is essential for problem-solving in various fields. By understanding the basics of rotations, types of rotations, and applications, you can develop a strong foundation in mathematics and science. Remember to practice regularly and apply your knowledge to real-world problems.

Related Terms:

• Rotations Practice Worksheet Answer Key
• Rotation worksheet with answers PDF
• Rotation Worksheet pdf
• Transformation Worksheet pdf
• Worksheet transformations geometry