5 Ways to Master Right Triangle Problems
Understanding Right Triangle Problems
Right triangle problems are a fundamental concept in geometry and trigonometry. They involve finding the lengths of the sides of a right triangle, given certain information about the triangle. Mastering right triangle problems requires a strong understanding of the relationships between the sides and angles of a right triangle. In this article, we will explore five ways to master right triangle problems.
1. Learn the Pythagorean Theorem
The Pythagorean Theorem is a fundamental concept in geometry that describes the relationship between the lengths of the sides of a right triangle. The theorem states that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. This can be expressed mathematically as:
a² + b² = c²
where a and b are the lengths of the two sides that form the right angle, and c is the length of the hypotenuse.
📝 Note: The Pythagorean Theorem only works for right triangles, so make sure you are dealing with a right triangle before applying the theorem.
2. Understand Trigonometric Ratios
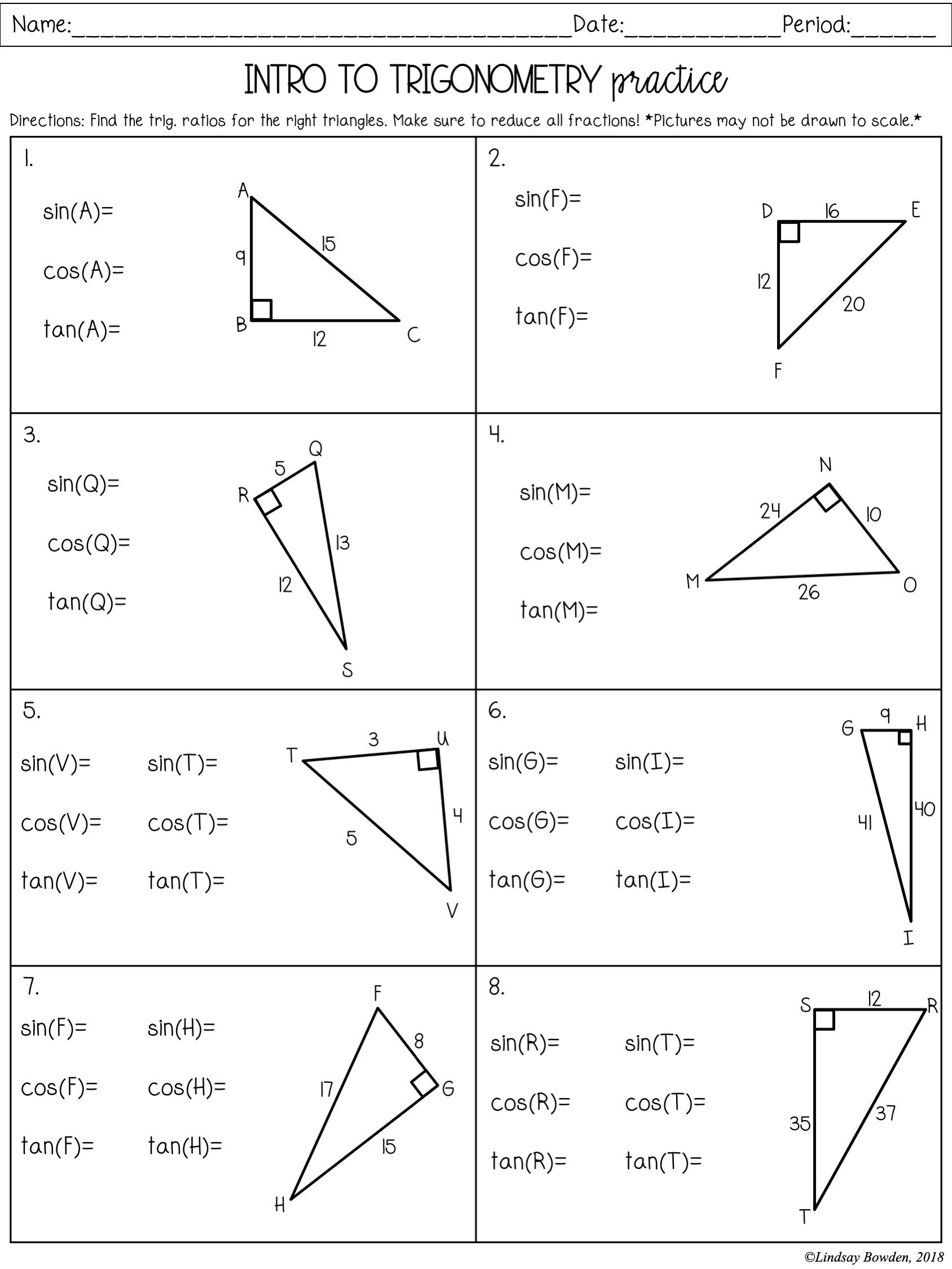
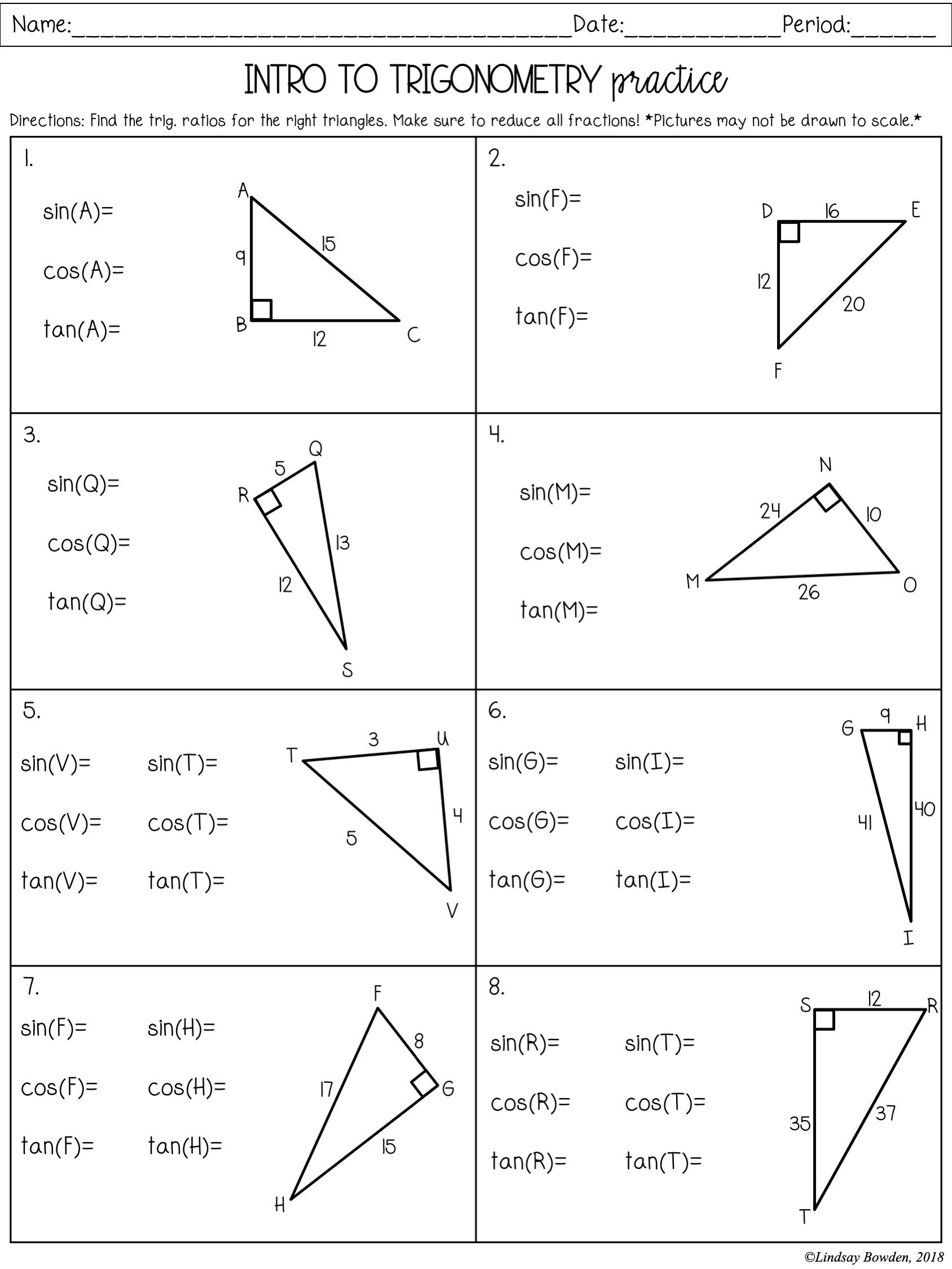
Trigonometric ratios are used to describe the relationships between the angles and sides of a right triangle. The three main trigonometric ratios are:
- Sine (sin): the ratio of the length of the side opposite the angle to the length of the hypotenuse
- Cosine (cos): the ratio of the length of the side adjacent to the angle to the length of the hypotenuse
- Tangent (tan): the ratio of the length of the side opposite the angle to the length of the side adjacent to the angle
These ratios can be used to find the lengths of the sides of a right triangle, given certain information about the triangle.
3. Practice, Practice, Practice
Practice is key to mastering right triangle problems. Start with simple problems and work your way up to more complex ones. Use online resources, such as Khan Academy or Mathway, to practice solving right triangle problems.
Here are some examples of right triangle problems:
- Find the length of the hypotenuse of a right triangle with legs of length 3 and 4.
- Find the length of the side opposite a 30° angle in a right triangle with a hypotenuse of length 10.
- Find the length of the side adjacent to a 45° angle in a right triangle with a hypotenuse of length 10.
4. Use Right Triangle Tables and Charts
Right triangle tables and charts can be useful tools for solving right triangle problems. These tables and charts provide pre-calculated values for common right triangle angles and side lengths.
For example, the following table provides the values of the sine, cosine, and tangent of common angles:
| Angle | Sine | Cosine | Tangent |
|---|---|---|---|
| 30° | 0.5 | 0.866 | 0.577 |
| 45° | 0.707 | 0.707 | 1 |
| 60° | 0.866 | 0.5 | 1.732 |
5. Learn to Draw Diagrams
Drawing diagrams is an important skill for solving right triangle problems. A diagram can help you visualize the problem and identify the relationships between the sides and angles.
When drawing a diagram, make sure to include the following:
- The right angle
- The lengths of the sides (if given)
- The angles (if given)
Here is an example of a diagram for a right triangle problem:
Find the length of the hypotenuse of a right triangle with legs of length 3 and 4.
In conclusion, mastering right triangle problems requires a strong understanding of the relationships between the sides and angles of a right triangle. By learning the Pythagorean Theorem, understanding trigonometric ratios, practicing, using right triangle tables and charts, and learning to draw diagrams, you can become proficient in solving right triangle problems.
What is the Pythagorean Theorem?
+The Pythagorean Theorem is a mathematical concept that describes the relationship between the lengths of the sides of a right triangle. It states that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
What are trigonometric ratios?
+Trigonometric ratios are used to describe the relationships between the angles and sides of a right triangle. The three main trigonometric ratios are sine, cosine, and tangent.
How can I practice solving right triangle problems?
+You can practice solving right triangle problems using online resources, such as Khan Academy or Mathway. Start with simple problems and work your way up to more complex ones.
Related Terms:
- Special Right triangle Worksheet
- Special Right Triangles Worksheet pdf