Reflections and Translations Math Worksheet Made Easy
Understanding Reflections and Translations in Math
Reflections and translations are two fundamental concepts in geometry and math that help us understand how shapes and objects move and change in different ways. In this article, we will delve into the world of reflections and translations, explore their definitions, and provide a comprehensive guide on how to tackle math problems related to these concepts.
What are Reflections in Math?
A reflection in math is a transformation that flips a shape or object over a line, called the line of reflection. This line acts as a mirror, and the shape is reflected on the other side of the line, creating a mirror image. The line of reflection can be a horizontal line, a vertical line, or even a diagonal line.
Key Properties of Reflections:
- The original shape and the reflected shape are congruent (same size and shape).
- The line of reflection is the perpendicular bisector of the segment connecting the original shape and the reflected shape.
- The reflected shape has the same orientation as the original shape.
What are Translations in Math?
A translation in math is a transformation that moves a shape or object from one location to another without changing its size or orientation. This means that the shape is slid or moved to a new position, without being rotated or reflected.
Key Properties of Translations:
- The original shape and the translated shape are congruent (same size and shape).
- The translated shape has the same orientation as the original shape.
- The translation can be represented by a vector, which shows the direction and distance of the translation.
How to Perform Reflections and Translations
Now that we have a good understanding of what reflections and translations are, let’s dive into how to perform them.
Reflections:
- Identify the line of reflection.
- Draw a perpendicular line from the original shape to the line of reflection.
- Draw a segment from the point of intersection to the reflected shape.
- The reflected shape should be the same size and shape as the original shape.
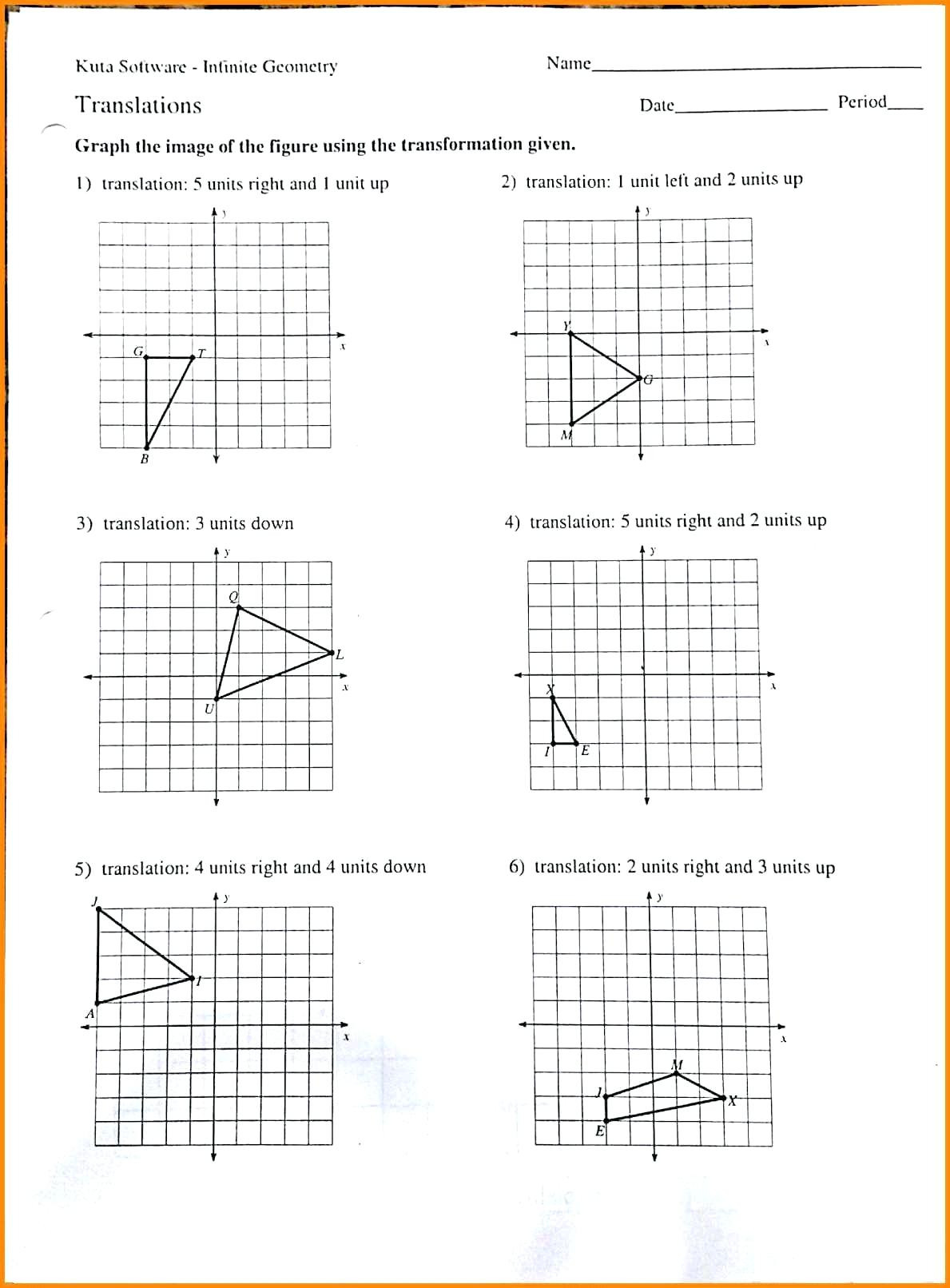
Translations:
- Identify the vector of translation (direction and distance).
- Draw a line from the original shape to the translated shape, using the vector as a guide.
- Move the original shape along the line, using the vector as a guide.
- The translated shape should be the same size and shape as the original shape.
📝 Note: When performing reflections and translations, it's essential to use graph paper or a coordinate plane to ensure accuracy and precision.
Real-World Applications of Reflections and Translations
Reflections and translations have numerous real-world applications in various fields, including:
- Architecture: Reflections and translations are used to design symmetrical buildings and structures.
- Engineering: Translations are used to move objects or machines from one location to another.
- Art: Reflections and translations are used to create symmetrical and visually appealing designs.
Common Mistakes to Avoid
When working with reflections and translations, it’s essential to avoid common mistakes that can lead to incorrect answers. Here are some common mistakes to watch out for:
- Failing to use graph paper or a coordinate plane, leading to inaccurate drawings.
- Not identifying the line of reflection or vector of translation correctly.
- Not using the correct properties of reflections and translations.
📝 Note: Practice, practice, practice! The more you practice working with reflections and translations, the more comfortable you'll become with these concepts.
What is the main difference between a reflection and a translation?
+A reflection flips a shape over a line, while a translation moves a shape from one location to another without changing its size or orientation.
How do I know which line to use as the line of reflection?
+The line of reflection is usually indicated by a dotted line or a clear indication in the problem. If not, you can use the properties of reflections to determine the line of reflection.
Can a translation be represented by a vector?
+Yes, a translation can be represented by a vector, which shows the direction and distance of the translation.
In conclusion, reflections and translations are fundamental concepts in math that help us understand how shapes and objects move and change. By understanding the definitions, properties, and how to perform these transformations, you’ll be well-equipped to tackle math problems and real-world applications with confidence. Remember to practice regularly and avoid common mistakes to become proficient in these concepts.
Related Terms:
- Reflections and Translations Worksheet PDF
- Reflections and Translations Worksheet answers
- Translations and reflections
- Translation and reflection Worksheet Kuta
- Reflections Worksheet PDF