7 Ways to Master Rational Number Operations
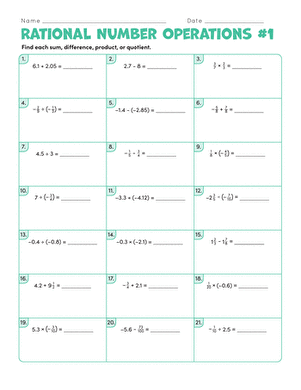
Understanding Rational Numbers
Rational numbers are a fundamental concept in mathematics, and mastering their operations is crucial for success in various mathematical disciplines. A rational number is a number that can be expressed as the quotient or fraction of two integers, with the denominator being non-zero. In other words, it is a number that can be written in the form a/b, where a and b are integers and b is not equal to zero.
Why Mastering Rational Number Operations is Important
Mastering rational number operations is essential for various reasons. Firstly, it helps build a strong foundation in mathematics, which is necessary for advanced mathematical concepts. Secondly, rational numbers are used extensively in real-life applications, such as finance, science, and engineering. Finally, understanding rational number operations enables individuals to solve problems and make informed decisions in various fields.
7 Ways to Master Rational Number Operations
Mastering rational number operations requires practice, patience, and persistence. Here are 7 ways to help you achieve mastery:
1. Understand the Basics
To master rational number operations, it is essential to understand the basics. This includes understanding the concept of rational numbers, their properties, and the different types of rational numbers, such as integers, fractions, and decimals.
- Integers: Whole numbers, either positive, negative, or zero.
- Fractions: Numbers expressed as the quotient of two integers, such as 1⁄2 or 3⁄4.
- Decimals: Numbers expressed as a finite or infinite sequence of digits, such as 0.5 or 3.14.
2. Learn the Rules of Arithmetic Operations
There are four basic arithmetic operations that can be performed on rational numbers: addition, subtraction, multiplication, and division. Each operation has its own set of rules and procedures.
- Addition and Subtraction: When adding or subtracting rational numbers, ensure that the denominators are the same. If not, find the least common multiple (LCM) of the denominators and convert the fractions accordingly.
- Multiplication: When multiplying rational numbers, multiply the numerators and denominators separately.
- Division: When dividing rational numbers, invert the second fraction (i.e., flip the numerator and denominator) and multiply.
3. Practice, Practice, Practice
Practice is key to mastering rational number operations. Start with simple problems and gradually move on to more complex ones. Use online resources, worksheets, or textbooks to practice.
- Simple Problems: 1⁄2 + 1⁄4, 3⁄4 - 1⁄6, 2⁄3 x 3⁄4, 4⁄5 ÷ 2⁄3
- Complex Problems: 2 1⁄2 + 3 3⁄4, 5 1⁄2 - 2 3⁄4, 3 3⁄4 x 2 1⁄2, 4 1⁄2 ÷ 3 3⁄4
4. Use Real-World Examples
Using real-world examples helps to make rational number operations more meaningful and relevant. For instance, calculating the cost of groceries, measuring ingredients for a recipe, or determining the area of a room.
- Example: A recipe requires 2 1⁄4 cups of flour. If you only have a 1⁄4 cup measuring cup, how many times will you need to fill it to get the required amount?
5. Watch Video Tutorials
Video tutorials can provide a visual and interactive way to learn rational number operations. Websites such as Khan Academy, Mathway, and IXL offer video tutorials and practice exercises.
- Khan Academy: Offers video tutorials and practice exercises on various mathematical topics, including rational number operations.
- Mathway: Provides video tutorials and an online calculator to help with mathematical problems, including rational number operations.
6. Join Online Communities
Joining online communities, such as forums or social media groups, can provide a platform to ask questions, share resources, and learn from others.
- Example: Reddit’s r/learnmath community is a great place to ask questions and get help with mathematical problems, including rational number operations.
7. Seek Help When Needed
Don’t be afraid to seek help when needed. Reach out to teachers, classmates, or tutors for support.
- Teachers: Teachers can provide one-on-one support and guidance.
- Classmates: Classmates can offer peer-to-peer support and study groups.
- Tutors: Tutors can provide individualized support and instruction.
📝 Note: Mastering rational number operations takes time and practice. Be patient, persistent, and don't be afraid to seek help when needed.
In conclusion, mastering rational number operations is essential for success in mathematics and real-life applications. By understanding the basics, learning the rules of arithmetic operations, practicing regularly, using real-world examples, watching video tutorials, joining online communities, and seeking help when needed, individuals can achieve mastery over rational number operations.
What is a rational number?
+A rational number is a number that can be expressed as the quotient or fraction of two integers, with the denominator being non-zero.
Why is mastering rational number operations important?
+Mastering rational number operations is essential for building a strong foundation in mathematics, which is necessary for advanced mathematical concepts and real-life applications.
How can I practice rational number operations?
+You can practice rational number operations by using online resources, worksheets, or textbooks. Start with simple problems and gradually move on to more complex ones.
Related Terms:
- Rational number Operations Worksheet PDF
- Operations with rational numbers