5 Ways to Simplify Rational Expressions

Understanding Rational Expressions

Rational expressions are a fundamental concept in algebra, and they can be used to solve a wide range of problems. A rational expression is a fraction that contains polynomials in the numerator and denominator. For example, x/x + 1 is a rational expression. Simplifying rational expressions is an essential skill in algebra, as it allows you to work with more complex equations and expressions.

Why Simplify Rational Expressions?

Simplifying rational expressions is important because it makes it easier to work with them. When a rational expression is in its simplest form, it is easier to add, subtract, multiply, and divide. Simplifying rational expressions also helps to eliminate any restrictions on the domain of the expression. For example, the expression x/x - 1 is undefined when x = 1, but when simplified to 1/(x - 1), it is clear that the expression is undefined when x = 1.

5 Ways to Simplify Rational Expressions

Here are five ways to simplify rational expressions:

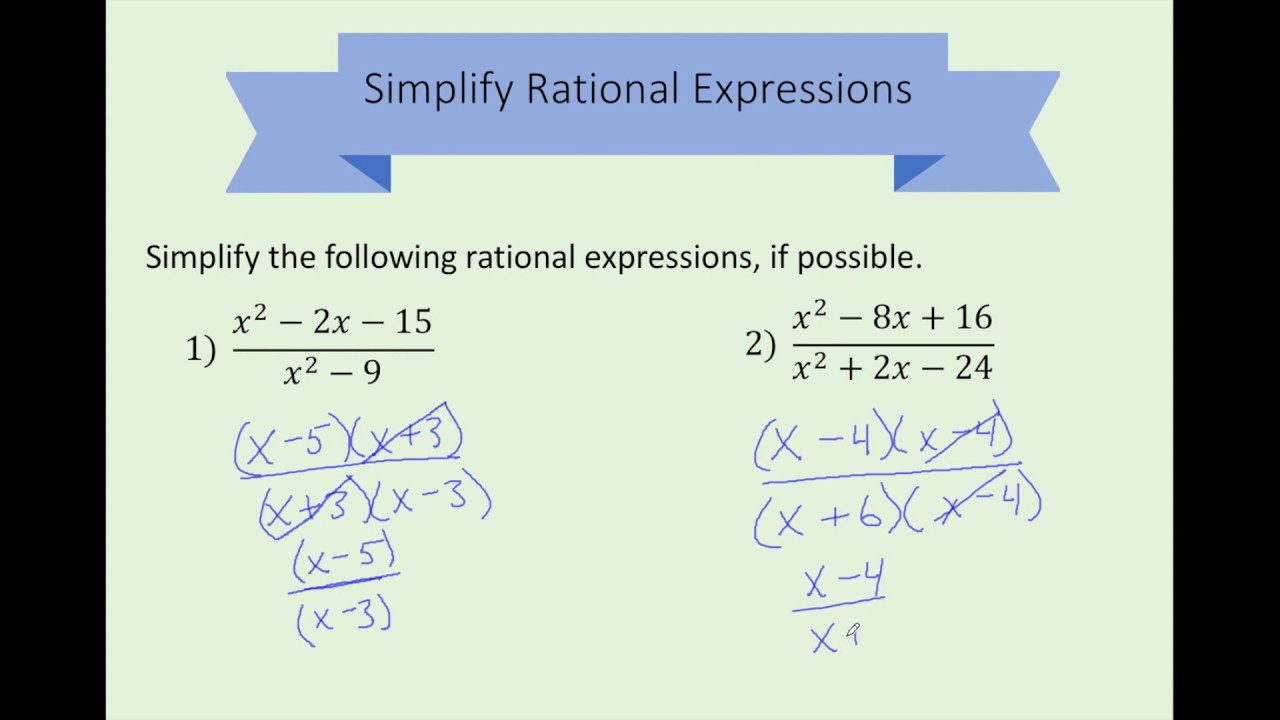
1. Factor the Numerator and Denominator

Factoring the numerator and denominator is a great way to simplify rational expressions. When you factor the numerator and denominator, you can cancel out any common factors, which simplifies the expression.

💡 Note: Make sure to factor the numerator and denominator completely before canceling out any common factors.

Example:

x^2 + 2x + 1 / x^2 - 1

= (x + 1)(x + 1) / (x + 1)(x - 1)

= (x + 1) / (x - 1)

2. Cancel Out Common Factors

Canceling out common factors is a simple way to simplify rational expressions. When you have a common factor in the numerator and denominator, you can cancel it out.

Example:

x^2 + 2x + 1 / x + 1

= (x + 1)(x + 1) / (x + 1)

= x + 1

3. Simplify Complex Fractions

Complex fractions are rational expressions that contain fractions in the numerator or denominator. To simplify complex fractions, you can multiply the numerator and denominator by the reciprocal of the fraction.

Example:

1 / (1 / x + 1)

= (1 / x) / (1 / x + 1)

= 1 / (x + 1)

4. Use the Zero Product Property

The zero product property states that if the product of two factors is zero, then one or both of the factors must be zero. You can use this property to simplify rational expressions.

Example:

x^2 + 2x + 1 / x^2 - 1

= (x + 1)(x + 1) / (x + 1)(x - 1)

= (x + 1) / (x - 1)

5. Combine Like Terms

Combining like terms is a simple way to simplify rational expressions. When you have like terms in the numerator or denominator, you can combine them.

Example:

x^2 + 2x + 1 + x^2 + 2x + 1 / x^2 - 1

= 2x^2 + 4x + 2 / x^2 - 1

= 2(x^2 + 2x + 1) / x^2 - 1

Conclusion

Simplifying rational expressions is an essential skill in algebra. By factoring the numerator and denominator, canceling out common factors, simplifying complex fractions, using the zero product property, and combining like terms, you can simplify even the most complex rational expressions. Remember to always check your work and make sure that the expression is in its simplest form.