5 Ways to Solve Rational Equations Easily

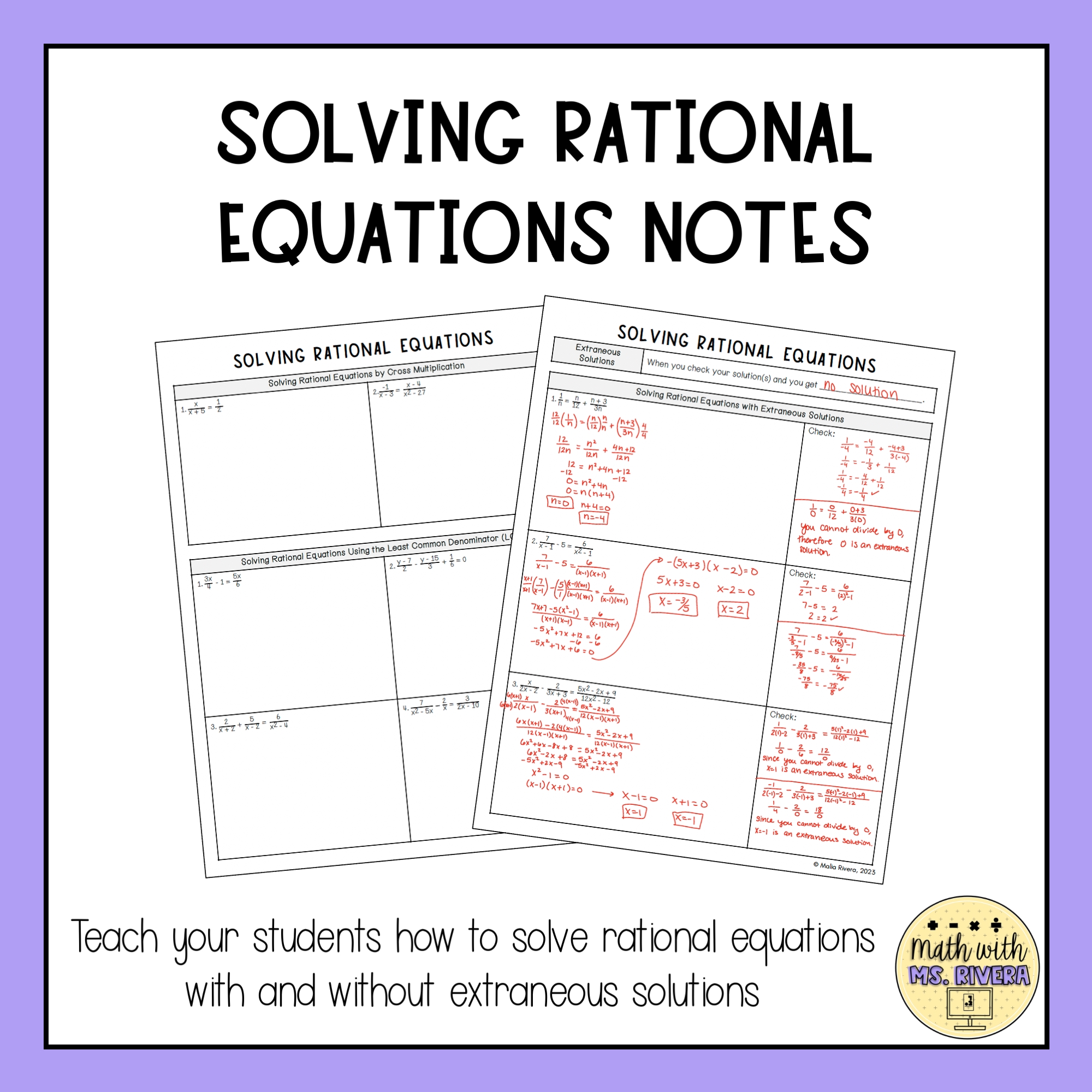
Understanding Rational Equations

Rational equations are a fundamental concept in algebra, and solving them can be a daunting task for many students. A rational equation is an equation that contains one or more rational expressions, which are fractions that have polynomials in the numerator and denominator. In this article, we will discuss five ways to solve rational equations easily.

Method 1: Cross-Multiplication

Cross-multiplication is a simple and effective method for solving rational equations. This method involves multiplying both sides of the equation by the least common multiple (LCM) of the denominators. The LCM is the smallest expression that is divisible by both denominators.

For example, consider the equation:

To solve this equation, we can cross-multiply by multiplying both sides by the LCM, which is 6x:

This simplifies to:

Solving for x, we get:

🤔 Note: When cross-multiplying, make sure to multiply both sides of the equation by the same value to avoid changing the equation's solution.

Method 2: Factoring and Canceling

Another method for solving rational equations is to factor and cancel out common factors. This method involves factoring the numerator and denominator of each rational expression and canceling out any common factors.

For example, consider the equation:

We can factor the numerator of the left-hand side as:

Canceling out the common factor (x + 2), we get:

Solving for x, we get:

Method 3: Finding the Least Common Denominator (LCD)

The least common denominator (LCD) is the smallest expression that is divisible by both denominators. Finding the LCD is an effective method for solving rational equations.

For example, consider the equation:

The LCD of the left-hand side is 2x(x + 1). Multiplying both sides by the LCD, we get:

This simplifies to:

Solving for x, we get:

📝 Note: When finding the LCD, make sure to multiply both sides of the equation by the same value to avoid changing the equation's solution.

Method 4: Using Proportional Reasoning

Proportional reasoning is a method for solving rational equations by using proportions. This method involves setting up a proportion and solving for the variable.

For example, consider the equation:

We can set up a proportion as:

Cross-multiplying, we get:

Solving for x, we get:

Method 5: Graphing

Graphing is a visual method for solving rational equations. This method involves graphing the rational expressions on both sides of the equation and finding the intersection point.

For example, consider the equation:

Graphing the rational expressions, we get:

The intersection point is x = 0.

📊 Note: When graphing, make sure to label the axes and include the key points, such as the x-intercepts and y-intercepts.

In summary, there are several methods for solving rational equations, including cross-multiplication, factoring and canceling, finding the least common denominator, using proportional reasoning, and graphing. By mastering these methods, you can solve rational equations with ease and confidence.

Related Terms:

• solving rational equations worksheet step-by-step
• Graphing Rational Equations Worksheet
• Simple Rational Equations worksheet