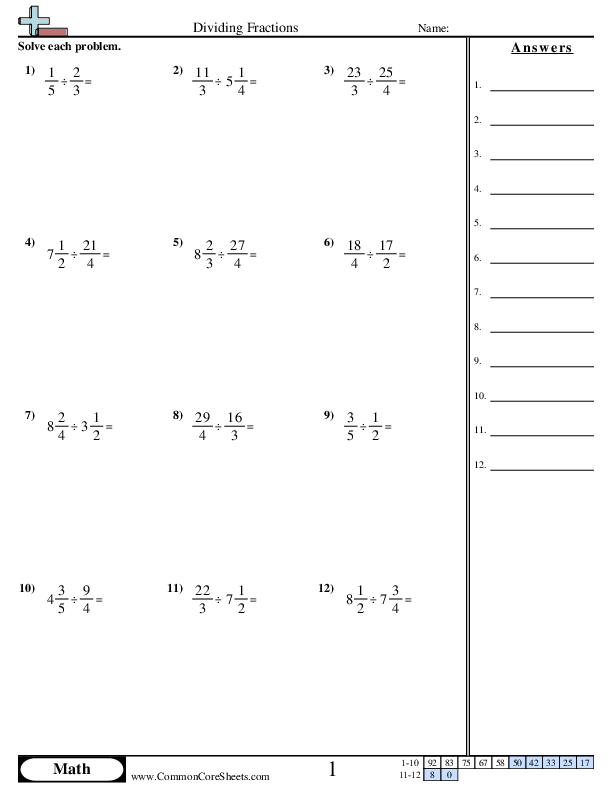
7 Ways to Solve Rate of Change Word Problems
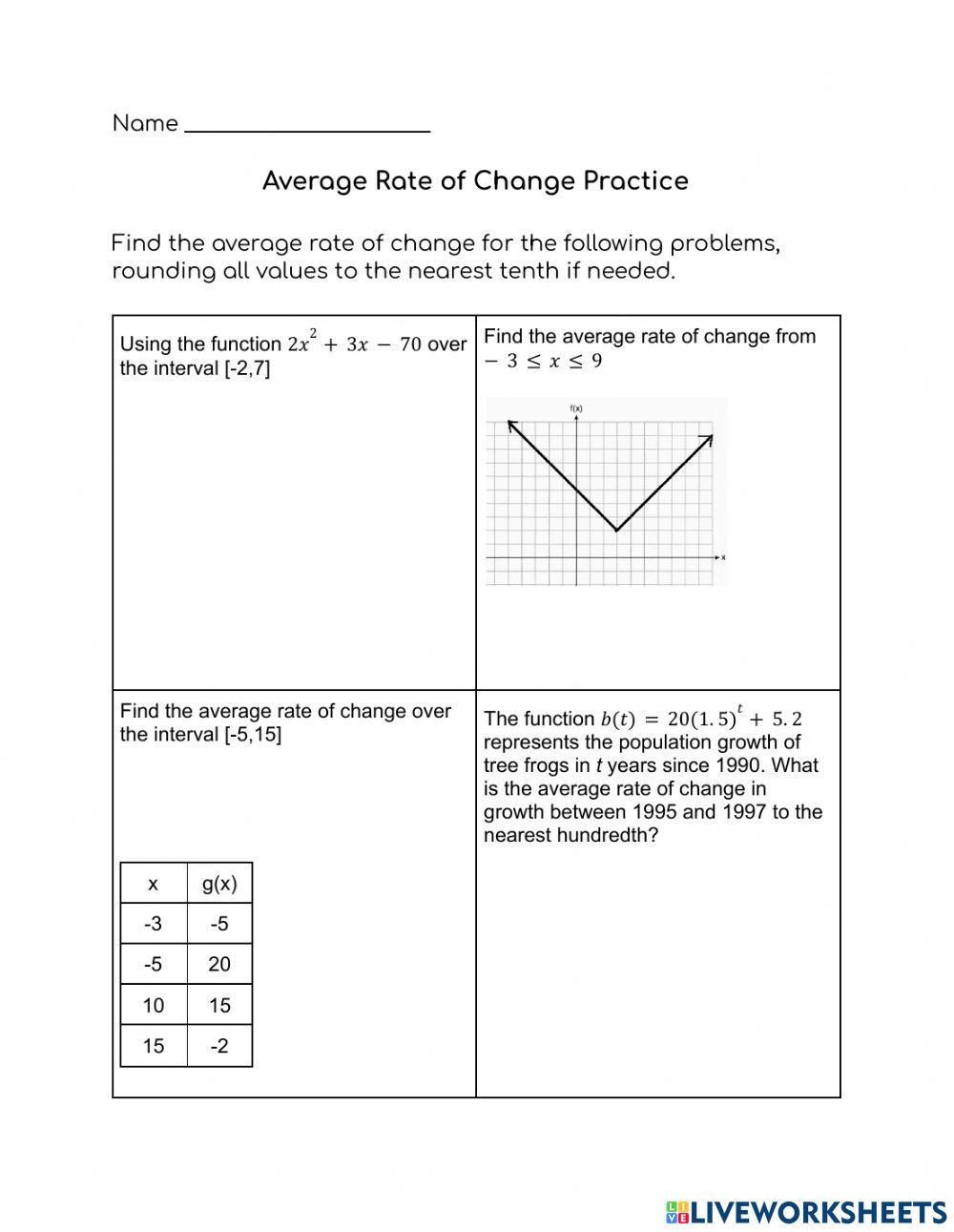
Understanding rate of change is a fundamental concept in mathematics and physics, and it’s used to describe how something changes over time or space. In word problems, you’ll often be asked to find the rate of change, given certain information. Here are 7 ways to solve rate of change word problems:
Understanding Rate of Change
Rate of change is a measure of how something changes over time or space. It’s often represented mathematically as a ratio of the change in one quantity to the change in another. In word problems, you’ll often be given information about the change in one quantity and asked to find the rate of change.
Method 1: Using the Formula
The formula for rate of change is:
Rate of Change = (Change in Quantity) / (Change in Time)
This formula can be applied to a wide range of word problems. For example:
“Tom’s height increases by 5 inches over the course of 2 years. What is his rate of change in height per year?”
To solve this problem, you would use the formula:
Rate of Change = (Change in Height) / (Change in Time) = 5 inches / 2 years = 2.5 inches per year
📝 Note: Make sure to label your units correctly when solving rate of change problems.
Method 2: Using Tables and Graphs
Sometimes, you’ll be given a table or graph that shows the relationship between two quantities. You can use this information to find the rate of change.
For example:

| Time (minutes) | Distance (miles) |
|---|---|
| 0 | 0 |
| 10 | 5 |
| 20 | 10 |
| 30 | 15 |
To find the rate of change, you can calculate the change in distance over the change in time:
Rate of Change = (Change in Distance) / (Change in Time) = (15 miles - 0 miles) / (30 minutes - 0 minutes) = 15 miles / 30 minutes = 0.5 miles per minute
Method 3: Using Proportional Relationships
If you know that two quantities are proportional, you can use this relationship to find the rate of change.
For example:
“The cost of renting a car is proportional to the number of days rented. If it costs $40 to rent a car for 2 days, how much will it cost to rent a car for 5 days?”
To solve this problem, you can set up a proportion:
$40 / 2 days = x / 5 days
Cross-multiplying and solving for x, you get:
x = $100
To find the rate of change, you can divide the cost by the number of days:
Rate of Change = 100 / 5 days = 20 per day
Method 4: Using Slope-Intercept Form
If you’re given a linear equation in slope-intercept form (y = mx + b), you can use the slope (m) to find the rate of change.
For example:
“The equation y = 2x + 1 represents the relationship between the number of hours worked (x) and the amount earned (y). What is the rate of change in amount earned per hour worked?”
To solve this problem, you can use the slope (m) to find the rate of change:
Rate of Change = m = 2
This means that for every hour worked, the amount earned increases by $2.
Method 5: Using Real-World Applications
Rate of change is used in many real-world applications, such as finance, physics, and engineering. You can use these applications to solve word problems.
For example:
“A company’s profit increases by 10,000 per month. If the company's initial profit was 50,000, how much will it make in 6 months?”
To solve this problem, you can use the rate of change to find the new profit:
New Profit = Initial Profit + (Rate of Change x Time) = 50,000 + (10,000 x 6) = $110,000
Method 6: Using Average Rate of Change
Sometimes, you’ll be asked to find the average rate of change over a given interval.
For example:
“A car travels from City A to City B in 4 hours, covering a distance of 240 miles. What is the average rate of change in distance per hour?”
To solve this problem, you can use the formula:
Average Rate of Change = (Change in Distance) / (Change in Time) = 240 miles / 4 hours = 60 miles per hour
Method 7: Using Multi-Step Problems
Finally, some word problems may require you to use multiple steps to find the rate of change.
For example:
“A water tank is filled at a rate of 2 gallons per minute. If the tank is initially empty and it takes 5 hours to fill the tank, how many gallons will the tank hold?”
To solve this problem, you’ll need to use multiple steps:
- Find the total amount of time it takes to fill the tank: 5 hours x 60 minutes per hour = 300 minutes
- Find the total amount of water in the tank: 2 gallons per minute x 300 minutes = 600 gallons
The final answer is: the tank will hold 600 gallons of water.
In conclusion, rate of change is a fundamental concept in mathematics and physics, and it’s used to describe how something changes over time or space. By using these 7 methods, you can solve a wide range of rate of change word problems.
What is rate of change?
+Rate of change is a measure of how something changes over time or space. It’s often represented mathematically as a ratio of the change in one quantity to the change in another.
How do I solve rate of change word problems?
+You can solve rate of change word problems using a variety of methods, including using the formula, tables and graphs, proportional relationships, slope-intercept form, real-world applications, average rate of change, and multi-step problems.
What is the formula for rate of change?
+The formula for rate of change is: Rate of Change = (Change in Quantity) / (Change in Time)
Related Terms:
- Rate of change Worksheet PDF