5 Ways to Master Quadratic Equation Factoring
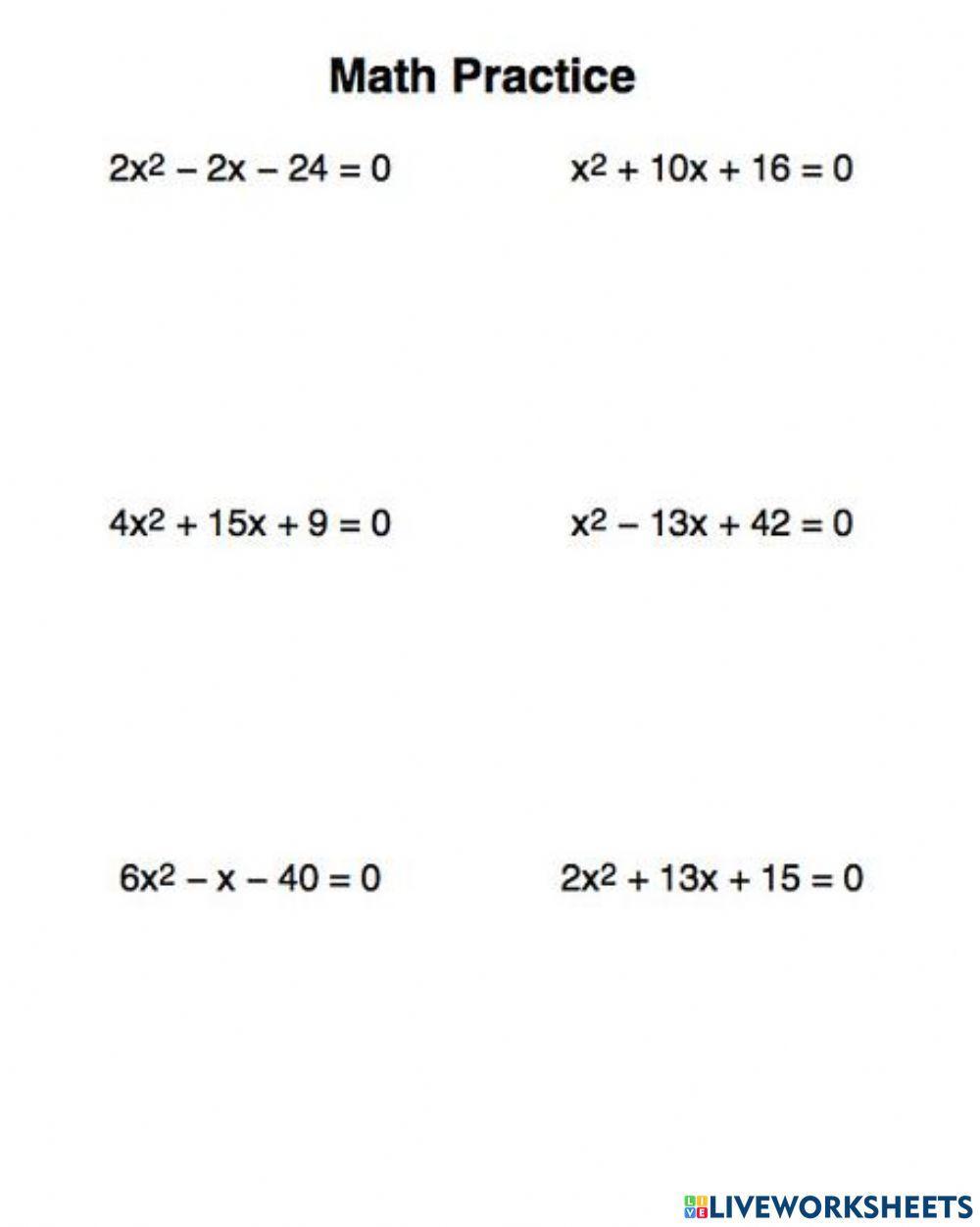
Understanding Quadratic Equations
Quadratic equations are a fundamental concept in algebra, and factoring them is an essential skill for any student or professional in mathematics. A quadratic equation is a polynomial equation of degree two, which means the highest power of the variable is two. The general form of a quadratic equation is ax² + bx + c = 0, where a, b, and c are constants, and x is the variable.
Why Factoring is Important
Factoring quadratic equations is crucial in solving them, as it allows us to find the roots or solutions of the equation. Factoring involves expressing the quadratic equation as a product of two binomials, which can then be easily solved. Mastering quadratic equation factoring can help you solve problems in physics, engineering, economics, and other fields that rely heavily on mathematical modeling.
Method 1: Factoring by Grouping
Factoring by grouping is a popular method for factoring quadratic equations. This method involves grouping the terms of the quadratic equation and then factoring out the greatest common factor (GCF). To factor by grouping, follow these steps:
- Write down the quadratic equation in the form ax² + bx + c = 0.
- Group the terms into two pairs, ax² + bx and c.
- Factor out the GCF from each pair.
- Factor out the common binomial factor from the two pairs.
For example, consider the quadratic equation x² + 5x + 6 = 0. We can factor this equation by grouping as follows:
x² + 5x + 6 = (x² + 3x) + (2x + 6) = x(x + 3) + 2(x + 3) = (x + 3)(x + 2) = 0
📝 Note: This method works best when the quadratic equation has a GCF that can be easily factored out.
Method 2: Factoring Using the AC Method
The AC method is another popular method for factoring quadratic equations. This method involves finding two numbers whose product is ac and whose sum is b. To factor using the AC method, follow these steps:
- Write down the quadratic equation in the form ax² + bx + c = 0.
- Find two numbers whose product is ac and whose sum is b.
- Write these two numbers as the coefficients of the two binomials.
- Factor out the common binomial factor from the two binomials.
For example, consider the quadratic equation x² + 7x + 12 = 0. We can factor this equation using the AC method as follows:
x² + 7x + 12 = x² + 3x + 4x + 12 = x(x + 3) + 4(x + 3) = (x + 3)(x + 4) = 0
📝 Note: This method works best when the quadratic equation has a product ac that can be easily factored into two numbers whose sum is b.
Method 3: Factoring Using the Perfect Square Trinomial Method
The perfect square trinomial method is a special case of factoring quadratic equations. This method involves recognizing that the quadratic equation is a perfect square trinomial, which can be factored into the square of a binomial. To factor using the perfect square trinomial method, follow these steps:
- Write down the quadratic equation in the form ax² + bx + c = 0.
- Check if the quadratic equation is a perfect square trinomial by checking if b² = 4ac.
- If b² = 4ac, then the quadratic equation can be factored into the square of a binomial.
For example, consider the quadratic equation x² + 6x + 9 = 0. We can factor this equation using the perfect square trinomial method as follows:
x² + 6x + 9 = (x + 3)² = 0
📝 Note: This method works best when the quadratic equation is a perfect square trinomial.
Method 4: Factoring Using the Quadratic Formula
The quadratic formula is a general method for solving quadratic equations. While it is not a factoring method per se, it can be used to factor quadratic equations by finding the roots of the equation. To factor using the quadratic formula, follow these steps:
- Write down the quadratic equation in the form ax² + bx + c = 0.
- Use the quadratic formula x = (-b ± √(b² - 4ac)) / 2a to find the roots of the equation.
- Write the roots as the factors of the quadratic equation.
For example, consider the quadratic equation x² + 5x + 6 = 0. We can factor this equation using the quadratic formula as follows:
x = (-5 ± √(5² - 4(1)(6))) / 2(1) = (-5 ± √(25 - 24)) / 2 = (-5 ± √1) / 2 = (-5 ± 1) / 2
x = (-5 + 1) / 2 or x = (-5 - 1) / 2 x = -2 or x = -3
x² + 5x + 6 = (x + 2)(x + 3) = 0
📝 Note: This method works best when the quadratic equation has a discriminant b² - 4ac that is a perfect square.
Method 5: Factoring Using the Completed Square Method
The completed square method is another method for factoring quadratic equations. This method involves completing the square by adding and subtracting a constant term to make the quadratic equation a perfect square trinomial. To factor using the completed square method, follow these steps:
- Write down the quadratic equation in the form ax² + bx + c = 0.
- Complete the square by adding and subtracting a constant term (b/2)².
- Factor the resulting perfect square trinomial.
For example, consider the quadratic equation x² + 6x + 8 = 0. We can factor this equation using the completed square method as follows:
x² + 6x + 8 = x² + 6x + 9 - 1 = (x + 3)² - 1 = 0
x + 3 = ±√1 x + 3 = ±1
x = -3 ± 1 x = -2 or x = -4
x² + 6x + 8 = (x + 2)(x + 4) = 0
📝 Note: This method works best when the quadratic equation has a discriminant b² - 4ac that is not a perfect square.
In conclusion, mastering quadratic equation factoring requires practice and patience. By using the methods outlined above, you can develop a strong foundation in factoring quadratic equations and improve your problem-solving skills in mathematics and other fields.
What is the difference between factoring and solving a quadratic equation?
+Factoring a quadratic equation involves expressing it as a product of two binomials, while solving a quadratic equation involves finding the roots or solutions of the equation.
Can all quadratic equations be factored?
+No, not all quadratic equations can be factored. Some quadratic equations may have complex roots or require numerical methods to solve.
What is the quadratic formula?
+The quadratic formula is a general method for solving quadratic equations of the form ax² + bx + c = 0. It is given by x = (-b ± √(b² - 4ac)) / 2a.