5 Ways to Calculate Distance with Pythagorean Theorem

Introduction to Pythagorean Theorem

The Pythagorean Theorem is a fundamental concept in geometry that has been widely used for centuries to calculate distances in various fields, including physics, engineering, architecture, and more. This theorem is named after the ancient Greek philosopher and mathematician Pythagoras, who is credited with its discovery. In this article, we will explore five ways to calculate distance using the Pythagorean Theorem.

What is the Pythagorean Theorem?

The Pythagorean Theorem is a mathematical concept that describes the relationship between the lengths of the sides of a right-angled triangle. The theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. Mathematically, this can be expressed as:

a² + b² = c²

where a and b are the lengths of the two sides, and c is the length of the hypotenuse.

Method 1: Calculating Distance in a Right-Angled Triangle

The most straightforward way to use the Pythagorean Theorem is to calculate the distance in a right-angled triangle. Suppose we have a triangle with two sides of lengths 3 cm and 4 cm, and we want to calculate the length of the hypotenuse.

Using the Pythagorean Theorem, we can calculate the length of the hypotenuse as follows:

c² = a² + b² c² = 3² + 4² c² = 9 + 16 c² = 25 c = √25 c = 5 cm

Therefore, the length of the hypotenuse is 5 cm.

📝 Note: When using the Pythagorean Theorem, it's essential to ensure that the triangle is right-angled. If the triangle is not right-angled, the theorem will not give accurate results.

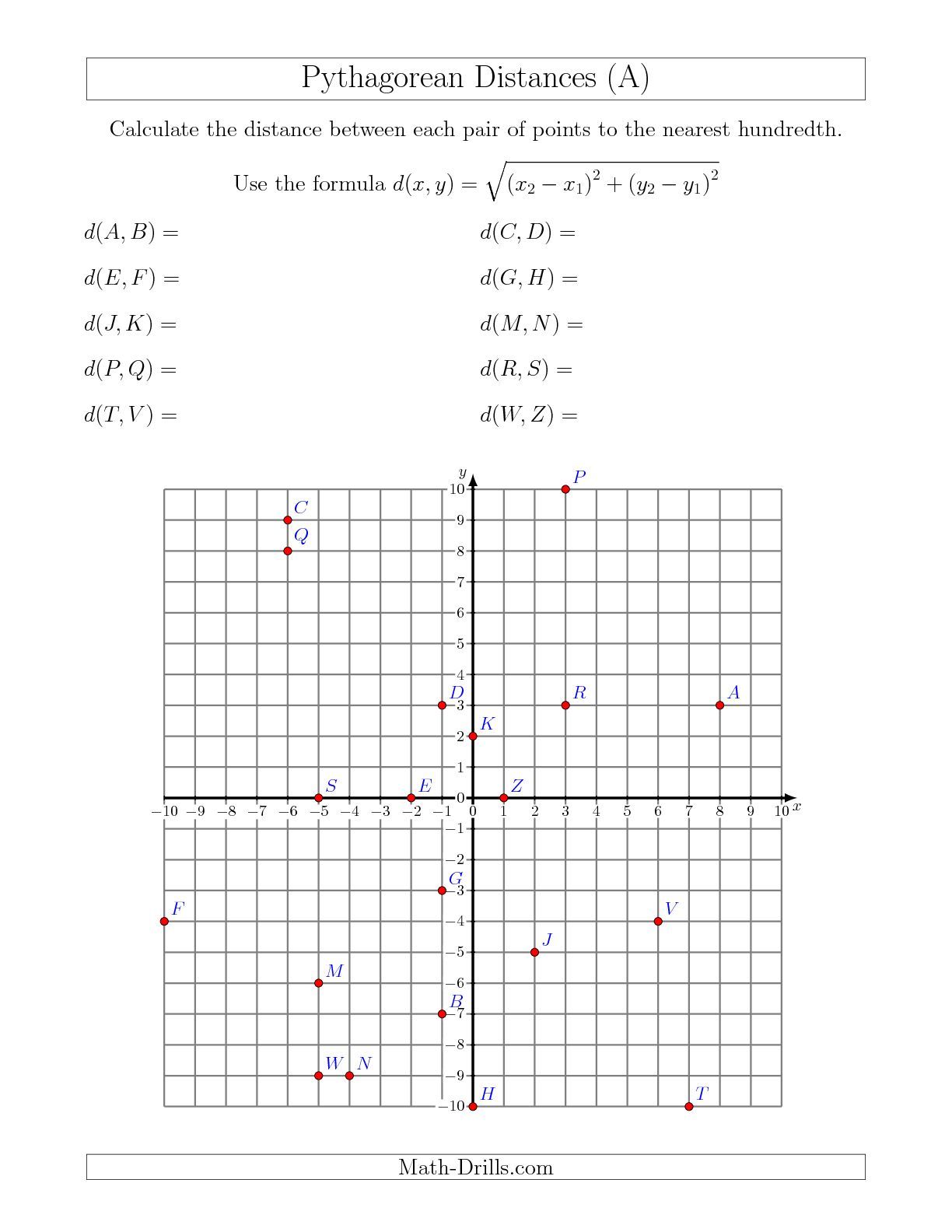
Method 2: Calculating Distance in a Coordinate Plane

Another way to use the Pythagorean Theorem is to calculate the distance between two points in a coordinate plane. Suppose we have two points, A (2, 3) and B (6, 8), and we want to calculate the distance between them.

Using the Pythagorean Theorem, we can calculate the distance as follows:

distance² = (x₂ - x₁)² + (y₂ - y₁)² distance² = (6 - 2)² + (8 - 3)² distance² = 4² + 5² distance² = 16 + 25 distance² = 41 distance = √41 distance ≈ 6.4

Therefore, the distance between points A and B is approximately 6.4 units.

Method 3: Calculating Distance in 3D Space

The Pythagorean Theorem can also be used to calculate distances in 3D space. Suppose we have two points, A (1, 2, 3) and B (4, 5, 6), and we want to calculate the distance between them.

Using the Pythagorean Theorem, we can calculate the distance as follows:

distance² = (x₂ - x₁)² + (y₂ - y₁)² + (z₂ - z₁)² distance² = (4 - 1)² + (5 - 2)² + (6 - 3)² distance² = 3² + 3² + 3² distance² = 9 + 9 + 9 distance² = 27 distance = √27 distance ≈ 5.2

Therefore, the distance between points A and B is approximately 5.2 units.

Method 4: Calculating Distance in a Rectangle

The Pythagorean Theorem can also be used to calculate the distance between two points in a rectangle. Suppose we have a rectangle with a length of 8 cm and a width of 5 cm, and we want to calculate the distance between two points, A and B, located at opposite corners of the rectangle.

Using the Pythagorean Theorem, we can calculate the distance as follows:

distance² = length² + width² distance² = 8² + 5² distance² = 64 + 25 distance² = 89 distance = √89 distance ≈ 9.4

Therefore, the distance between points A and B is approximately 9.4 cm.

Method 5: Calculating Distance in a Circle

Finally, the Pythagorean Theorem can be used to calculate the distance between two points on a circle. Suppose we have a circle with a radius of 4 cm, and we want to calculate the distance between two points, A and B, located on the circle.

Using the Pythagorean Theorem, we can calculate the distance as follows:

distance² = radius² + radius² distance² = 4² + 4² distance² = 16 + 16 distance² = 32 distance = √32 distance ≈ 5.7

Therefore, the distance between points A and B is approximately 5.7 cm.

In conclusion, the Pythagorean Theorem is a powerful tool for calculating distances in various fields, including geometry, physics, engineering, and more. By applying the theorem in different ways, we can calculate distances in right-angled triangles, coordinate planes, 3D space, rectangles, and circles.