Prove Lines Are Parallel Worksheet
Proving Lines Are Parallel: A Comprehensive Guide
Proving lines are parallel is an essential concept in geometry, and it’s crucial to understand the various methods to determine if two lines are parallel. In this article, we’ll delve into the world of parallel lines, explore the different techniques to prove they are parallel, and provide a worksheet to help you practice.
What Are Parallel Lines?
Parallel lines are two or more lines that lie in the same plane and never intersect, no matter how far they are extended. In other words, parallel lines have the same slope and never touch each other.
Methods to Prove Lines Are Parallel
There are several methods to prove that two lines are parallel. Here are some of the most common techniques:
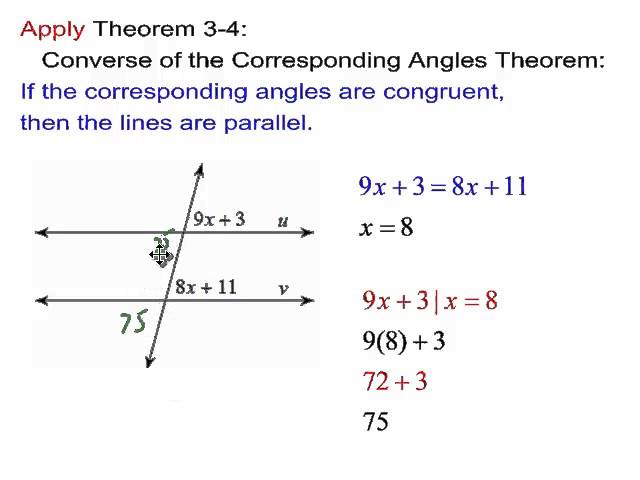
1. Corresponding Angles
If two lines are cut by a transversal, and the corresponding angles are congruent, then the lines are parallel.

| Angle | Corresponding Angle |
|---|---|
| ∠A | ∠D |
| ∠B | ∠E |
| ∠C | ∠F |
2. Alternate Interior Angles
If two lines are cut by a transversal, and the alternate interior angles are congruent, then the lines are parallel.
| Angle | Alternate Interior Angle |
|---|---|
| ∠A | ∠E |
| ∠B | ∠F |
| ∠C | ∠D |
3. Same Side Interior Angles
If two lines are cut by a transversal, and the same side interior angles are supplementary (add up to 180°), then the lines are parallel.
| Angle | Same Side Interior Angle |
|---|---|
| ∠A | ∠B |
| ∠C | ∠D |
| ∠E | ∠F |
4. Slope
If two lines have the same slope, then they are parallel.
Proving Lines Are Parallel Worksheet
Directions: Determine if the lines are parallel or not parallel. If they are parallel, state the reason.
1. Two lines are cut by a transversal. The corresponding angles are ∠A = 60° and ∠D = 60°. Are the lines parallel?
Answer: Yes, the lines are parallel because the corresponding angles are congruent.
2. Two lines are cut by a transversal. The alternate interior angles are ∠A = 30° and ∠E = 30°. Are the lines parallel?
Answer: Yes, the lines are parallel because the alternate interior angles are congruent.
3. Two lines have slopes m1 = 2⁄3 and m2 = 2⁄3. Are the lines parallel?
Answer: Yes, the lines are parallel because they have the same slope.
4. Two lines are cut by a transversal. The same side interior angles are ∠A = 120° and ∠B = 60°. Are the lines parallel?
Answer: Yes, the lines are parallel because the same side interior angles are supplementary.
💡 Note: The transversal can be any line that intersects the two lines.
📝 Note: You can use the slope formula (m = y2 - y1 / x2 - x1) to find the slope of the lines.
Now that you’ve learned the different methods to prove lines are parallel, try the following exercises:
Exercises:
1. Two lines are cut by a transversal. The corresponding angles are ∠A = 45° and ∠D = 45°. Are the lines parallel?
2. Two lines are cut by a transversal. The alternate interior angles are ∠A = 60° and ∠E = 60°. Are the lines parallel?
3. Two lines have slopes m1 = 1⁄2 and m2 = 1⁄2. Are the lines parallel?
4. Two lines are cut by a transversal. The same side interior angles are ∠A = 90° and ∠B = 90°. Are the lines parallel?
What is the difference between corresponding angles and alternate interior angles?
+Corresponding angles are angles that are in the same relative position, while alternate interior angles are angles that are on opposite sides of the transversal and inside the two lines.
Can two lines be parallel if they have different slopes?
+No, two lines cannot be parallel if they have different slopes. Parallel lines must have the same slope.
What is the purpose of the transversal in proving lines are parallel?
+The transversal is used to create corresponding angles, alternate interior angles, and same side interior angles, which can be used to prove that the lines are parallel.
In conclusion, proving lines are parallel is a fundamental concept in geometry, and there are several methods to determine if two lines are parallel. By understanding the different techniques, you’ll be able to identify parallel lines and apply this knowledge to various mathematical problems.
Related Terms:
- Proving lines parallel Worksheet PDF
- Which lines are parallel Worksheet