5 Ways to Master 7th Grade Proportional Relationships
Unlocking the Secrets of Proportional Relationships in 7th Grade
As a 7th grader, you’re probably familiar with the concept of proportional relationships. But, do you know how to master them? Proportional relationships are a fundamental concept in mathematics, and understanding them is crucial for solving various problems in algebra, geometry, and even real-life scenarios. In this article, we’ll explore five ways to help you master 7th grade proportional relationships.
1. Understanding the Basics of Proportional Relationships
Before we dive into the strategies, let’s review the basics. A proportional relationship is a relationship between two quantities that change at a constant rate. This means that as one quantity increases or decreases, the other quantity changes in a predictable way. For example, if you have 2 cookies and your friend has 4 cookies, the ratio of your cookies to your friend’s cookies is 1:2. If you both get 2 more cookies, the ratio remains the same.
To represent proportional relationships, we use the following notation:
y = kx
where y is the dependent variable, x is the independent variable, and k is the constant of proportionality.
📝 Note: The constant of proportionality (k) is a crucial part of proportional relationships. It helps you understand the rate at which the quantities change.
2. Visualizing Proportional Relationships with Graphs
Graphs are an excellent way to visualize proportional relationships. A graph of a proportional relationship is a straight line that passes through the origin (0, 0). The constant of proportionality (k) is the slope of the line.
For example, let’s say you have a proportional relationship between the number of hours you work (x) and the amount of money you earn (y). If you earn $10 per hour, the graph would be a straight line with a slope of 10.
| x (hours) | y (dollars) |
|---|---|
| 1 | 10 |
| 2 | 20 |
| 3 | 30 |
As you can see, the graph shows a clear proportional relationship between the number of hours worked and the amount of money earned.
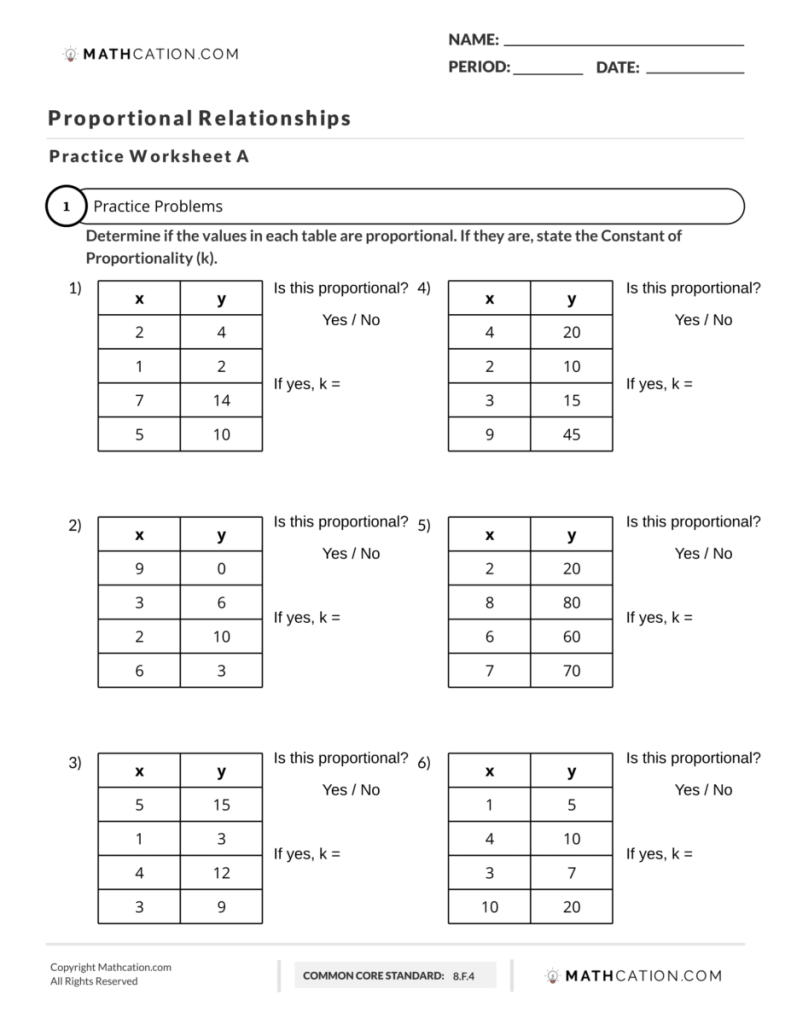
3. Using Tables to Represent Proportional Relationships
Tables are another effective way to represent proportional relationships. By creating a table with the input and output values, you can easily identify the constant of proportionality.
For example, let’s say you have a proportional relationship between the number of pencils (x) and the number of erasers (y). If you have 2 pencils for every 3 erasers, the table would look like this:
| x (pencils) | y (erasers) |
|---|---|
| 2 | 3 |
| 4 | 6 |
| 6 | 9 |
By analyzing the table, you can see that the constant of proportionality (k) is 3⁄2.
4. Solving Proportional Relationship Problems with Equations
Now that you’ve learned how to represent proportional relationships with graphs and tables, it’s time to solve problems using equations. To solve proportional relationship problems, you can use the following steps:
- Write the equation in the form y = kx
- Plug in the given values for x and y
- Solve for k, the constant of proportionality
- Use the value of k to find the missing value
For example, let’s say you have a proportional relationship between the number of books (x) and the number of pages (y). If you have 5 books with 120 pages each, and you want to know how many pages 8 books would have, you can use the following equation:
y = kx
Plug in the given values:
120 = k(5)
Solve for k:
k = 120⁄5 k = 24
Now, use the value of k to find the missing value:
y = 24(8) y = 192
Therefore, 8 books would have 192 pages.
📝 Note: Remember to always plug in the given values and solve for k before finding the missing value.
5. Real-World Applications of Proportional Relationships
Proportional relationships are all around us, and understanding them can help you make sense of real-world problems. For example, if you’re planning a road trip, you can use proportional relationships to calculate the distance, time, and fuel consumption.
Let’s say you’re driving from City A to City B, and you want to know how much fuel you’ll need for the trip. If you know that your car consumes 25 miles per gallon, and you have a 15-gallon tank, you can use proportional relationships to calculate the distance you can travel:
Distance = Rate x Time Distance = 25 miles/gallon x 15 gallons Distance = 375 miles
By understanding proportional relationships, you can make informed decisions in various aspects of life, from finance to science.
In conclusion, mastering 7th grade proportional relationships requires a combination of understanding the basics, visualizing relationships with graphs and tables, solving problems with equations, and applying them to real-world scenarios. By following these five strategies, you’ll be well on your way to becoming a proportional relationship pro!
What is the difference between a proportional relationship and a non-proportional relationship?
+A proportional relationship is a relationship between two quantities that change at a constant rate, whereas a non-proportional relationship is a relationship between two quantities that do not change at a constant rate.
How do I identify a proportional relationship in a graph?
+A graph of a proportional relationship is a straight line that passes through the origin (0, 0). The constant of proportionality (k) is the slope of the line.
Can proportional relationships be used to model real-world problems?
+Related Terms:
- teacher synergy llc
- Khan Academy
- IXL
- BrainPOP
- Udacity
- Duolingo