Mastering Point Slope Form Made Easy

Unlocking the Secrets of Point Slope Form

When it comes to linear equations, point slope form is an essential concept that every student and math enthusiast should master. It’s a powerful tool that helps you find the equation of a line given a point and the slope. In this article, we’ll delve into the world of point slope form, exploring its definition, formula, and applications. We’ll also provide step-by-step examples and tips to help you become proficient in using this form.

What is Point Slope Form?

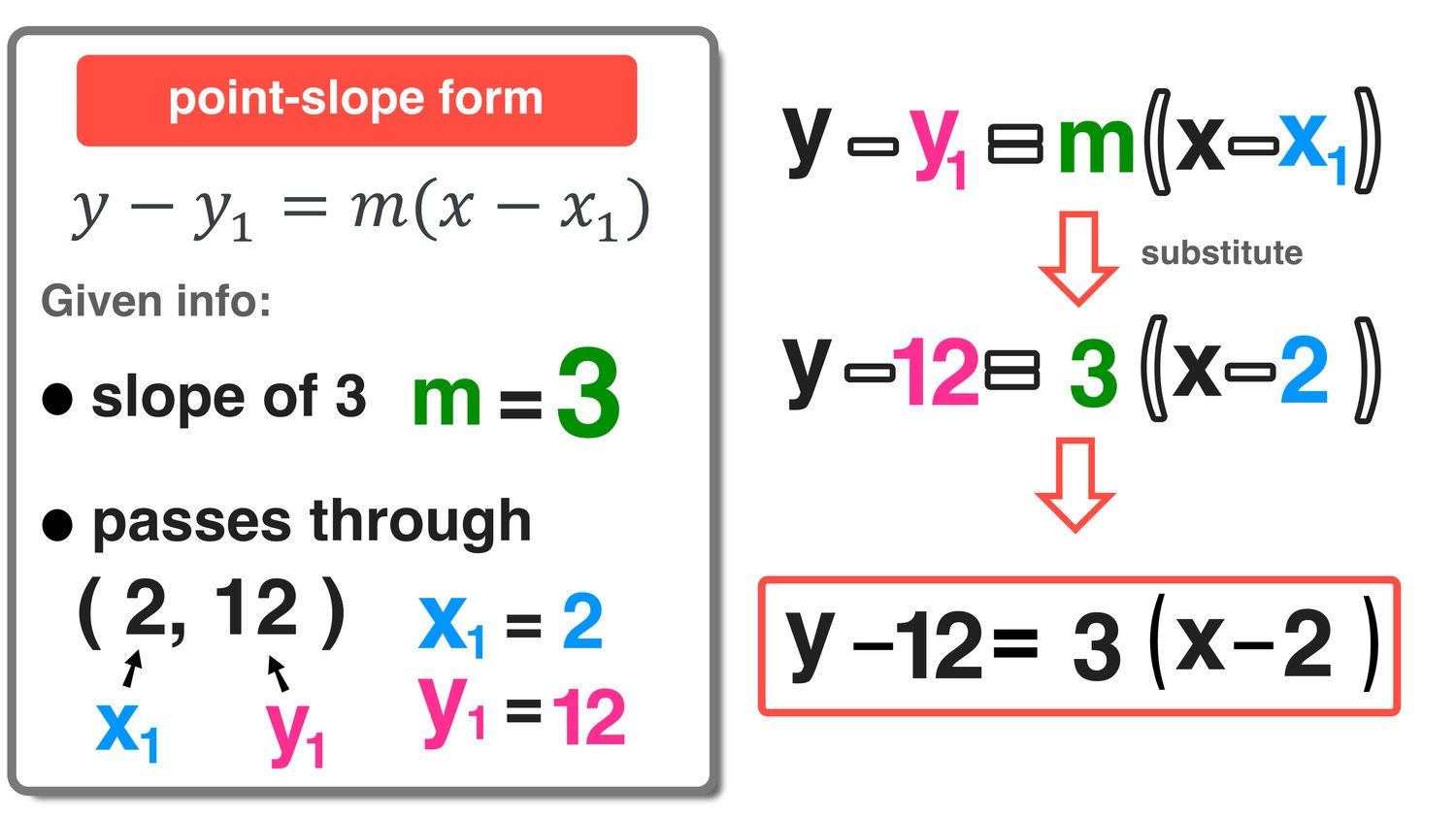
Point slope form is a way of expressing a linear equation in the form y - y1 = m(x - x1), where:

• m is the slope of the line
• (x1, y1) is a point on the line
• x and y are the variables

This form is particularly useful when you’re given a point and the slope, and you need to find the equation of the line.

The Formula: Breaking it Down

The point slope formula is:

y - y1 = m(x - x1)

Let’s dissect this formula:

• y - y1 represents the change in y-coordinates between two points on the line.
• m(x - x1) represents the change in x-coordinates between two points on the line, multiplied by the slope.
• The slope, m, tells you how steep the line is. A positive slope indicates a line that slopes upward from left to right, while a negative slope indicates a line that slopes downward from left to right.

Examples: Putting Point Slope Form into Practice

Now that we’ve covered the basics, let’s dive into some examples to illustrate how point slope form works.

Example 1: Find the equation of the line that passes through the point (2, 3) with a slope of 4.

Using the point slope formula, we get:

y - 3 = 4(x - 2)

Simplifying this equation, we get:

y = 4x - 5

Example 2: Find the equation of the line that passes through the point (-1, 2) with a slope of -3.

Using the point slope formula, we get:

y - 2 = -3(x + 1)

Simplifying this equation, we get:

y = -3x - 1

Applications of Point Slope Form

Point slope form has numerous applications in various fields, including:

• Physics: Point slope form is used to model the motion of objects, where the slope represents the velocity and the point represents the initial position.
• Economics: Point slope form is used to model supply and demand curves, where the slope represents the rate of change of the curve.
• Computer Science: Point slope form is used in computer graphics to render lines and curves.

Tips and Tricks for Mastering Point Slope Form

Here are some tips to help you become proficient in using point slope form:

• Practice, practice, practice: The more you practice, the more comfortable you’ll become with using point slope form.
• Pay attention to the signs: Make sure to pay attention to the signs of the slope and the point, as this can affect the direction of the line.
• Use graphing tools: Graphing tools like graphing calculators or online graphing software can help you visualize the line and check your work.

🤔 Note: When using point slope form, make sure to label the point and slope clearly, and double-check your work to avoid mistakes.

Now that we’ve covered the basics of point slope form, you’re ready to tackle more complex problems and applications. Remember to practice regularly and use graphing tools to help you visualize the lines. With time and practice, you’ll become a master of point slope form!