Permutations vs Combinations Worksheet: Math Concepts Made Easy

Understanding Permutations and Combinations: A Comprehensive Guide

Permutations and combinations are two fundamental concepts in mathematics that are used to calculate the number of ways to arrange or select items from a set. While they may seem similar, there are key differences between the two. In this article, we will delve into the world of permutations and combinations, explore their definitions, formulas, and examples, and provide a worksheet to help you practice and reinforce your understanding.

What are Permutations?

A permutation is an arrangement of objects in a specific order. The order of the objects matters, and each object can only be used once. For example, if you have three letters - A, B, and C - and you want to arrange them in all possible orders, the permutations would be:

ABC, ACB, BAC, BCA, CAB, CBA

There are 6 permutations in this case, as there are 3! (3 factorial) ways to arrange the letters.

Permutation Formula

The formula for permutations is:

n! / (n-r)!

Where:

• n is the total number of objects
• r is the number of objects being chosen *! denotes the factorial function (e.g., 5! = 5 × 4 × 3 × 2 × 1)

For example, if you have 5 letters - A, B, C, D, and E - and you want to arrange 3 of them in all possible orders, the formula would be:

5! / (5-3)! = 5! / 2! = (5 × 4 × 3 × 2 × 1) / (2 × 1) = 120 / 2 = 60

There are 60 permutations in this case.

What are Combinations?

A combination is a selection of objects from a set, where the order does not matter. Each object can only be used once. For example, if you have three letters - A, B, and C - and you want to select two of them, the combinations would be:

AB, AC, BC

There are 3 combinations in this case, as there are 3 choose 2 ways to select the letters.

Combination Formula

The formula for combinations is:

n! / (r!(n-r)!)

Where:

• n is the total number of objects
• r is the number of objects being chosen *! denotes the factorial function (e.g., 5! = 5 × 4 × 3 × 2 × 1)

For example, if you have 5 letters - A, B, C, D, and E - and you want to select 3 of them, the formula would be:

5! / (3!(5-3)!) = 5! / (3!2!) = (5 × 4 × 3 × 2 × 1) / ((3 × 2 × 1)(2 × 1)) = 120 / (6 × 2) = 120 / 12 = 10

There are 10 combinations in this case.

Key Differences between Permutations and Combinations

The key differences between permutations and combinations are:

• Order matters in permutations: The order of the objects matters in permutations, whereas it does not matter in combinations.
• Repetition is not allowed: In both permutations and combinations, each object can only be used once.
• Different formulas: The formulas for permutations and combinations are different, reflecting the different ways in which objects are arranged or selected.

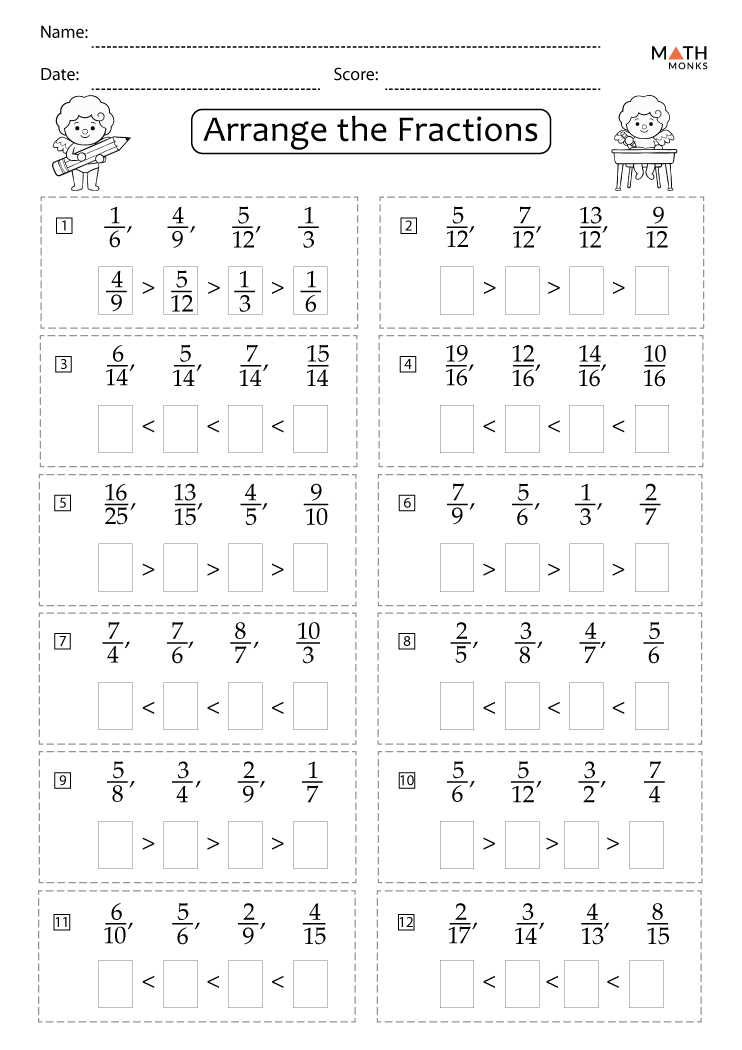
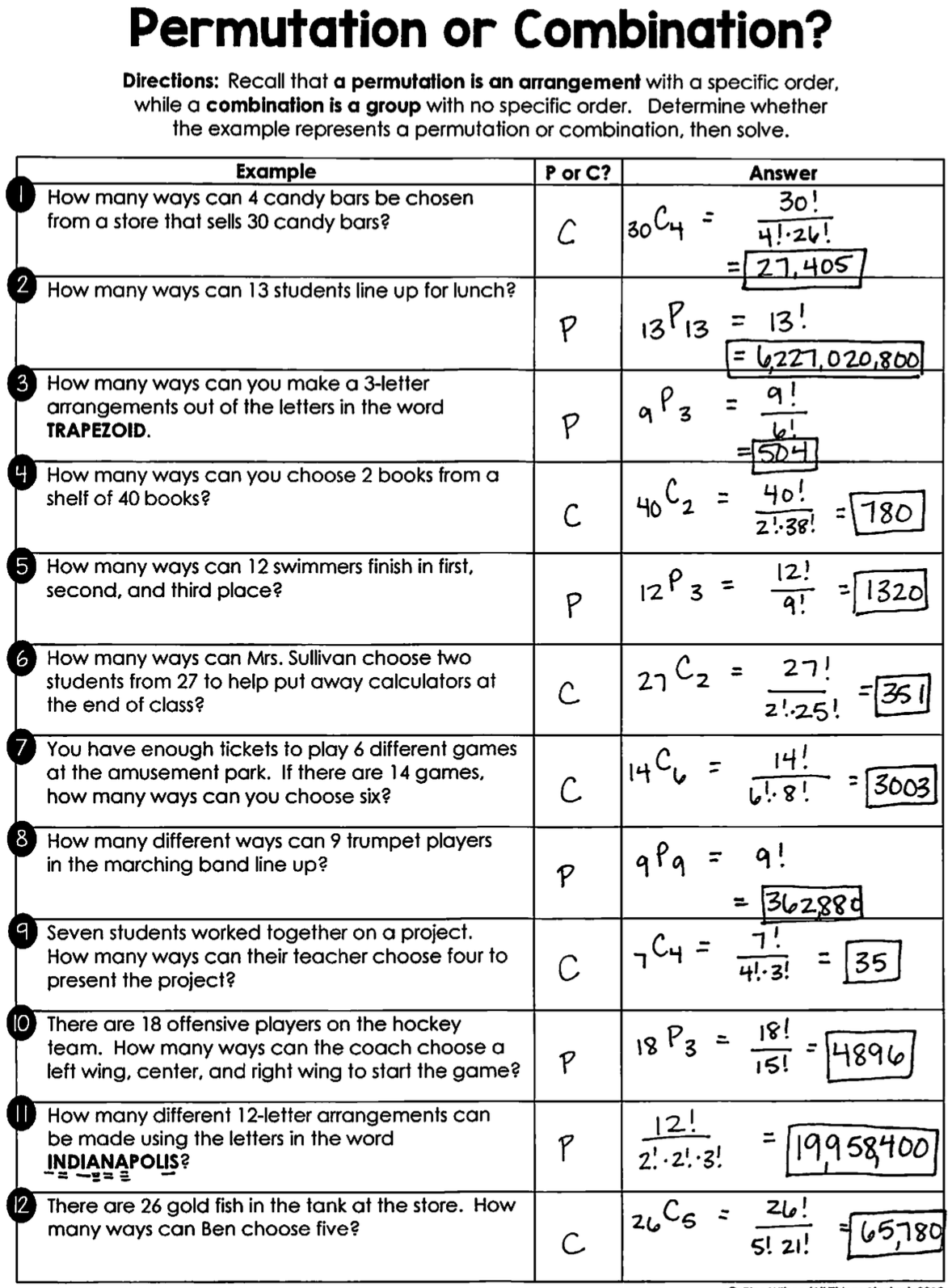
Worksheet: Permutations and Combinations Practice

Now that you have a good understanding of permutations and combinations, it’s time to practice! Here are some questions to help you reinforce your understanding:

Permutations

1. How many permutations are there of the letters A, B, and C?
2. If you have 5 letters - A, B, C, D, and E - and you want to arrange 3 of them in all possible orders, how many permutations are there?
3. A bookshelf has 5 books on it. How many permutations are there if you want to arrange all 5 books in different orders?

Combinations

1. How many combinations are there of the letters A, B, and C if you want to select 2 of them?
2. If you have 5 letters - A, B, C, D, and E - and you want to select 3 of them, how many combinations are there?
3. A box of chocolates has 10 chocolates in it. How many combinations are there if you want to select 4 chocolates?

📝 Note: Take your time to work through the worksheet and check your answers. You can use the formulas and examples provided earlier to help you.

Final Thoughts

Permutations and combinations are fundamental concepts in mathematics that are used to calculate the number of ways to arrange or select items from a set. By understanding the definitions, formulas, and examples provided in this article, you should now have a solid grasp of these concepts. Remember to practice regularly to reinforce your understanding and become more confident in your ability to work with permutations and combinations.

Related Terms:

• Permutations vs Combinations Worksheet answers
• Permutation and combination Worksheet pdf
• Permutations Worksheet with answers pdf
• Permutations and Combinations Quiz PDF