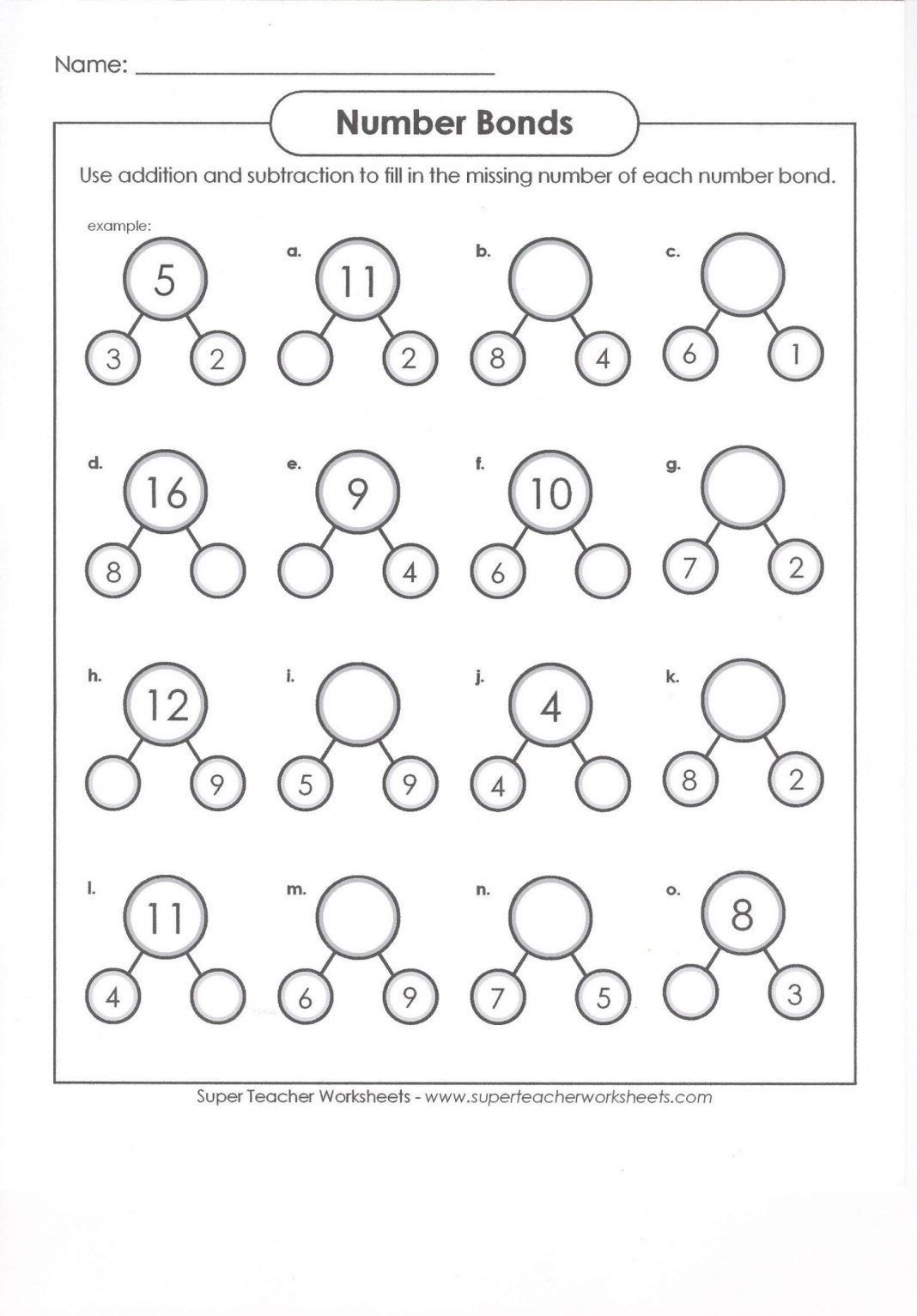
Partial Product Multiplication Worksheet for Math Mastery
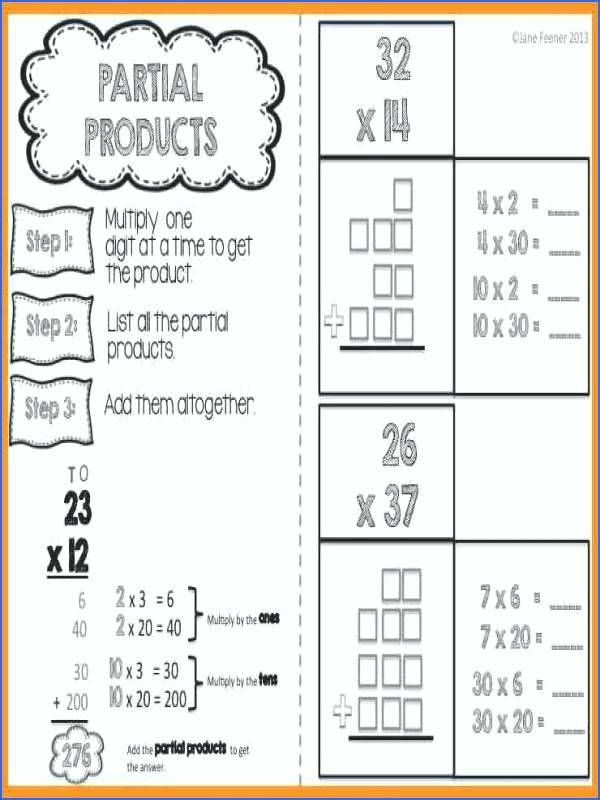
Mastering Partial Product Multiplication: A Comprehensive Guide
Partial product multiplication is a powerful strategy used to multiply multi-digit numbers. It involves breaking down the multiplication problem into smaller parts, making it easier to calculate and understand the process. In this article, we will delve into the world of partial product multiplication, exploring its benefits, step-by-step instructions, and providing a worksheet for practice.
What is Partial Product Multiplication?
Partial product multiplication is a method of multiplying numbers by breaking them down into smaller parts. This approach helps to reduce the complexity of the multiplication problem, making it more manageable and easier to understand. The partial products are then added together to obtain the final result.
Benefits of Partial Product Multiplication
The partial product multiplication strategy offers several benefits, including:
- Improved understanding: By breaking down the multiplication problem into smaller parts, students can better understand the process and develop a deeper understanding of place value.
- Increased accuracy: Partial product multiplication reduces the likelihood of errors, as students are working with smaller numbers and can easily check their calculations.
- Enhanced flexibility: This strategy can be used to multiply numbers of varying lengths, making it a versatile tool for math students.
Step-by-Step Instructions for Partial Product Multiplication
To use the partial product multiplication strategy, follow these steps:
- Write the multiplication problem: Write the multiplication problem in the standard format, with the multiplicand (the number being multiplied) and the multiplier (the number by which we are multiplying).
- Break down the multiplicand: Break down the multiplicand into smaller parts, usually tens and ones.
- Multiply each part: Multiply each part of the multiplicand by the multiplier.
- Calculate the partial products: Calculate the partial products by multiplying each part of the multiplicand by the multiplier.
- Add the partial products: Add the partial products together to obtain the final result.
📝 Note: It's essential to line up the numbers correctly and use the correct place value when adding the partial products.
Partial Product Multiplication Worksheet
Practice your partial product multiplication skills with the following worksheet:
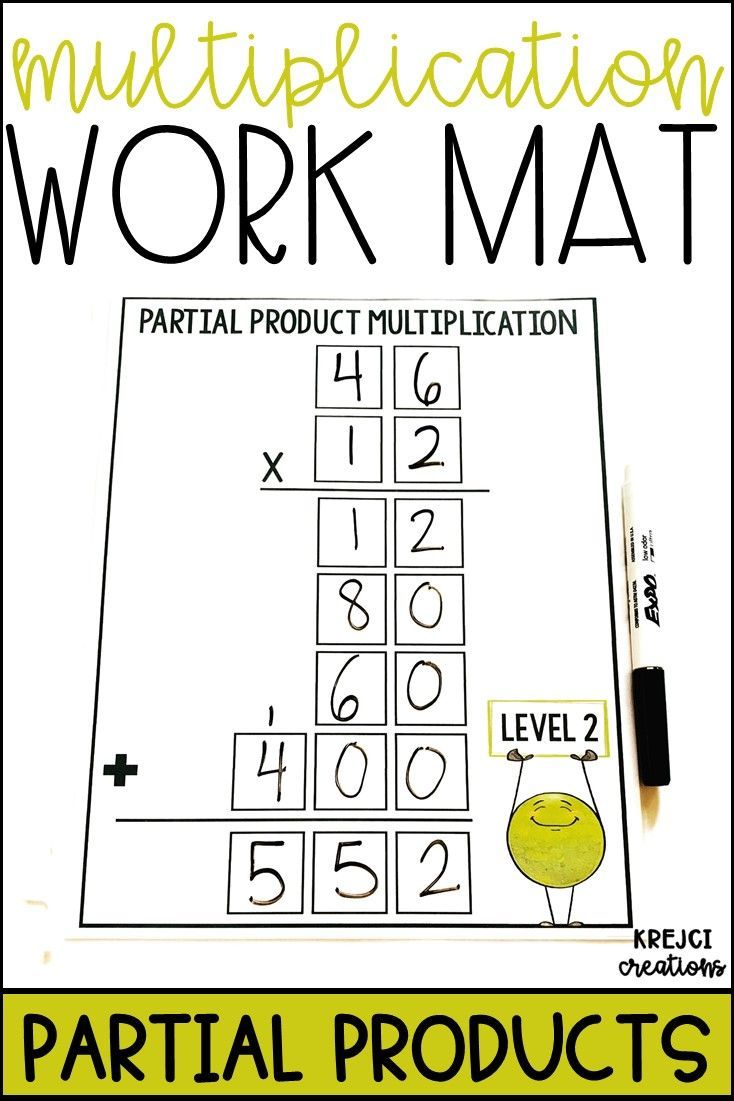
| Multiplication Problem | Partial Products | Final Answer |
|---|---|---|
| 43 × 27 | 40 × 27 = 1080, 3 × 27 = 81 | 1161 |
| 95 × 43 | 90 × 43 = 3870, 5 × 43 = 215 | 4085 |
| 76 × 92 | 70 × 92 = 6440, 6 × 92 = 552 | 6992 |
| 34 × 56 | 30 × 56 = 1680, 4 × 56 = 224 | 1904 |
| 98 × 75 | 90 × 75 = 6750, 8 × 75 = 600 | 7350 |
Conclusion
Mastering partial product multiplication is an essential skill for math students. By breaking down the multiplication problem into smaller parts, students can develop a deeper understanding of place value and improve their accuracy. With practice and patience, students can become proficient in using this strategy to solve multiplication problems. Remember to line up the numbers correctly and use the correct place value when adding the partial products.
What is the main benefit of using partial product multiplication?
+The main benefit of using partial product multiplication is that it helps to reduce the complexity of the multiplication problem, making it easier to understand and calculate.
How do I calculate the partial products?
+To calculate the partial products, multiply each part of the multiplicand by the multiplier. For example, if the multiplicand is 43 and the multiplier is 27, the partial products would be 40 × 27 = 1080 and 3 × 27 = 81.
What is the importance of lining up the numbers correctly when adding the partial products?
+Lining up the numbers correctly is essential when adding the partial products to ensure that the place value is correct. This helps to avoid errors and ensures that the final answer is accurate.