Simplifying Complex Numbers: Operations Worksheet Answers
Simplifying Complex Numbers: A Comprehensive Guide
Complex numbers are a fundamental concept in mathematics, particularly in algebra and calculus. They consist of a real part and an imaginary part, which can be represented as a+bi, where ‘a’ is the real part and ‘b’ is the imaginary part. In this article, we will delve into the world of complex numbers and provide a comprehensive guide on how to simplify them, along with a worksheet with answers.
What are Complex Numbers?
Complex numbers are numbers that have both a real and an imaginary part. They are represented in the form a+bi, where ‘a’ is the real part and ‘b’ is the imaginary part. The imaginary part is denoted by the symbol ‘i’, which is defined as the square root of -1.
Types of Complex Numbers
There are several types of complex numbers, including:
- Real Numbers: These are complex numbers with an imaginary part of 0. For example, 5+0i is a real number.
- Imaginary Numbers: These are complex numbers with a real part of 0. For example, 0+5i is an imaginary number.
- Purely Imaginary Numbers: These are complex numbers with a real part of 0 and a non-zero imaginary part. For example, 0+5i is a purely imaginary number.
- Complex Numbers with Non-Zero Real and Imaginary Parts: These are complex numbers with both a real and an imaginary part. For example, 3+4i is a complex number with a non-zero real and imaginary part.
Operations with Complex Numbers
There are several operations that can be performed with complex numbers, including:
- Addition: The sum of two complex numbers is obtained by adding their real parts and imaginary parts separately. For example, (3+4i) + (2+5i) = 5+9i.
- Subtraction: The difference of two complex numbers is obtained by subtracting their real parts and imaginary parts separately. For example, (3+4i) - (2+5i) = 1-i.
- Multiplication: The product of two complex numbers is obtained by multiplying their real parts and imaginary parts separately. For example, (3+4i) x (2+5i) = 6+15i+8i-20 = -14+23i.
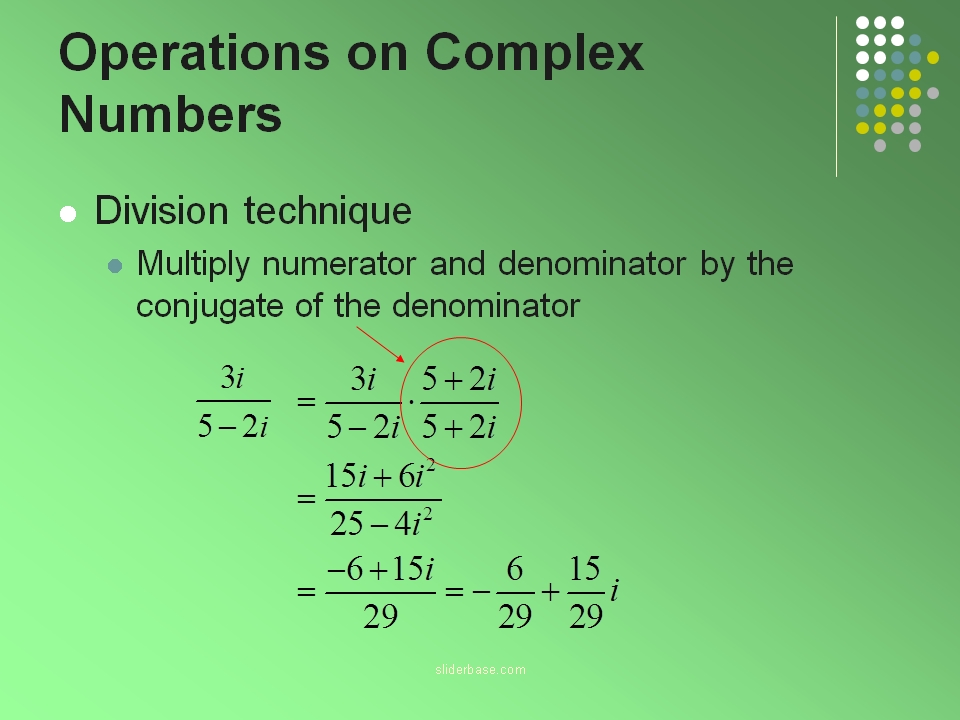
- Division: The quotient of two complex numbers is obtained by dividing their real parts and imaginary parts separately. For example, (3+4i) / (2+5i) = 1+i.
Simplifying Complex Numbers
Simplifying complex numbers involves converting them into their simplest form. This can be done by:
- Combining Like Terms: Combine the real parts and imaginary parts separately. For example, 3+4i+2+5i = 5+9i.
- Removing Zeroes: Remove any zeroes from the real or imaginary part. For example, 0+5i = 5i.
- Simplifying Fractions: Simplify any fractions in the real or imaginary part. For example, 1⁄2 + 3/4i = 1⁄4 + 3/4i.
Worksheet with Answers
Here is a worksheet with 10 questions to help you practice simplifying complex numbers:
| Question | Answer |
|---|---|
| 1. Simplify 3+4i+2+5i | 5+9i |
| 2. Simplify 0+5i+0+2i | 5i+2i = 7i |
| 3. Simplify 1/2 + 3/4i | 1/4 + 3/4i |
| 4. Simplify 2+5i-1-3i | 1+2i |
| 5. Simplify 3+4i x 2+5i | -14+23i |
| 6. Simplify 1/2 + 1/4i | 1/4 + 1/4i |
| 7. Simplify 0+5i-0-2i | 5i-2i = 3i |
| 8. Simplify 3+4i / 2+5i | 1+i |
| 9. Simplify 2+5i+1+3i | 3+8i |
| 10. Simplify 1/2 + 1/4i | 1/4 + 1/4i |
📝 Note: The answers to the worksheet are provided above each question.
In conclusion, simplifying complex numbers is an essential skill in mathematics, particularly in algebra and calculus. By combining like terms, removing zeroes, and simplifying fractions, you can simplify complex numbers and make them easier to work with. We hope this guide and worksheet have helped you practice simplifying complex numbers and improve your math skills.
What is a complex number?
+A complex number is a number that has both a real and an imaginary part, represented in the form a+bi.
How do I simplify complex numbers?
+To simplify complex numbers, combine like terms, remove zeroes, and simplify fractions.
What is the difference between a real number and an imaginary number?
+A real number has no imaginary part, while an imaginary number has no real part.