6 Ways to Solve One Step Equations Easily
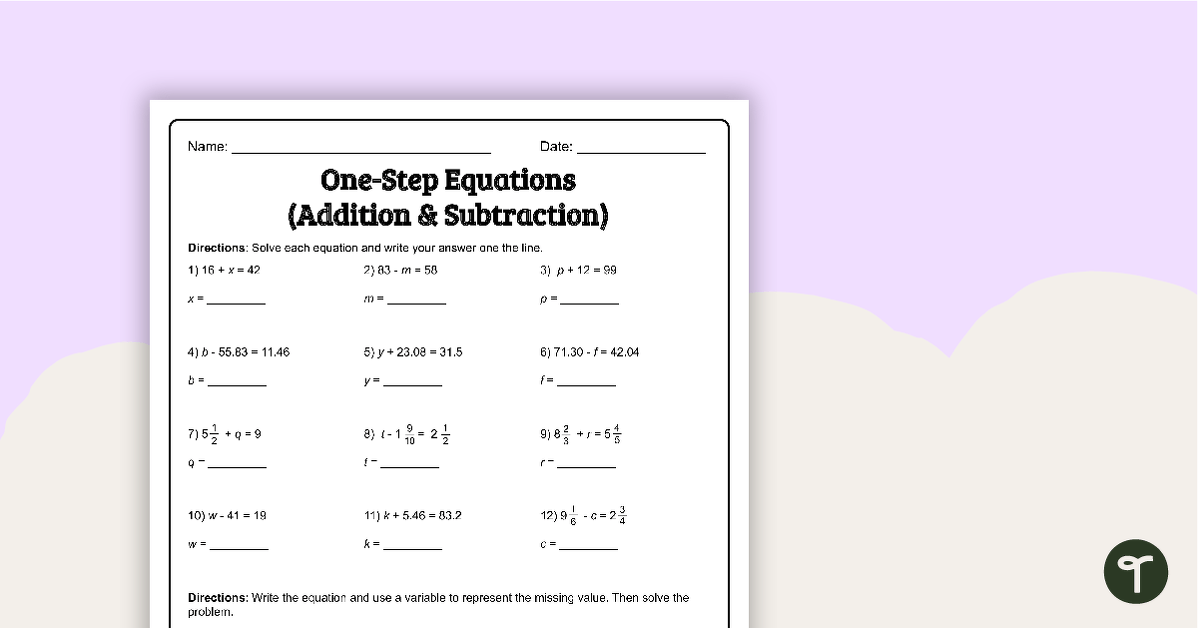
Understanding One Step Equations
One step equations are a fundamental concept in algebra, and they are used to solve equations that can be simplified in just one step. These equations typically involve addition, subtraction, multiplication, or division, and the goal is to isolate the variable, usually represented by a letter such as x.
Why are One Step Equations Important?
One step equations are crucial in mathematics because they serve as a building block for more complex equations. Mastering one step equations helps students develop problem-solving skills, critical thinking, and analytical reasoning. It also lays the groundwork for solving multi-step equations, linear equations, and other advanced mathematical concepts.
6 Ways to Solve One Step Equations Easily
1. Addition and Subtraction Method
This method involves isolating the variable by adding or subtracting the same value to both sides of the equation.
Example: 2x + 5 = 11
To solve for x, subtract 5 from both sides:
2x = 11 - 5 2x = 6 x = 6 ÷ 2 x = 3
📝 Note: When adding or subtracting, make sure to perform the same operation on both sides of the equation to maintain equality.
2. Multiplication and Division Method
This method involves isolating the variable by multiplying or dividing both sides of the equation by the same value.
Example: 4x = 24
To solve for x, divide both sides by 4:
x = 24 ÷ 4 x = 6
📝 Note: When multiplying or dividing, make sure to perform the same operation on both sides of the equation to maintain equality.
3. Balancing Method
This method involves balancing the equation by performing the inverse operation on both sides.
Example: x - 3 = 7
To solve for x, add 3 to both sides:
x = 7 + 3 x = 10
📝 Note: When using the balancing method, make sure to perform the inverse operation on both sides of the equation to maintain equality.
4. Inverse Operation Method
This method involves using the inverse operation to isolate the variable.
Example: x/2 = 9
To solve for x, multiply both sides by 2:
x = 9 × 2 x = 18
📝 Note: When using the inverse operation method, make sure to perform the inverse operation on both sides of the equation to maintain equality.
5. Working Backwards Method
This method involves working backwards to isolate the variable.
Example: x + 2 = 9
To solve for x, subtract 2 from both sides:
x = 9 - 2 x = 7
📝 Note: When using the working backwards method, make sure to perform the inverse operation on both sides of the equation to maintain equality.
6. Using Visual Models Method
This method involves using visual models to represent the equation and isolate the variable.
Example: 2x + 3 = 7
Use a number line or a hundreds chart to visualize the equation:
x + 3 = 7 x = 7 - 3 x = 4
📝 Note: When using visual models, make sure to represent the equation accurately and perform the correct operations to isolate the variable.
Conclusion
Solving one step equations is a fundamental skill in mathematics that requires practice and patience. By mastering the 6 methods outlined above, students can develop problem-solving skills, critical thinking, and analytical reasoning. Remember to always read the equation carefully, identify the operation, and perform the inverse operation to isolate the variable.
What is the purpose of one step equations?
+One step equations are used to develop problem-solving skills, critical thinking, and analytical reasoning, and they serve as a building block for more complex equations.
What are some common methods for solving one step equations?
+Some common methods for solving one step equations include the addition and subtraction method, multiplication and division method, balancing method, inverse operation method, working backwards method, and using visual models method.
Why is it important to perform the same operation on both sides of the equation?
+Performing the same operation on both sides of the equation maintains equality and ensures that the solution is accurate.
Related Terms:
- one-step equations addition worksheet
- One step Equations worksheets