5 Ways to Multiply Monomials with Ease
Understanding Monomials and Their Multiplication
Monomials are algebraic expressions consisting of a single term, which can be a number, a variable, or the product of numbers and variables. Multiplying monomials is a fundamental concept in algebra, and it’s essential to understand the process to simplify expressions and solve equations. In this article, we’ll explore five ways to multiply monomials with ease, making it a breeze for you to tackle algebraic problems.
Method 1: Multiplying Coefficients and Variables Separately
When multiplying monomials, you can separate the coefficients (numbers) from the variables and multiply them individually. This method is straightforward and helps you avoid confusion.
📝 Note: Coefficients are numbers that are multiplied by variables in an algebraic expression.
For example, let’s multiply the monomials 3x and 4y:
- Multiply the coefficients: 3 × 4 = 12
- Multiply the variables: x × y = xy
- Combine the results: 12xy
Method 2: Using the Associative Property
The associative property states that the order in which you multiply numbers or variables does not change the result. This property can help you multiply monomials by grouping coefficients and variables in a way that makes the calculation easier.
For instance, let’s multiply the monomials 2x, 3y, and 4z:
- Group the coefficients: 2 × 3 × 4 = 24
- Group the variables: x × y × z = xyz
- Combine the results: 24xyz
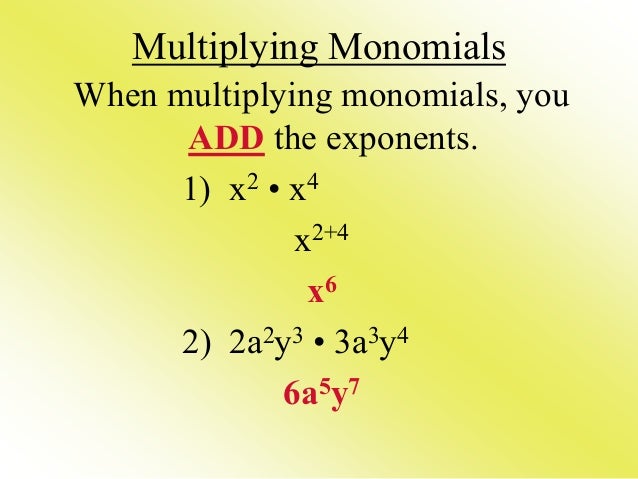
Method 3: Multiplying Monomials with Exponents
When multiplying monomials with exponents, you need to follow the rules of exponentiation. Specifically, when you multiply variables with the same base, you add their exponents.
For example, let’s multiply the monomials x² and x⁴:
- Multiply the coefficients: 1 × 1 = 1 ( implicit coefficient is 1)
- Add the exponents: 2 + 4 = 6
- Combine the results: x⁶
Method 4: Using the Distributive Property
The distributive property allows you to distribute a coefficient to multiple variables or terms. This property can help you multiply monomials by breaking them down into simpler expressions.
For instance, let’s multiply the monomials 2(x + y) and 3(z + w):
- Distribute the coefficients: 2 × 3 = 6
- Multiply the variables: x × z = xz, x × w = xw, y × z = yz, y × w = yw
- Combine the results: 6xz + 6xw + 6yz + 6yw
Method 5: Using Real-World Examples
Multiplying monomials can be more intuitive when you use real-world examples. For instance, imagine you have 3 groups of 4 pencils each. How many pencils do you have in total?
- Multiply the coefficients: 3 × 4 = 12
- Identify the variable: pencils
- Combine the results: 12 pencils
By using real-world examples, you can make the concept of multiplying monomials more relatable and easier to understand.
Common Mistakes to Avoid
When multiplying monomials, be careful not to make the following common mistakes:
- Forgetting to multiply coefficients
- Forgetting to multiply variables
- Incorrectly adding or subtracting exponents
- Not distributing coefficients correctly
By avoiding these mistakes, you can ensure that you’re multiplying monomials correctly and with confidence.
In conclusion, multiplying monomials is a fundamental concept in algebra that can be mastered with practice and patience. By using the five methods outlined in this article, you can become proficient in multiplying monomials and tackle more complex algebraic problems with ease.
What is a monomial in algebra?
+A monomial is an algebraic expression consisting of a single term, which can be a number, a variable, or the product of numbers and variables.
What is the associative property in algebra?
+The associative property states that the order in which you multiply numbers or variables does not change the result.
How do you multiply monomials with exponents?
+When multiplying monomials with exponents, you add the exponents when the variables have the same base.