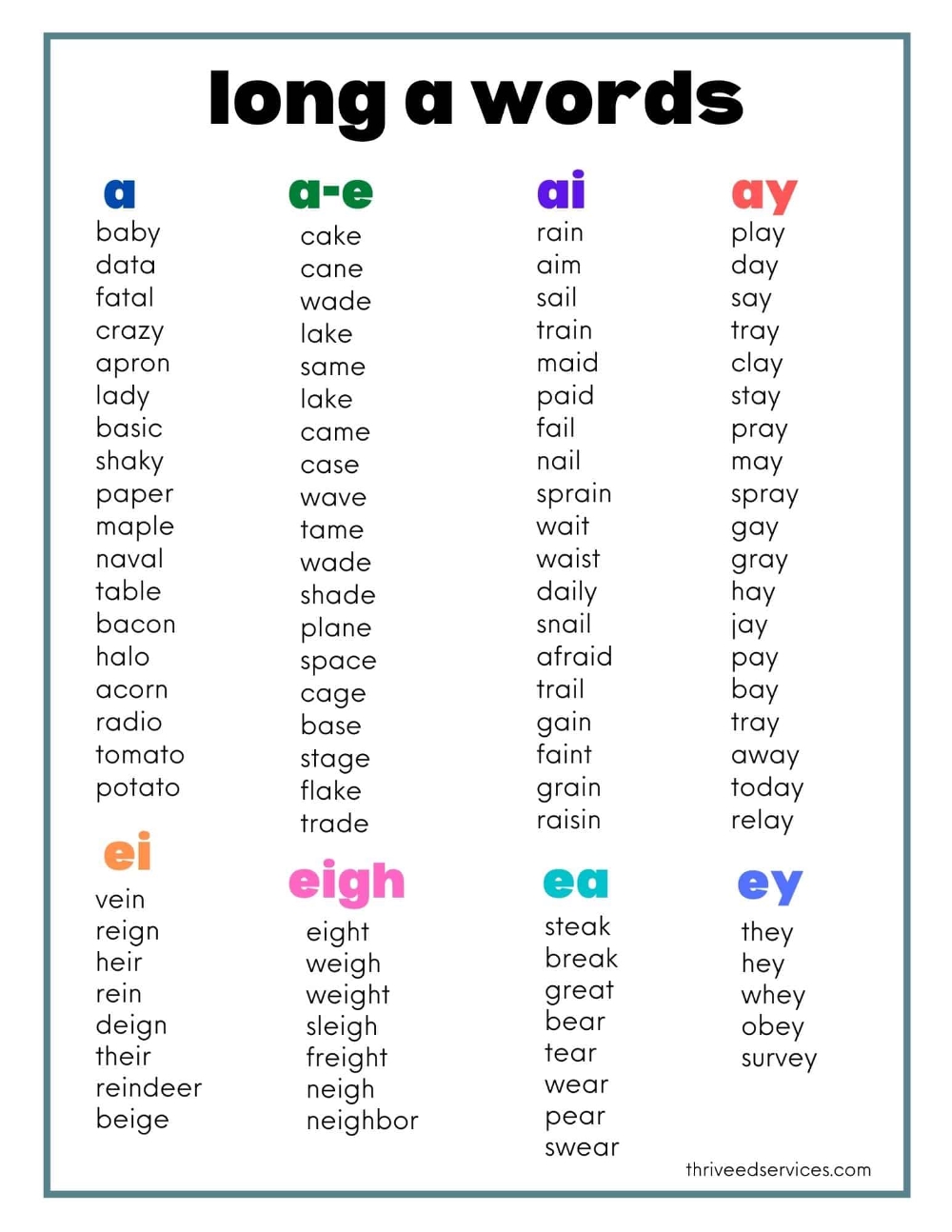
Multiplying Fractions Made Easy with Cross Cancelling

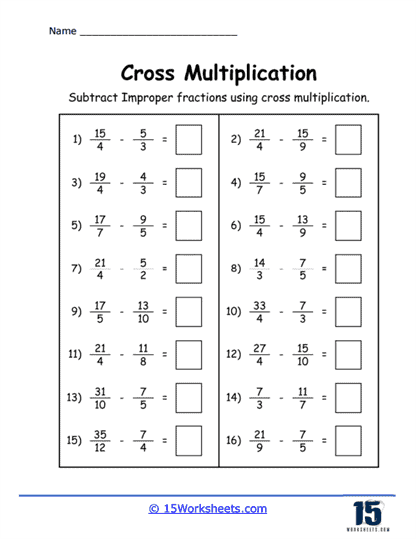
Understanding the Basics of Multiplying Fractions

Multiplying fractions is a fundamental concept in mathematics that can seem intimidating at first, but with the right techniques, it can be made easy. One such technique is cross cancelling, which simplifies the process of multiplying fractions by eliminating common factors. In this article, we will explore the concept of multiplying fractions and learn how to apply the cross cancelling technique to make calculations easier.

The Concept of Multiplying Fractions

Multiplying fractions involves multiplying the numerators (the numbers on top) and the denominators (the numbers on the bottom) separately. The formula for multiplying fractions is:

Numerator 1 x Numerator 2 / Denominator 1 x Denominator 2

For example, let’s multiply two fractions:

¹⁄₂ x ³⁄₄

Using the formula, we get:

1 x 3 / 2 x 4 = ³⁄₈

What is Cross Cancelling?

Cross cancelling is a technique used to simplify the process of multiplying fractions by eliminating common factors between the numerators and denominators. This technique involves cancelling out the common factors before multiplying the fractions.

How to Apply Cross Cancelling

To apply cross cancelling, follow these steps:

1. Identify the common factors between the numerators and denominators.
2. Cancel out the common factors by drawing a line through them.
3. Multiply the remaining numbers.

Let’s use the example from earlier to demonstrate cross cancelling:

¹⁄₂ x ³⁄₄

Identify the common factors:

• The numerator 1 and the denominator 2 have a common factor of 1.
• The numerator 3 and the denominator 4 have a common factor of 1.

Cancel out the common factors:

¹⁄₂ x ³⁄₄ = 1/ / 3/ = ³⁄₄ x ¹⁄₂ = ³⁄₈

As you can see, cross cancelling simplifies the calculation and reduces the risk of errors.

Examples of Cross Cancelling

Here are some examples to illustrate the concept of cross cancelling:

• ²⁄₃ x ³⁄₅

Cancel out the common factor 3:

²⁄₃ x ³⁄₅ = 2/ x ¹⁄₅ = ²⁄₅

• ¹⁄₄ x ⁴⁄₉

Cancel out the common factor 4:

¹⁄₄ x ⁴⁄₉ = 1/ x ¹⁄₉ = ¹⁄₉

Notes

📝 Note: Cross cancelling can only be applied when there are common factors between the numerators and denominators. If there are no common factors, the fractions must be multiplied using the traditional method.

📝 Note: Cross cancelling is a useful technique for simplifying fraction calculations, but it is essential to check the final answer for accuracy.

Conclusion

Multiplying fractions can be made easy with the cross cancelling technique. By eliminating common factors between the numerators and denominators, you can simplify the calculation and reduce the risk of errors. With practice, cross cancelling becomes second nature, and you will be able to tackle even the most complex fraction calculations with confidence.

Related Terms:

• Cross cancellation worksheet with answers
• Multiplying fractions with cross canceling