5 Ways to Master Momentum Practice Problems
Understanding Momentum and Its Importance in Physics
Momentum is a fundamental concept in physics that is crucial for understanding various phenomena in the natural world. It is defined as the product of an object’s mass and velocity, and it plays a vital role in describing the motion of objects. Mastering momentum practice problems is essential for any physics student, as it helps in developing problem-solving skills and deepening understanding of the subject.
Why Practice Problems Are Essential for Mastering Momentum
Practice problems are an integral part of learning physics, and momentum is no exception. By solving practice problems, students can:
- Develop problem-solving skills and strategies
- Improve understanding of momentum concepts and formulas
- Enhance critical thinking and analytical skills
- Build confidence in solving complex problems
5 Ways to Master Momentum Practice Problems
1. Understand the Basics of Momentum
Before diving into practice problems, it’s essential to understand the basics of momentum. This includes:
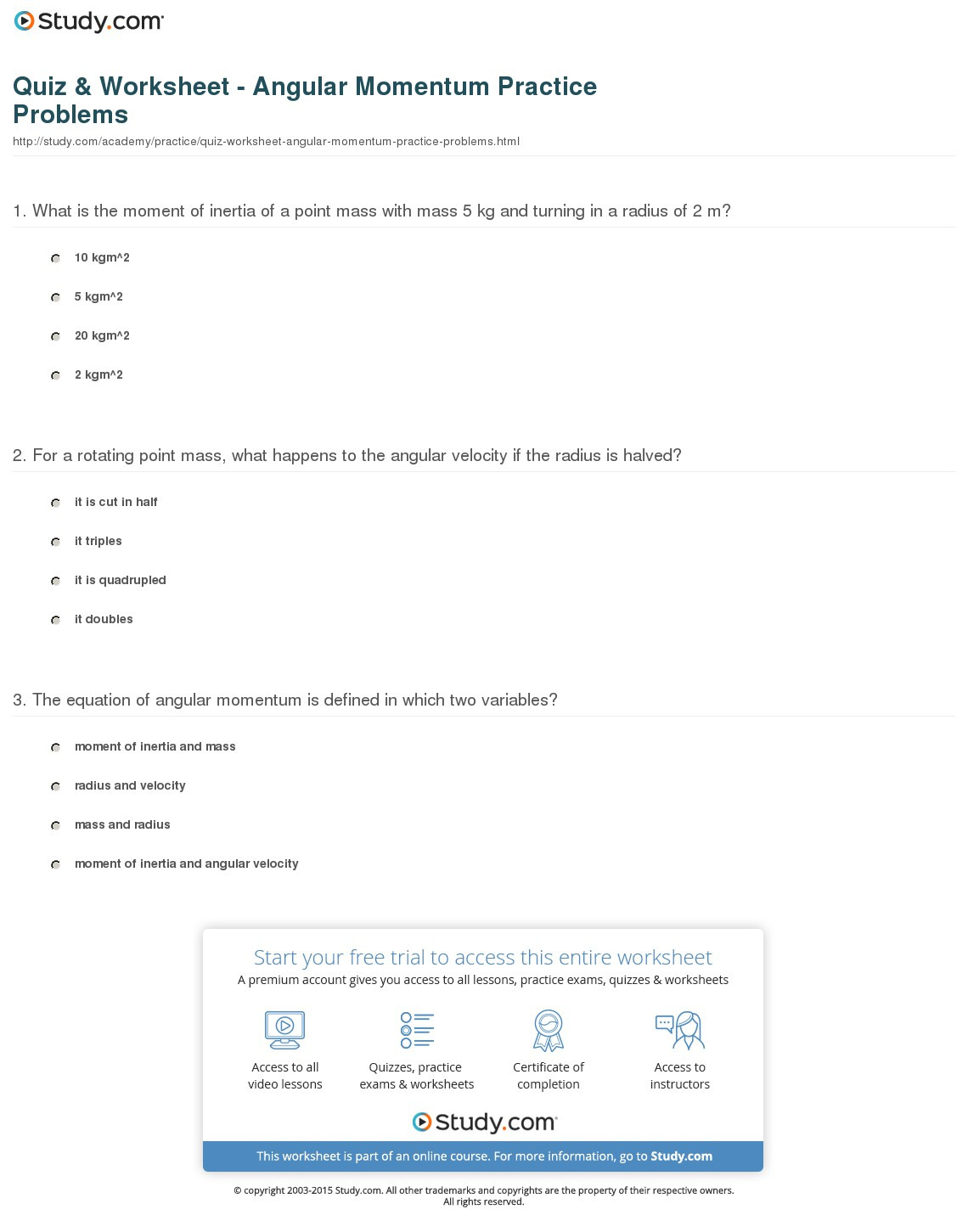
- Definition of momentum: Momentum (p) is the product of an object’s mass (m) and velocity (v): p = m × v
- Units of momentum: The unit of momentum is kilogram-meter per second (kg·m/s)
- Types of momentum: There are two types of momentum: linear momentum and angular momentum
2. Practice Different Types of Problems
To master momentum practice problems, it’s crucial to practice different types of problems, including:
- Simple problems: Problems involving simple calculations, such as finding the momentum of an object given its mass and velocity
- Complex problems: Problems involving multiple objects, collisions, and energy transformations
- Word problems: Problems that require critical thinking and application of momentum concepts to real-world scenarios
3. Use Visual Aids and Diagrams
Visual aids and diagrams can help in understanding complex momentum problems. Use:
- Free-body diagrams: To visualize forces acting on an object
- Motion diagrams: To visualize the motion of an object
- Graphs: To visualize relationships between momentum and other physical quantities
4. Break Down Complex Problems
Complex momentum problems can be overwhelming, but breaking them down into simpler steps can make them more manageable. Use:
- Step-by-step approach: Break down complex problems into simpler steps
- Identify given information: Identify the given information and the unknown quantities
- Apply momentum formulas: Apply relevant momentum formulas to solve the problem
5. Review and Reflect
Finally, reviewing and reflecting on practice problems is essential for mastering momentum. Use:
- Review notes: Review class notes and textbook sections on momentum
- Reflect on mistakes: Reflect on mistakes and identify areas for improvement
- Practice regularly: Practice regularly to reinforce understanding and build confidence
📝 Note: Consistency is key when practicing momentum problems. Set aside a specific time each day or week to practice, and review notes regularly.
Common Mistakes to Avoid When Solving Momentum Practice Problems
When solving momentum practice problems, there are several common mistakes to avoid, including:
- Unit mistakes: Ensure that units are consistent throughout the problem
- Sign mistakes: Pay attention to signs when adding or subtracting velocities
- Formula mistakes: Use the correct momentum formulas for each problem
| Momentum Formula | Description |
|---|---|
| p = m × v | Linear momentum formula |
| L = r × m × v | Angular momentum formula |
| Δp = F × Δt | Impulse-momentum theorem |
What is the difference between linear and angular momentum?
+Linear momentum is the product of an object's mass and velocity, while angular momentum is the product of an object's moment of inertia and angular velocity.
How do I know which momentum formula to use?
+Use the linear momentum formula (p = m × v) for problems involving linear motion, and the angular momentum formula (L = r × m × v) for problems involving rotational motion.
What is the impulse-momentum theorem?
+The impulse-momentum theorem states that the impulse of a force is equal to the change in momentum of an object (Δp = F × Δt).
Mastering momentum practice problems requires a combination of understanding the basics, practicing different types of problems, using visual aids, breaking down complex problems, and reviewing and reflecting regularly. By following these steps and avoiding common mistakes, you can develop a deep understanding of momentum and improve your problem-solving skills.
Related Terms:
- Momentum Worksheet with answers PDF
- Momentum Practice Problems worksheet answers
- Momentum Worksheet PDF
- Momentum Problems with Solutions
- Momentum word problems answers