5 Ways to Master Midpoint and Distance Formula
Understanding the Midpoint and Distance Formula
The midpoint and distance formula are essential concepts in mathematics, particularly in geometry and trigonometry. These formulas help calculate the midpoint of a line segment and the distance between two points in a coordinate plane. Mastering these formulas is crucial for problem-solving and critical thinking in mathematics. In this article, we will explore five ways to master the midpoint and distance formula.
1. Understanding the Midpoint Formula
The midpoint formula is used to find the midpoint of a line segment given the coordinates of its endpoints. The formula is:
Midpoint = ((x1 + x2)/2, (y1 + y2)/2)
where (x1, y1) and (x2, y2) are the coordinates of the endpoints.
To master the midpoint formula, it’s essential to understand the concept of averages. The midpoint of a line segment is the average of the x-coordinates and the average of the y-coordinates. By applying this concept, you can easily find the midpoint of a line segment.
Example:
Find the midpoint of the line segment with endpoints (2, 3) and (4, 5).
Using the midpoint formula:
Midpoint = ((2 + 4)/2, (3 + 5)/2) Midpoint = (6⁄2, 8⁄2) Midpoint = (3, 4)
📝 Note: The midpoint formula can be applied to find the midpoint of a line segment in any coordinate plane.
2. Understanding the Distance Formula
The distance formula is used to find the distance between two points in a coordinate plane. The formula is:
Distance = √((x2 - x1)^2 + (y2 - y1)^2)
where (x1, y1) and (x2, y2) are the coordinates of the points.
To master the distance formula, it’s essential to understand the concept of Pythagoras’ theorem. The distance formula is an application of Pythagoras’ theorem in a coordinate plane.
Example:
Find the distance between the points (2, 3) and (4, 5).
Using the distance formula:
Distance = √((4 - 2)^2 + (5 - 3)^2) Distance = √(2^2 + 2^2) Distance = √(8) Distance = 2√2
📝 Note: The distance formula can be applied to find the distance between any two points in a coordinate plane.
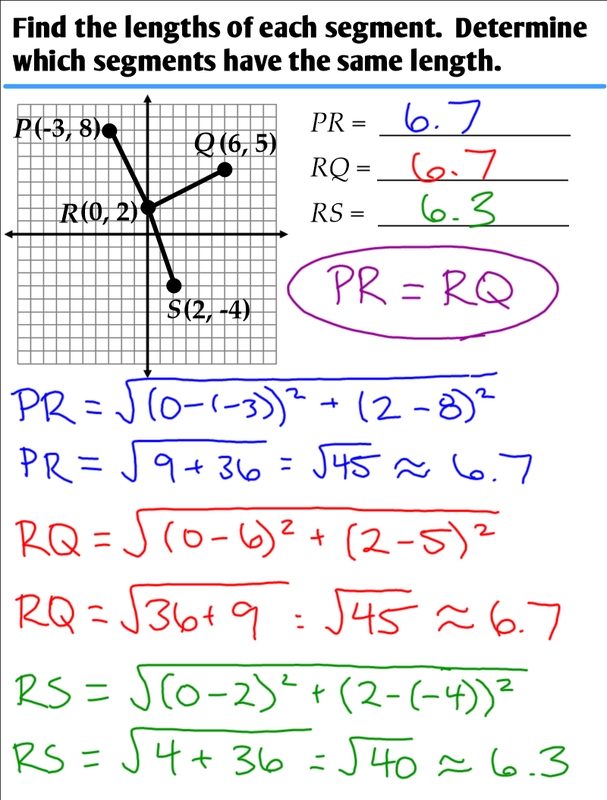
3. Visualizing the Midpoint and Distance Formula
Visualizing the midpoint and distance formula can help you understand and master these concepts better. You can use graph paper to plot the points and draw the line segments. By visualizing the midpoint and distance formula, you can see how these formulas work in practice.

| Midpoint | Distance |
|---|---|
| Find the midpoint of a line segment | Find the distance between two points |
| Use the midpoint formula: ((x1 + x2)/2, (y1 + y2)/2) | Use the distance formula: √((x2 - x1)^2 + (y2 - y1)^2) |
4. Practicing with Examples
Practicing with examples is an excellent way to master the midpoint and distance formula. You can find many examples online or create your own examples using graph paper. By practicing with examples, you can reinforce your understanding of these formulas.
Example:
Find the midpoint and distance between the points (1, 2) and (3, 4).
Using the midpoint formula:
Midpoint = ((1 + 3)/2, (2 + 4)/2) Midpoint = (4⁄2, 6⁄2) Midpoint = (2, 3)
Using the distance formula:
Distance = √((3 - 1)^2 + (4 - 2)^2) Distance = √(2^2 + 2^2) Distance = √(8) Distance = 2√2
5. Applying the Midpoint and Distance Formula in Real-Life Scenarios
The midpoint and distance formula have many real-life applications. You can use these formulas to find the midpoint and distance between two cities, the distance between two objects, or the midpoint of a bridge. By applying the midpoint and distance formula in real-life scenarios, you can see the practicality of these formulas.
Example:
A surveyor wants to find the midpoint of a bridge that spans a river. The coordinates of the two endpoints of the bridge are (100, 200) and (150, 300). Find the midpoint of the bridge.
Using the midpoint formula:
Midpoint = ((100 + 150)/2, (200 + 300)/2) Midpoint = (250⁄2, 500⁄2) Midpoint = (125, 250)
By mastering the midpoint and distance formula, you can solve many problems in mathematics and real-life scenarios.
The midpoint and distance formula are essential concepts in mathematics that have many real-life applications. By understanding and mastering these formulas, you can solve many problems and reinforce your critical thinking skills. Remember to practice with examples, visualize the formulas, and apply them in real-life scenarios to become proficient in using these formulas.