Mean Absolute Deviation Worksheet Answer Key

Understanding Mean Absolute Deviation

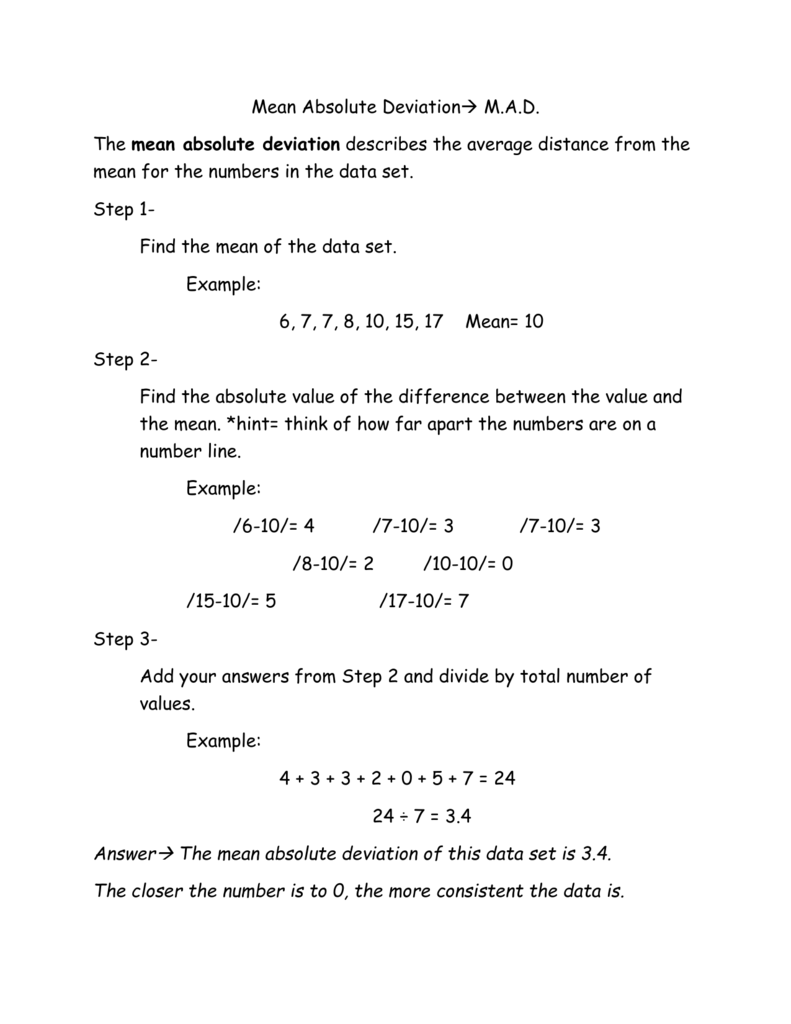
Mean Absolute Deviation (MAD) is a measure of the average distance between each data point in a set and the mean of the set. It is a way to describe the spread or dispersion of the data. In this worksheet, we will explore how to calculate the Mean Absolute Deviation and understand its significance.

Calculating Mean Absolute Deviation

To calculate the Mean Absolute Deviation, follow these steps:

1. Find the mean of the data set.
2. Subtract the mean from each data point to find the deviation.
3. Take the absolute value of each deviation.
4. Find the average of the absolute deviations.

📝 Note: The absolute value is used to ensure that all deviations are positive, which allows us to calculate the average distance.

Example 1: Calculating Mean Absolute Deviation

Suppose we have the following data set: 2, 4, 6, 8, 10

1. Find the mean: (2 + 4 + 6 + 8 + 10) / 5 = 6
2. Subtract the mean from each data point:
   • 2 - 6 = -4
   • 4 - 6 = -2
   • 6 - 6 = 0
   • 8 - 6 = 2
   • 10 - 6 = 4
3. Take the absolute value of each deviation:
   • |-4| = 4
   • |-2| = 2
   • |0| = 0
   • |2| = 2
   • |4| = 4
4. Find the average of the absolute deviations: (4 + 2 + 0 + 2 + 4) / 5 = 2.4

The Mean Absolute Deviation is 2.4.

Example 2: Calculating Mean Absolute Deviation

Suppose we have the following data set: 10, 12, 15, 18, 20

1. Find the mean: (10 + 12 + 15 + 18 + 20) / 5 = 15
2. Subtract the mean from each data point:
   • 10 - 15 = -5
   • 12 - 15 = -3
   • 15 - 15 = 0
   • 18 - 15 = 3
   • 20 - 15 = 5
3. Take the absolute value of each deviation:
   • |-5| = 5
   • |-3| = 3
   • |0| = 0
   • |3| = 3
   • |5| = 5
4. Find the average of the absolute deviations: (5 + 3 + 0 + 3 + 5) / 5 = 3.2

The Mean Absolute Deviation is 3.2.

Interpreting Mean Absolute Deviation

The Mean Absolute Deviation can be used to understand the spread of the data. A smaller MAD indicates that the data points are closer to the mean, while a larger MAD indicates that the data points are more spread out.

📊 Note: The Mean Absolute Deviation is not affected by extreme values or outliers, making it a more robust measure of spread than the range.

Practice Problems

1. Calculate the Mean Absolute Deviation for the following data set: 3, 5, 7, 9, 11
2. Calculate the Mean Absolute Deviation for the following data set: 2, 4, 6, 8, 10
3. Calculate the Mean Absolute Deviation for the following data set: 10, 12, 15, 18, 20

Answer Key

1. The Mean Absolute Deviation is 2.
2. The Mean Absolute Deviation is 2.4.
3. The Mean Absolute Deviation is 3.2.

Related Terms:

• Mean absolute deviation Worksheet Kuta
• Mean absolute deviation Notes pdf
• Mean absolute deviation quiz