5 Essential Math Properties to Master

Understanding the Basics: 5 Essential Math Properties to Master

Mathematics is built on a foundation of fundamental properties that enable us to solve equations, manipulate expressions, and model real-world phenomena. Mastering these essential math properties is crucial for success in various mathematical disciplines, including algebra, geometry, calculus, and beyond. In this article, we will explore five fundamental math properties that every student should know and understand.

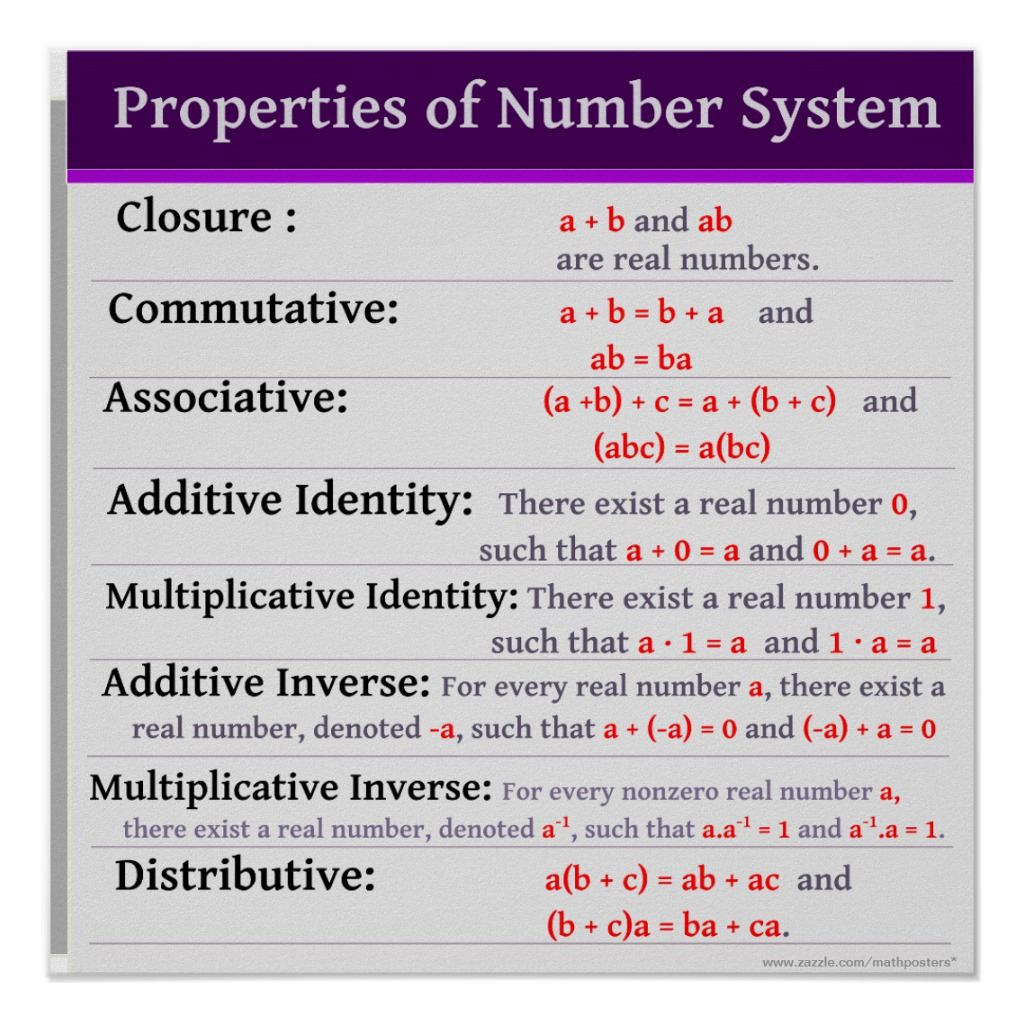
1. The Commutative Property

The commutative property states that the order of the numbers or variables being added or multiplied does not change the result. In other words, a + b = b + a and a × b = b × a. This property applies to addition and multiplication, but not to subtraction or division.

For example:

• 2 + 3 = 3 + 2 = 5
• 4 × 5 = 5 × 4 = 20

The commutative property allows us to rearrange the numbers or variables in an expression without changing its value, making it easier to solve equations and manipulate expressions.

2. The Associative Property

The associative property states that when we add or multiply three or more numbers, the order in which we perform the operations does not change the result. In other words, (a + b) + c = a + (b + c) and (a × b) × c = a × (b × c).

For example:

• (2 + 3) + 4 = 2 + (3 + 4) = 9
• (4 × 5) × 2 = 4 × (5 × 2) = 40

The associative property enables us to regroup numbers or variables in an expression to simplify calculations or solve equations.

3. The Distributive Property

The distributive property states that when we multiply a single value to multiple values inside parentheses, we can distribute the multiplication to each value separately. In other words, a × (b + c) = a × b + a × c.

For example:

• 2 × (3 + 4) = 2 × 3 + 2 × 4 = 14
• 5 × (2 + 1) = 5 × 2 + 5 × 1 = 15

The distributive property allows us to expand and simplify expressions by distributing the multiplication.

4. The Identity Property

The identity property states that there exists a unique value, called the identity element, which does not change the result when added or multiplied to any number. For addition, the identity element is 0, and for multiplication, the identity element is 1.

For example:

• 2 + 0 = 2
• 4 × 1 = 4

The identity property provides a reference point for understanding the behavior of numbers and variables in different mathematical operations.

5. The Inverse Property

The inverse property states that for every number or variable, there exists an inverse value that, when added or multiplied, results in the identity element. For addition, the inverse is -a, and for multiplication, the inverse is 1/a.

For example:

• 2 + (-2) = 0
• 4 × (¹⁄₄) = 1

The inverse property enables us to find the opposite or reciprocal of a number or variable, which is essential for solving equations and working with variables.

🔥 Note: Mastering these five essential math properties takes practice and patience. Be sure to practice each property with different numbers and variables to build a strong foundation in mathematics.

To summarize, understanding and mastering the commutative, associative, distributive, identity, and inverse properties are crucial for success in mathematics. These properties provide the building blocks for more advanced mathematical concepts and enable us to solve equations, manipulate expressions, and model real-world phenomena with confidence.