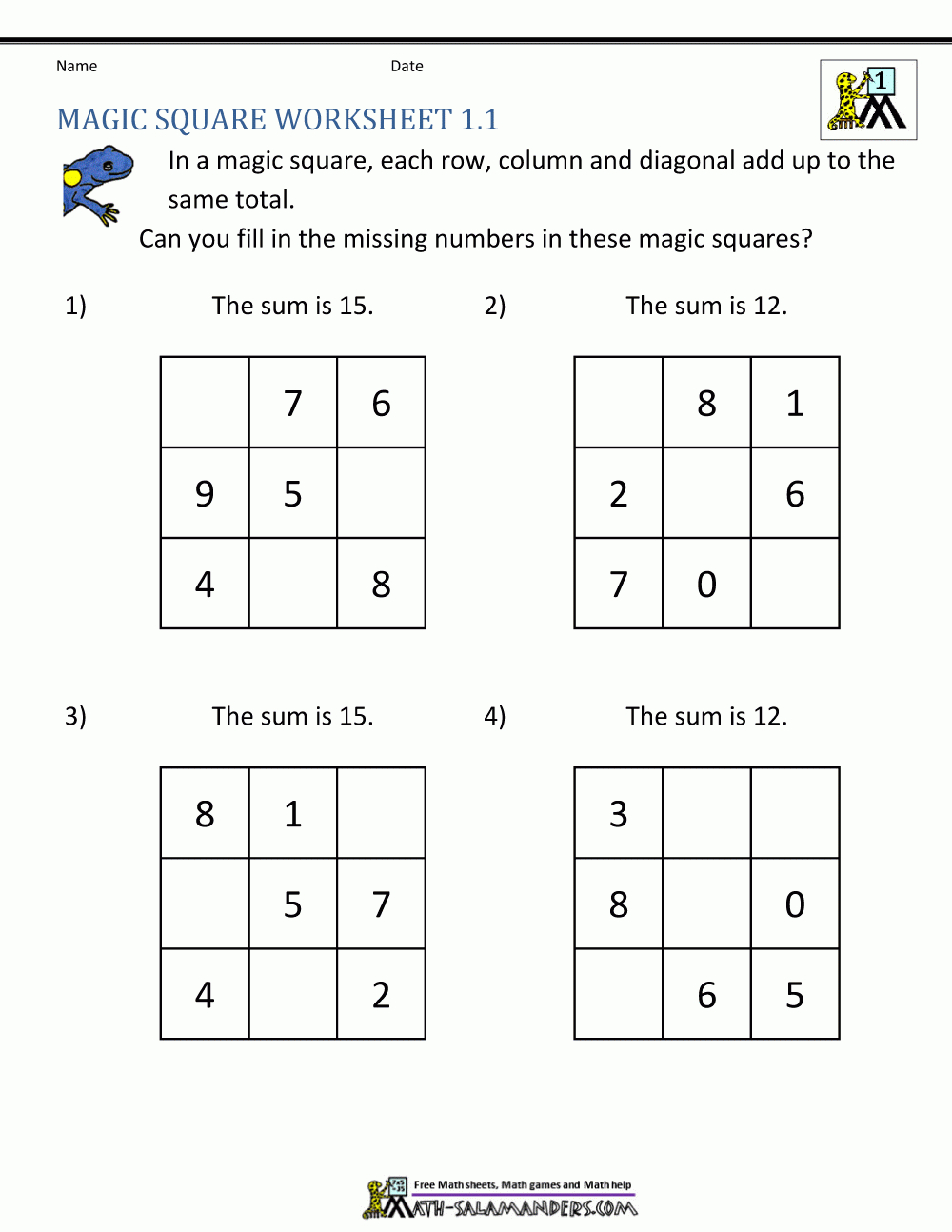
Mastering Long Division with Remainders Made Easy

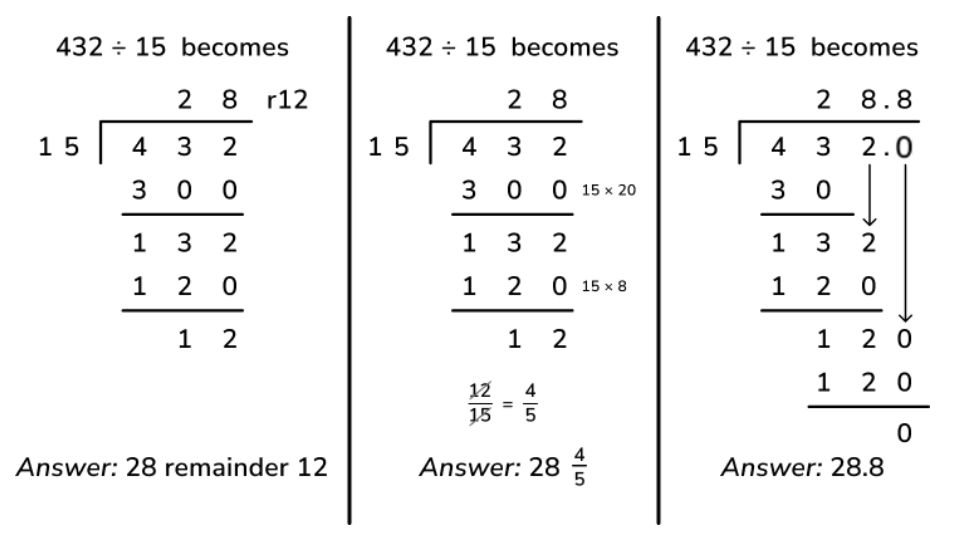
Understanding the Basics of Long Division

Long division is a mathematical operation used to divide a large number (dividend) by a smaller number (divisor) to find the quotient and remainder. It’s a fundamental concept in mathematics, and mastering it can help you solve various problems in algebra, geometry, and other math disciplines. In this article, we’ll focus on long division with remainders and provide a step-by-step guide on how to perform it easily.

The Long Division Formula

The long division formula is:

Dividend (large number)

÷

Divisor (smaller number)

=

Quotient (result of division)

+

Remainder (amount left over)

Step-by-Step Guide to Long Division with Remainders

Here’s a step-by-step guide to performing long division with remainders:

Step 1: Write the dividend and divisor

Write the dividend (large number) on top of a line, and the divisor (smaller number) below it.

Step 2: Divide the first digit of the dividend by the divisor

Divide the first digit of the dividend by the divisor, and write the result below the line.

Step 3: Multiply the result by the divisor and subtract

Multiply the result from Step 2 by the divisor, and subtract the product from the dividend.

Step 4: Bring down the next digit of the dividend

Bring down the next digit of the dividend, and repeat the process from Step 2.

Step 5: Continue the process until the remainder is less than the divisor

Continue the process until the remainder is less than the divisor. This will give you the final quotient and remainder.

Example Problem: Long Division with Remainders

Let’s use the example problem: 432 ÷ 12 =?

Step 1: Write the dividend and divisor

Step 2: Divide the first digit of the dividend by the divisor

Divide 4 by 12: 4 ÷ 12 = 0 with a remainder of 4.

Step 3: Multiply the result by the divisor and subtract

Multiply 0 by 12: 0 × 12 = 0. Subtract 0 from 4: 4 - 0 = 4.

Step 4: Bring down the next digit of the dividend

Bring down the next digit (3): 43.

Step 5: Continue the process

Continue the process until the remainder is less than the divisor:

43 ÷ 12 = 3 with a remainder of 7

Multiply 3 by 12: 3 × 12 = 36. Subtract 36 from 43: 43 - 36 = 7.

Bring down the next digit (2): 72.

72 ÷ 12 = 6 with a remainder of 0.

The final quotient and remainder are:

Quotient: 36 Remainder: 0

Answer: 432 ÷ 12 = 36 with a remainder of 0.

Tips and Tricks for Mastering Long Division with Remainders

Here are some tips and tricks to help you master long division with remainders:

• Use a chart or diagram: Create a chart or diagram to help you visualize the process and keep track of the dividend, divisor, quotient, and remainder.
• Check your work: Check your work by multiplying the quotient by the divisor and adding the remainder. This should equal the dividend.
• Practice, practice, practice: Practice long division with remainders regularly to build your skills and confidence.

📝 Note: Make sure to check your work and use a chart or diagram to help you visualize the process. This will help you avoid mistakes and ensure accuracy.

Long division with remainders may seem daunting at first, but with practice and patience, you can master it. Remember to follow the step-by-step guide, use a chart or diagram, and check your work to ensure accuracy. With time and practice, you’ll become proficient in long division with remainders and be able to tackle more complex math problems with confidence.