5 Ways to Solve Triangles with Sine and Cosine Laws
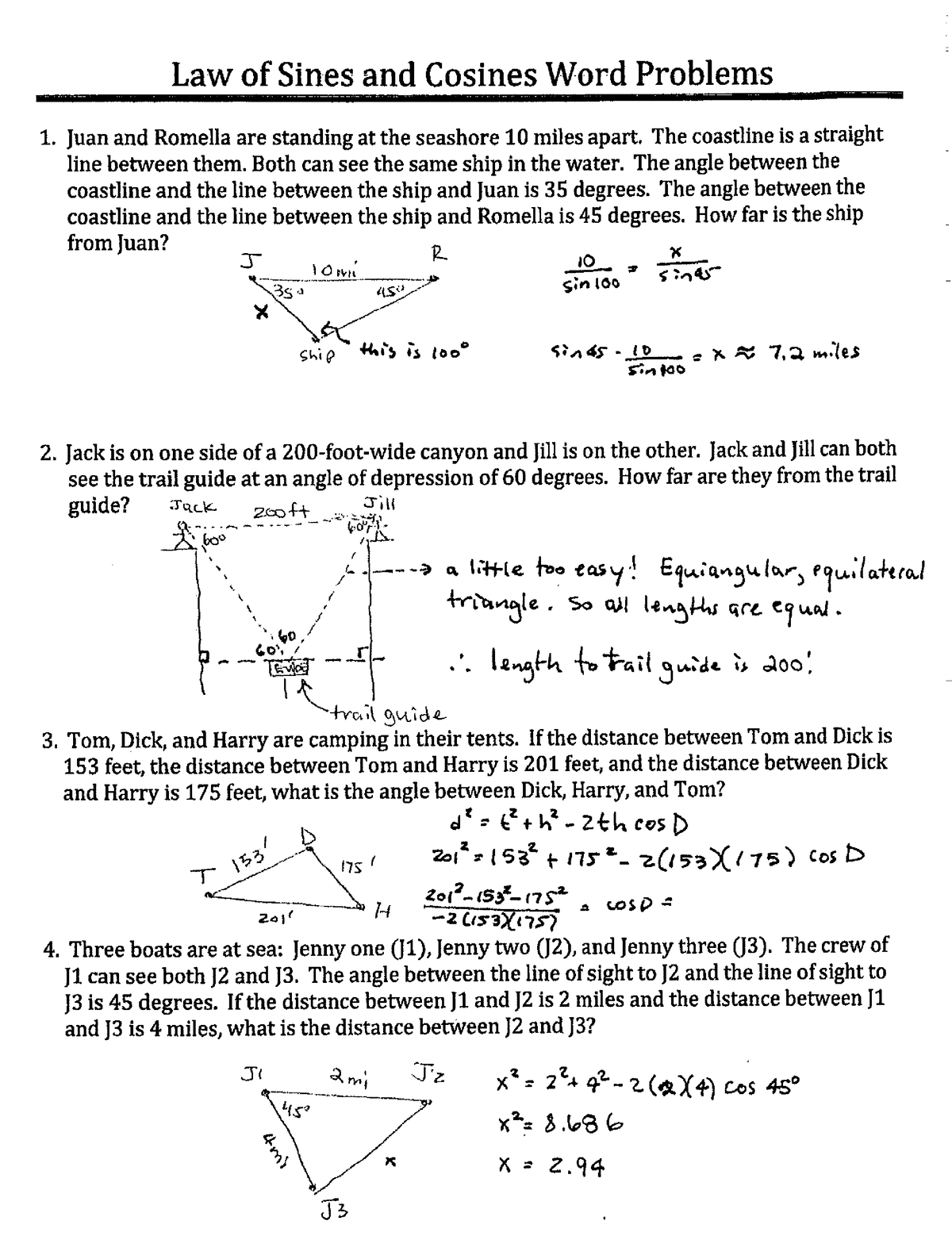
Understanding the Basics of Sine and Cosine Laws
When dealing with triangles, especially those that are not right-angled, the sine and cosine laws become invaluable tools for finding missing sides and angles. These laws, also known as the law of sines and the law of cosines, are fundamental principles in trigonometry that help in solving oblique triangles. In this article, we will delve into the world of triangles and explore five ways to solve them using the sine and cosine laws.
What are the Sine and Cosine Laws?
The Law of Sines: The law of sines states that for any given triangle with sides of length a, b, and c, and opposite angles A, B, and C, respectively, the following relationship holds:
a / sin(A) = b / sin(B) = c / sin©
This law is useful for finding missing sides or angles in a triangle when you know at least one side and its opposite angle, and another side or angle.
The Law of Cosines: The law of cosines, on the other hand, relates the lengths of the sides of a triangle to the cosine of one of its angles. The formula is:
c² = a² + b² - 2ab * cos©
This law is particularly useful for finding the length of the third side of a triangle when you know the lengths of the other two sides and the included angle.
Method 1: Finding a Missing Side with the Law of Sines
Let’s consider a scenario where we have a triangle with sides a, b, and c, and we know the length of side a, angle A, and angle B. We can use the law of sines to find the length of side b.
Given: - a = 10 cm - A = 60° - B = 70°
We need to find b.
Using the law of sines:
a / sin(A) = b / sin(B)
10 / sin(60°) = b / sin(70°)
Solving for b:
b = (10 * sin(70°)) / sin(60°)
b ≈ 11.04 cm
Notes on Calculation
When solving for missing sides or angles, it’s essential to ensure that your calculator is set to the correct mode (degrees or radians) to avoid errors in calculation.
Method 2: Finding an Angle with the Law of Cosines
Now, let’s say we have a triangle with sides a, b, and c, and we know the lengths of all three sides. We can use the law of cosines to find one of the angles.
Given: - a = 8 cm - b = 10 cm - c = 12 cm
We need to find angle C.
Using the law of cosines:
c² = a² + b² - 2ab * cos©
12² = 8² + 10² - 2 * 8 * 10 * cos©
Solving for cos©:
cos© = (8² + 10² - 12²) / (2 * 8 * 10)
cos© = -0.25
C = arccos(-0.25)
C ≈ 103.9°
Method 3: Solving for a Side with the Law of Cosines
We can also use the law of cosines to find the length of a side when we know the lengths of the other two sides and the included angle.
Given: - a = 15 cm - b = 20 cm - C = 120°
We need to find c.
Using the law of cosines:
c² = a² + b² - 2ab * cos©
c² = 15² + 20² - 2 * 15 * 20 * cos(120°)
Solving for c:
c² = 625 - 600 * (-0.5)
c² = 625 + 300
c = √925
c ≈ 30.41 cm
Method 4: Using the Law of Sines to Find an Angle
When we know the lengths of two sides and one angle, we can use the law of sines to find another angle.
Given: - a = 12 cm - b = 15 cm - A = 40°
We need to find angle B.
Using the law of sines:
a / sin(A) = b / sin(B)
12 / sin(40°) = 15 / sin(B)
Solving for sin(B):
sin(B) = (15 * sin(40°)) / 12
sin(B) ≈ 0.766
B ≈ arcsin(0.766)
B ≈ 50°
Method 5: Solving a Triangle with Two Sides and the Included Angle
Finally, when we know two sides and the included angle, we can use both the law of cosines and the law of sines to solve the triangle.
Given: - a = 10 cm - b = 12 cm - C = 60°
We need to find c and angle A.
Using the law of cosines to find c:
c² = a² + b² - 2ab * cos©
c² = 10² + 12² - 2 * 10 * 12 * cos(60°)
Solving for c:
c² = 100 + 144 - 240 * 0.5
c² = 244 - 120
c = √124
c ≈ 11.14 cm
Now, using the law of sines to find angle A:
a / sin(A) = c / sin©
10 / sin(A) = 11.14 / sin(60°)
Solving for sin(A):
sin(A) = (10 * sin(60°)) / 11.14
sin(A) ≈ 0.806
A ≈ arcsin(0.806)
A ≈ 53.5°
Conclusion
In conclusion, the sine and cosine laws are powerful tools for solving triangles. By understanding how to apply these laws, you can find missing sides and angles in a variety of scenarios. Remember to always check your calculator settings and to consider the context of the problem to ensure accurate calculations.
What is the difference between the law of sines and the law of cosines?
+The law of sines relates the lengths of the sides of a triangle to the sines of its angles, while the law of cosines relates the lengths of the sides of a triangle to the cosine of one of its angles.
When should I use the law of sines versus the law of cosines?
+Use the law of sines when you know at least one side and its opposite angle, and another side or angle. Use the law of cosines when you know the lengths of two sides and the included angle, or when you know the lengths of all three sides.
Can I use the law of sines and the law of cosines to solve right triangles?
+Yes, you can use both laws to solve right triangles, but it’s often easier to use the Pythagorean theorem or trigonometric ratios like sine, cosine, and tangent, which are more straightforward for right triangles.
Related Terms:
- Law of Sines Worksheet pdf
- Law of Cosines Worksheet pdf