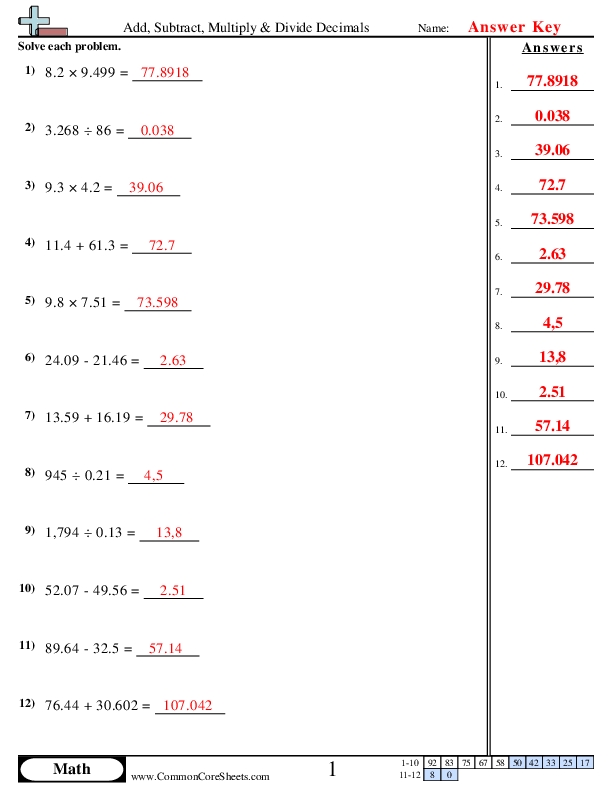
Graphing Rational Functions Worksheet Answers and Solutions Guide
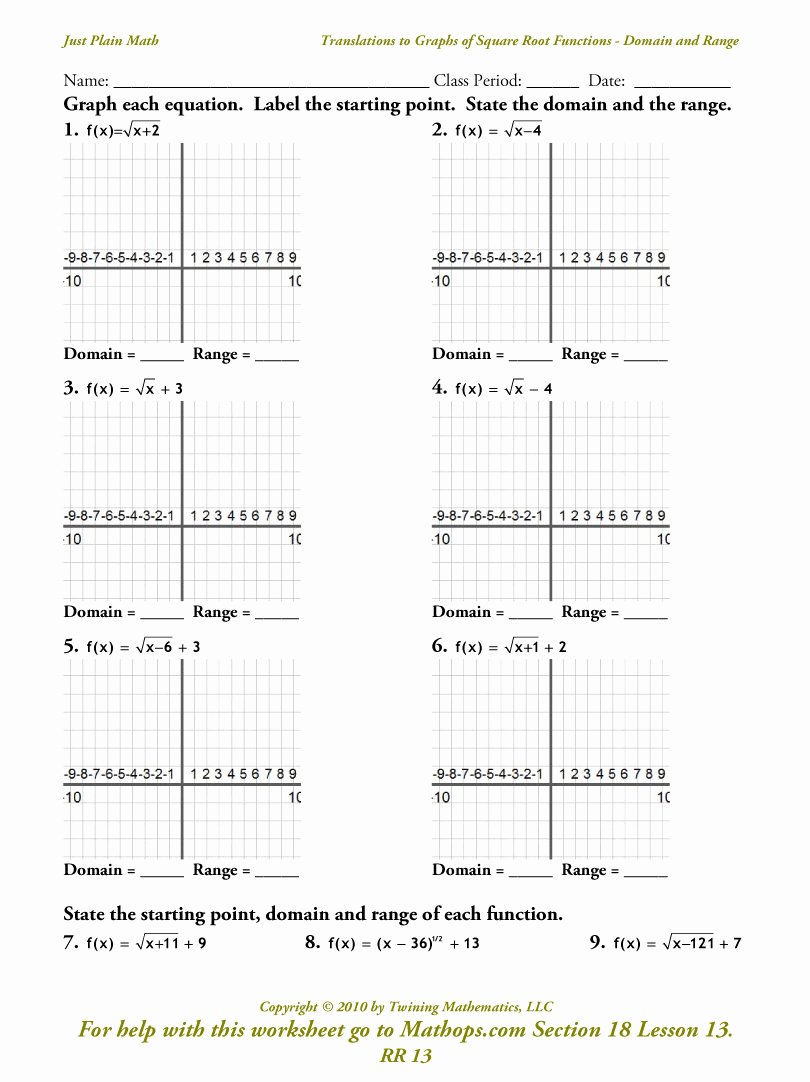
Understanding Rational Functions and Their Graphs
Rational functions are an essential part of algebra and are used to model a wide range of real-world phenomena. A rational function is a ratio of two polynomials, where the denominator is not equal to zero. Graphing rational functions can be challenging, but with the right techniques and strategies, you can become proficient in graphing these functions.
Key Features of Rational Functions
Before we dive into graphing rational functions, let’s review some key features of these functions:
- Domain: The set of all input values (x-values) for which the function is defined. The domain of a rational function excludes values that make the denominator equal to zero.
- Range: The set of all possible output values (y-values) of the function.
- Vertical Asymptotes: Vertical lines that the graph approaches but never touches. These occur when the denominator is equal to zero.
- Horizontal Asymptotes: Horizontal lines that the graph approaches as x goes to positive or negative infinity.
- Holes: Points on the graph where the function is undefined, but the graph is continuous.
Graphing Rational Functions
To graph a rational function, follow these steps:
- Find the domain: Identify values of x that make the denominator equal to zero and exclude them from the domain.
- Find the vertical asymptotes: Set the denominator equal to zero and solve for x. These values are the vertical asymptotes.
- Find the horizontal asymptote: Compare the degrees of the numerator and denominator. If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote is y = 0. If the degrees are equal, the horizontal asymptote is the ratio of the leading coefficients.
- Find the holes: Set the numerator and denominator equal to zero and solve for x. If there are common factors, there may be holes in the graph.
- Graph the function: Use the information gathered to sketch the graph.
Example 1: Graphing a Simple Rational Function
Let’s graph the rational function:
f(x) = 1 / (x - 2)

| x | f(x) |
|---|---|
| 0 | 1/2 |
| 1 | 1 |
| 2 | undefined |
| 3 | 1 |
| 4 | 1/2 |
From the table, we can see that the function is undefined at x = 2, which is a vertical asymptote. The graph approaches the horizontal asymptote y = 0 as x goes to positive or negative infinity.
📝 Note: The graph has a hole at x = 2, since the function is undefined at this point.
Example 2: Graphing a Rational Function with a Horizontal Asymptote
Let’s graph the rational function:
f(x) = (2x + 1) / (x + 1)
| x | f(x) |
|---|---|
| -2 | 3 |
| -1 | 1 |
| 0 | 1 |
| 1 | 3 |
From the table, we can see that the graph approaches the horizontal asymptote y = 2 as x goes to positive or negative infinity.
📝 Note: The graph has a vertical asymptote at x = -1, since the denominator is equal to zero at this point.
Conclusion
Graphing rational functions requires attention to detail and a solid understanding of the key features of these functions. By following the steps outlined in this guide, you can become proficient in graphing rational functions and develop a deeper understanding of their behavior.
What is the domain of a rational function?
+The domain of a rational function is the set of all input values (x-values) for which the function is defined. The domain excludes values that make the denominator equal to zero.
How do I find the vertical asymptotes of a rational function?
+To find the vertical asymptotes, set the denominator equal to zero and solve for x. These values are the vertical asymptotes.
What is a hole in a rational function?
+A hole is a point on the graph where the function is undefined, but the graph is continuous. Holes occur when there are common factors in the numerator and denominator.
Related Terms:
- Desmos
- GeoGebra
- Symbolab
- Photomath
- Microsoft Math
- Wolfram Alpha