5 Ways to Master Grade 4 Fractions
Understanding the Basics of Fractions
As students progress to Grade 4, fractions become an essential part of their math curriculum. Fractions are a way to represent part of a whole, and mastering them can be challenging for some students. However, with practice and the right strategies, students can overcome any obstacles and become proficient in fractions. In this article, we will explore five ways to master Grade 4 fractions.
1. Visualizing Fractions with Real-Life Objects
Using real-life objects is an effective way to help students visualize fractions. You can use everyday objects such as pizzas, cakes, or even fruits to demonstrate fractions. For example, if you have a pizza that is divided into 8 slices, and 2 of them are eaten, you can represent this as a fraction: 2⁄8. This visual representation helps students understand the concept of fractions and how they can be used to represent part of a whole.
📝 Note: Encourage students to draw pictures or diagrams to represent fractions. This will help them develop their problem-solving skills and build confidence in working with fractions.
2. Simplifying Fractions with Greatest Common Divisors
Simplifying fractions is an important skill that students need to master. To simplify a fraction, students need to find the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both numbers without leaving a remainder. For example, if you have the fraction 6⁄8, the GCD of 6 and 8 is 2. Dividing both numbers by 2 gives you the simplified fraction: 3⁄4.

| Fraction | GCD | Simplified Fraction |
|---|---|---|
| 6/8 | 2 | 3/4 |
| 4/6 | 2 | 2/3 |
| 8/10 | 2 | 4/5 |
3. Adding and Subtracting Fractions with Like Denominators
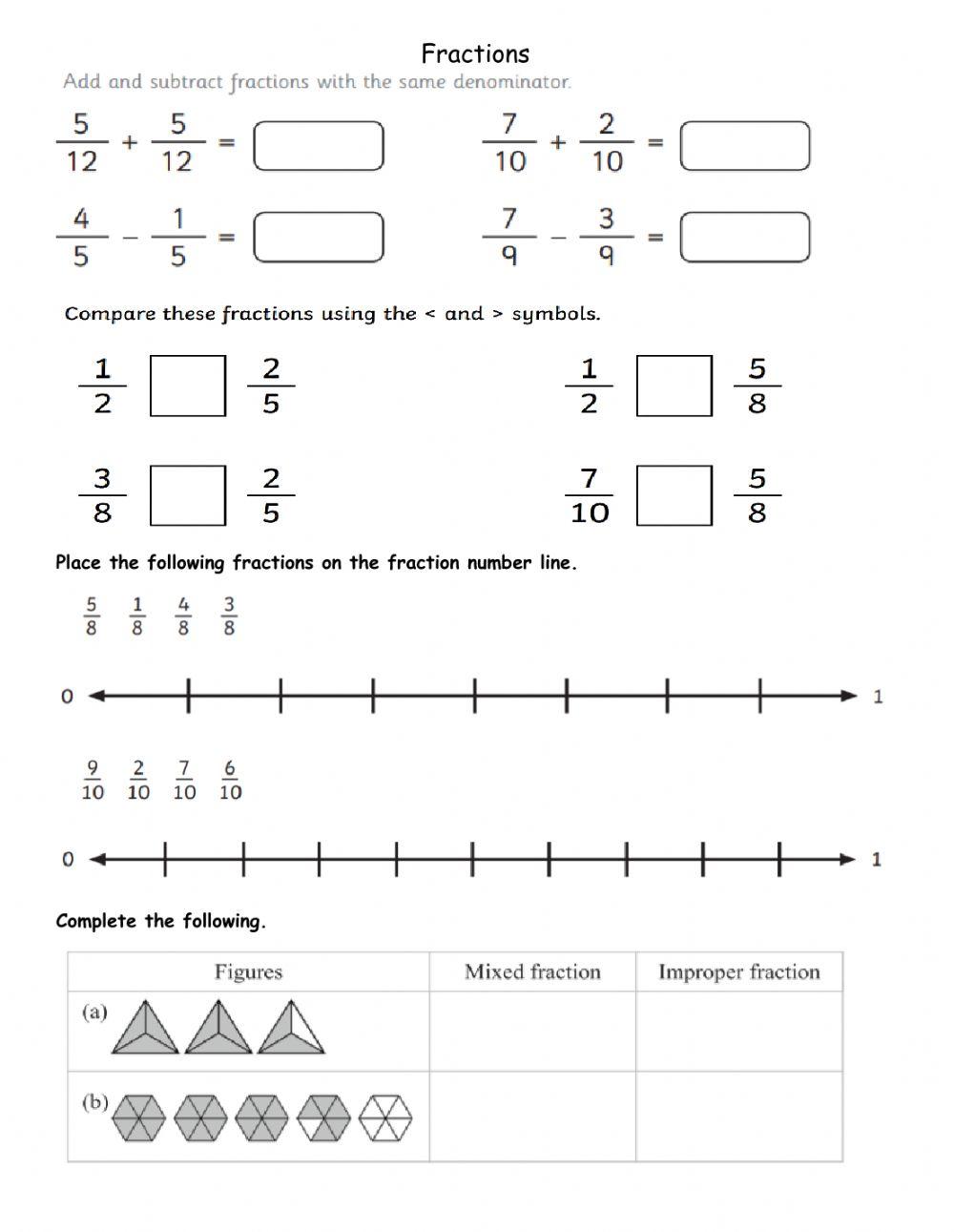
Adding and subtracting fractions with like denominators is a fundamental skill that students need to master. When adding or subtracting fractions with like denominators, students need to add or subtract the numerators and keep the denominator the same. For example, if you have the fractions 1⁄4 and 2⁄4, you can add them by adding the numerators: 1 + 2 = 3. The result is 3⁄4.
📝 Note: Emphasize the importance of having like denominators when adding or subtracting fractions. This will help students avoid common mistakes and build confidence in working with fractions.
4. Comparing Fractions with Different Denominators
Comparing fractions with different denominators can be challenging, but there are strategies that can help. One way to compare fractions is to find the least common multiple (LCM) of the denominators. The LCM is the smallest number that both denominators can divide into evenly. For example, if you have the fractions 1⁄4 and 1⁄6, the LCM of 4 and 6 is 12. You can then convert both fractions to have a denominator of 12: 3⁄12 and 2⁄12. Comparing the numerators, you can see that 3⁄12 is greater than 2⁄12.
5. Applying Fractions to Real-World Problems
Applying fractions to real-world problems is an essential part of mastering Grade 4 fractions. Fractions are used in various real-world applications, such as cooking, building, and finance. Encourage students to use fractions to solve problems that involve part of a whole. For example, if a recipe calls for 3⁄4 cup of sugar, students can practice measuring out the correct amount.
In conclusion, mastering Grade 4 fractions requires practice, patience, and persistence. By using real-life objects, simplifying fractions, adding and subtracting fractions with like denominators, comparing fractions with different denominators, and applying fractions to real-world problems, students can build a strong foundation in fractions and develop problem-solving skills that will benefit them in the long run.
What is the greatest common divisor (GCD) of two numbers?
+The GCD is the largest number that divides both numbers without leaving a remainder.
How do you simplify a fraction?
+To simplify a fraction, divide both the numerator and denominator by the greatest common divisor (GCD).
What is the least common multiple (LCM) of two numbers?
+The LCM is the smallest number that both denominators can divide into evenly.
Related Terms:
- Fraction Worksheet Grade 5
- Fraction to decimal Grade 4
- Fraction Worksheet Grade 3
- Worksheet multiplication grade 4
- Basic fraction worksheets pdf