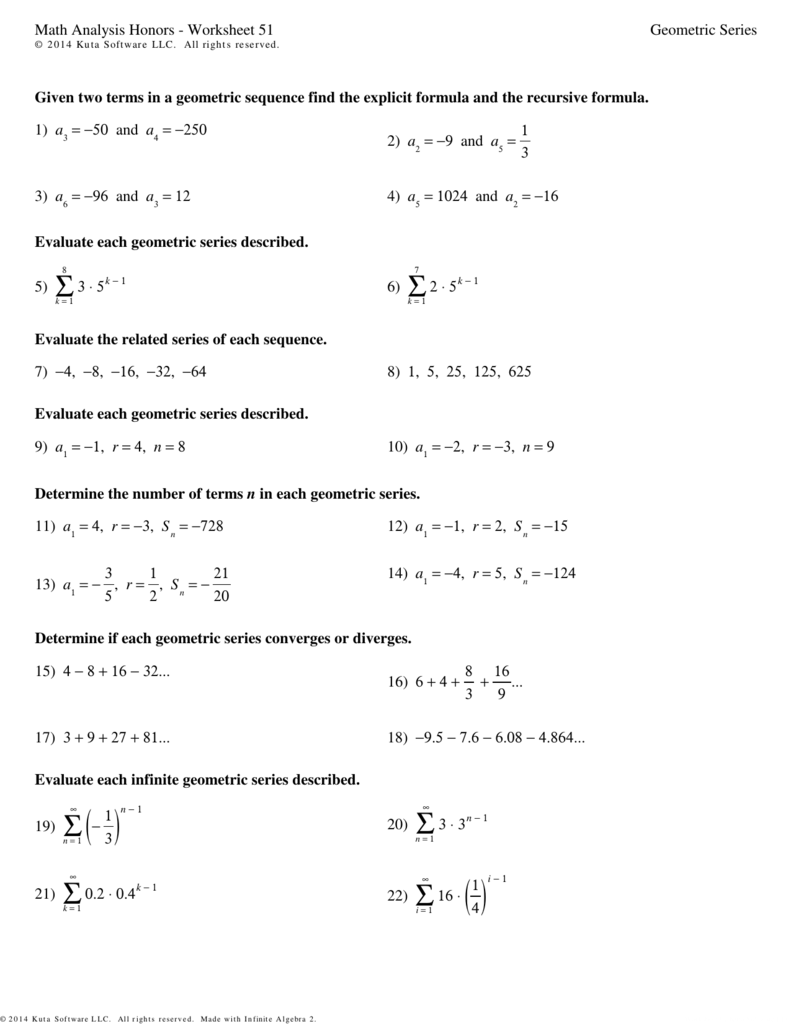
Geometric Mean Worksheet With Answers for Easy Practice
Understanding Geometric Mean: A Comprehensive Guide
Geometric mean is a mathematical concept that represents the average of a set of numbers in a multiplicative way. It’s an essential tool for data analysis, finance, and other fields where percentages and growth rates are crucial. In this post, we’ll delve into the world of geometric mean, provide a worksheet with answers for practice, and explore its real-world applications.
What is Geometric Mean?
The geometric mean is a type of average that indicates the central tendency of a set of numbers by multiplying them together and taking the nth root of the product. The formula for geometric mean is:
Geometric Mean = (Product of all numbers)^(1/n)
where n is the number of values in the dataset.
How to Calculate Geometric Mean
Calculating geometric mean involves the following steps:
- Multiply all the numbers in the dataset together.
- Take the nth root of the product, where n is the number of values.
For example, let’s calculate the geometric mean of the numbers 2, 4, and 6:
- Multiply the numbers: 2 × 4 × 6 = 48
- Take the cube root (since there are 3 numbers): ∛48 ≈ 3.62
Therefore, the geometric mean of 2, 4, and 6 is approximately 3.62.
Geometric Mean Worksheet with Answers
Practice makes perfect! Here’s a worksheet with 10 questions to help you reinforce your understanding of geometric mean:
Worksheet:
- Calculate the geometric mean of 3, 5, and 7.
- Find the geometric mean of 2, 6, and 12.
- What is the geometric mean of 1, 3, and 9?
- Calculate the geometric mean of 4, 8, and 16.
- Find the geometric mean of 5, 10, and 20.
- What is the geometric mean of 2, 8, and 32?
- Calculate the geometric mean of 3, 9, and 27.
- Find the geometric mean of 1, 2, and 4.
- What is the geometric mean of 6, 12, and 24?
- Calculate the geometric mean of 8, 16, and 32.
Answers:
- ∛(3 × 5 × 7) ≈ 4.93
- ∛(2 × 6 × 12) ≈ 4.62
- ∛(1 × 3 × 9) ≈ 3
- ∛(4 × 8 × 16) ≈ 8
- ∛(5 × 10 × 20) ≈ 10
- ∛(2 × 8 × 32) ≈ 8
- ∛(3 × 9 × 27) ≈ 9
- ∛(1 × 2 × 4) ≈ 2
- ∛(6 × 12 × 24) ≈ 12
- ∛(8 × 16 × 32) ≈ 16
📝 Note: You can use a calculator to find the cube root (or nth root) of the product.
Real-World Applications of Geometric Mean
Geometric mean has numerous applications in finance, economics, and other fields, including:
- Investment returns: Geometric mean is used to calculate the average annual return of an investment portfolio.
- Growth rates: Geometric mean is used to calculate the average growth rate of a company’s revenue or earnings.
- Interest rates: Geometric mean is used to calculate the average interest rate of a loan or investment.
- Data analysis: Geometric mean is used to analyze and compare data sets with varying scales or units.
Benefits of Using Geometric Mean
Geometric mean offers several benefits over other types of averages, including:
- More accurate representation: Geometric mean provides a more accurate representation of the data, especially when dealing with percentages and growth rates.
- Reduced bias: Geometric mean is less sensitive to extreme values and outliers, reducing bias in the calculation.
- Improved forecasting: Geometric mean can be used to make more accurate forecasts and predictions, especially in finance and economics.
What is the difference between arithmetic mean and geometric mean?
+Arithmetic mean is the sum of all values divided by the number of values, while geometric mean is the nth root of the product of all values.
Why is geometric mean used in finance and economics?
+Geometric mean is used in finance and economics to calculate average returns, growth rates, and interest rates, as it provides a more accurate representation of the data.
How is geometric mean calculated?
+Geometric mean is calculated by multiplying all the numbers in the dataset together and taking the nth root of the product, where n is the number of values.
In conclusion, geometric mean is a powerful tool for data analysis and decision-making. By understanding how to calculate and apply geometric mean, you can gain valuable insights into finance, economics, and other fields. Remember to practice with the worksheet provided to reinforce your knowledge and become proficient in using geometric mean.