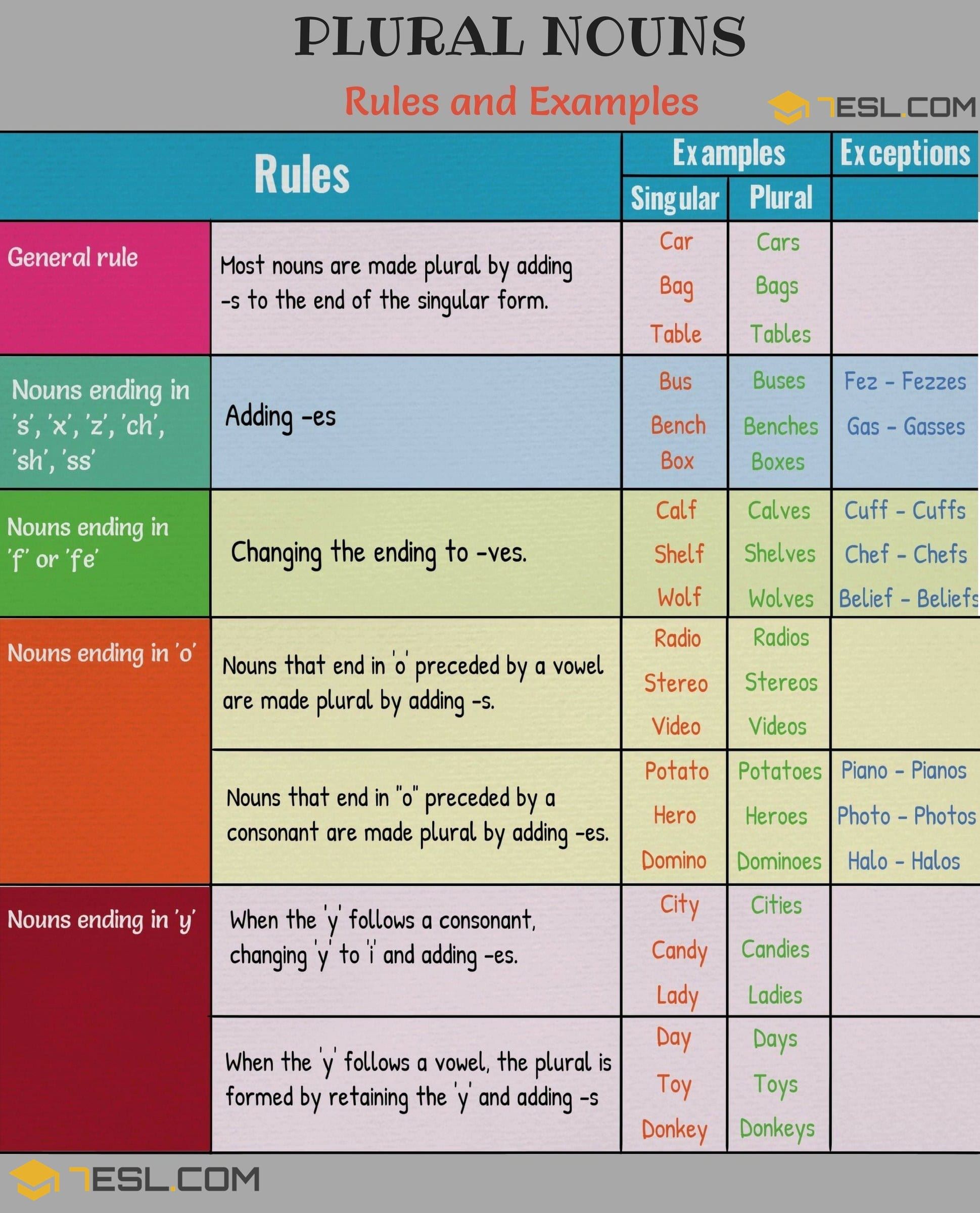
Simplify Polynomials with GCF Factoring Worksheets

Simplifying polynomials with Greatest Common Factor (GCF) factoring is a fundamental concept in algebra that helps students understand the properties of polynomials and how to manipulate them. In this article, we will explore the concept of GCF factoring and provide worksheets to help students practice and master this skill.
What is GCF Factoring?
GCF factoring is a method of factoring polynomials that involves finding the greatest common factor (GCF) of two or more terms. The GCF is the largest factor that divides all the terms of the polynomial without leaving a remainder. By factoring out the GCF, students can simplify polynomials and make them easier to work with.
How to Factor Polynomials with GCF
Factoring polynomials with GCF involves the following steps:
- Identify the terms of the polynomial.
- Find the greatest common factor (GCF) of the terms.
- Factor out the GCF from each term.
- Write the factored form of the polynomial.
For example, consider the polynomial 6x + 12. To factor this polynomial, we need to find the GCF of 6x and 12. The GCF is 6, so we can factor out 6 from each term:
6x + 12 = 6(x + 2)
Types of Polynomials that Can be Factored with GCF
Not all polynomials can be factored with GCF. However, the following types of polynomials can be factored using this method:
- Binomials: polynomials with two terms, such as 2x + 3
- Trinomials: polynomials with three terms, such as x^2 + 4x + 4
- Quadratics: polynomials with four terms, such as x^2 + 2x + 3x + 6
Worksheets for Practicing GCF Factoring
To help students practice and master GCF factoring, we provide the following worksheets:
Worksheet 1: Factoring Binomials with GCF

| Polynomial | Factored Form |
|---|---|
| 2x + 4 | |
| 3x - 6 | |
| 4x + 8 |
Worksheet 2: Factoring Trinomials with GCF
| Polynomial | Factored Form |
|---|---|
| x^2 + 4x + 4 | |
| 2x^2 + 6x + 4 | |
| 3x^2 + 9x + 6 |
Worksheet 3: Factoring Quadratics with GCF
| Polynomial | Factored Form |
|---|---|
| x^2 + 2x + 3x + 6 | |
| 2x^2 + 4x + 6x + 12 | |
| 3x^2 + 6x + 9x + 18 |
Tips and Tricks for Factoring Polynomials with GCF
- Always check if the polynomial can be factored with GCF before trying other factoring methods.
- Look for common factors among the terms of the polynomial.
- Use the distributive property to factor out the GCF.
📝 Note: Factoring polynomials with GCF is a fundamental skill in algebra. Practice regularly to become proficient in this skill.
Conclusion
Simplifying polynomials with GCF factoring is an essential skill in algebra. By understanding how to factor polynomials with GCF, students can simplify complex polynomials and make them easier to work with. With the worksheets provided, students can practice and master this skill.
What is the greatest common factor (GCF) of two or more terms?
+The GCF is the largest factor that divides all the terms of the polynomial without leaving a remainder.
How do I factor a polynomial with GCF?
+To factor a polynomial with GCF, identify the terms of the polynomial, find the GCF, factor out the GCF from each term, and write the factored form of the polynomial.
What types of polynomials can be factored with GCF?
+Binomials, trinomials, and quadratics can be factored with GCF.
Related Terms:
- GCF Factoring Worksheet pdf
- Factoring Binomials Worksheet pdf
- Factoring Worksheet with Answers pdf
- GCF Factoring Worksheet Kuta