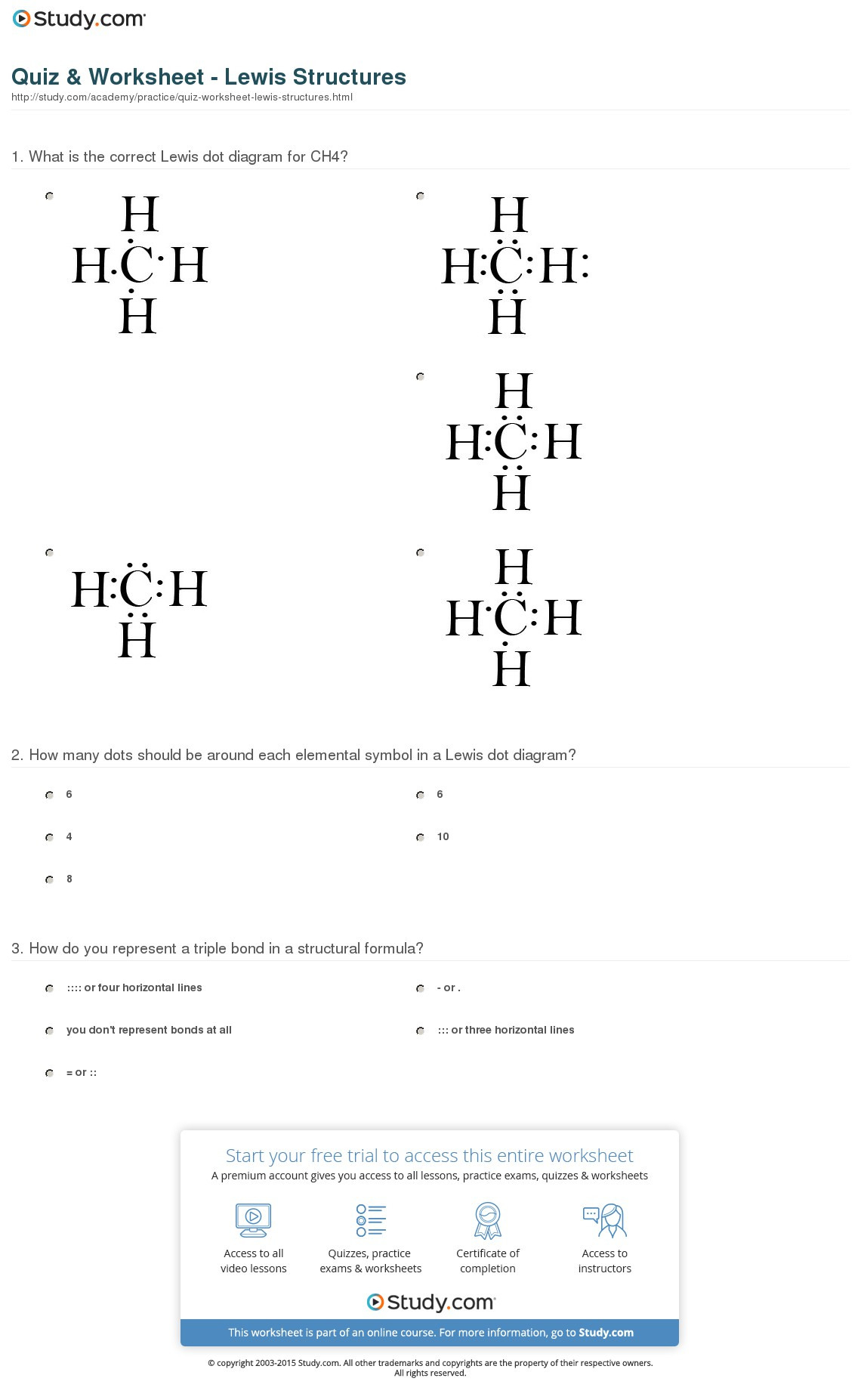
5 Free Exponent Worksheets to Try Today

Mastering Exponents: 5 Free Worksheets to Try Today
Exponents are a fundamental concept in mathematics, and mastering them is crucial for success in various math disciplines, including algebra, geometry, and calculus. To help students and educators, we’ve compiled a list of 5 free exponent worksheets that can be used to practice and reinforce exponent skills. These worksheets cover various aspects of exponents, from basic exponent rules to more advanced concepts.
What are Exponents?
Before diving into the worksheets, let’s briefly review what exponents are. An exponent is a small number that is raised to a power, indicating how many times the base number should be multiplied by itself. For example, in the expression 2^3, the base is 2, and the exponent is 3. This expression can be evaluated as 2 × 2 × 2 = 8.
Free Exponent Worksheets
Here are 5 free exponent worksheets that you can try today:
Worksheet 1: Basic Exponent Rules
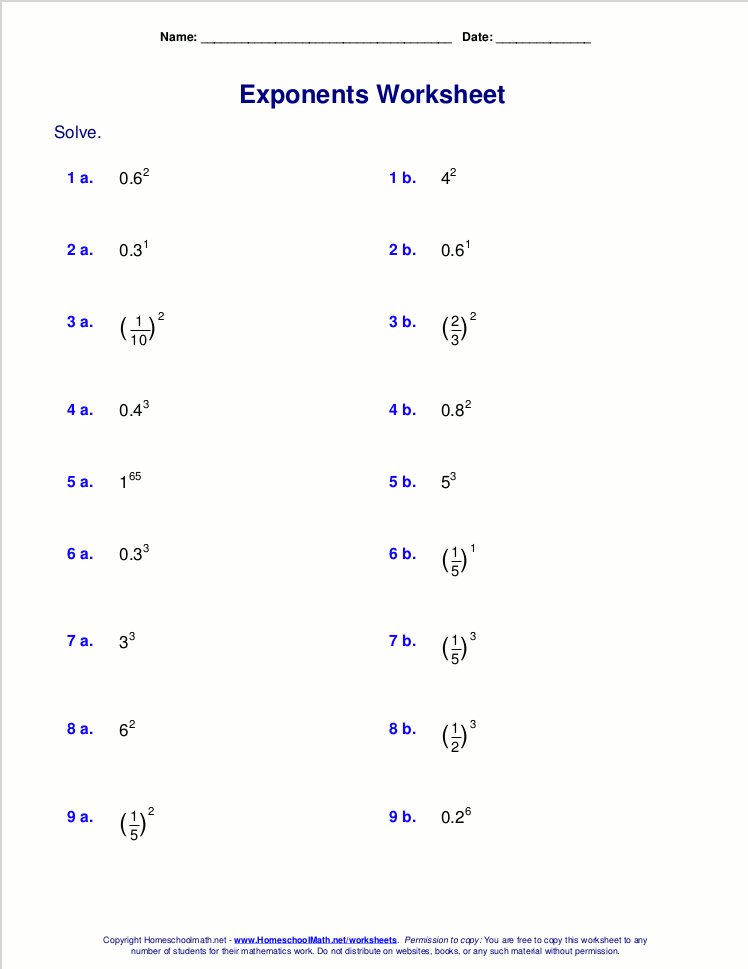
| Expression | Evaluation |
|---|---|
| 2^1 | 2 |
| 2^2 | 4 |
| 2^3 | 8 |
| 3^1 | 3 |
| 3^2 | 9 |
| 3^3 | 27 |
📝 Note: This worksheet focuses on basic exponent rules, where the exponent is a small positive integer. Students should be able to evaluate each expression by multiplying the base number by itself the specified number of times.
Worksheet 2: Exponent Properties
| Expression | Simplified Expression |
|---|---|
| (2^2)^3 | 2^(2×3) |
| (3^3)^2 | 3^(3×2) |
| 2^2 × 2^3 | 2^(2+3) |
| 3^3 ÷ 3^2 | 3^(3-2) |
💡 Note: This worksheet covers exponent properties, including the power of a power, product of powers, and quotient of powers. Students should be able to simplify each expression using the relevant exponent property.
Worksheet 3: Negative Exponents
| Expression | Evaluation |
|---|---|
| 2^(-1) | 1⁄2 |
| 2^(-2) | 1⁄4 |
| 3^(-1) | 1⁄3 |
| 3^(-2) | 1⁄9 |
| 2^(-3) | 1⁄8 |
📊 Note: This worksheet introduces negative exponents, which represent reciprocals of the base number. Students should be able to evaluate each expression by taking the reciprocal of the base number.
Worksheet 4: Zero Exponents
| Expression | Evaluation |
|---|---|
| 2^0 | 1 |
| 3^0 | 1 |
| 4^0 | 1 |
| 0^2 | 0 |
| 0^3 | 0 |
🤔 Note: This worksheet covers zero exponents, which are defined as 1. Students should be able to evaluate each expression, recognizing that any non-zero base raised to the power of 0 is equal to 1.
Worksheet 5: Advanced Exponents
| Expression | Simplified Expression |
|---|---|
| (2^2)^3 × 2^4 | 2^(2×3+4) |
| (3^3)^2 ÷ 3^2 | 3^(3×2-2) |
| 2^(-2) × 2^3 | 2^(-2+3) |
| 3^(-1) ÷ 3^(-2) | 3^(-1+2) |
📈 Note: This worksheet challenges students to apply their knowledge of exponents to more complex expressions, involving multiple exponent properties and negative exponents.
Conclusion
Mastering exponents is essential for success in mathematics, and these 5 free worksheets provide a great starting point for practice. By working through these worksheets, students will develop a deeper understanding of exponent rules, properties, and applications. Whether you’re a student, teacher, or tutor, these worksheets are an excellent resource to help you reinforce your knowledge of exponents.
What is the difference between a base and an exponent?
+The base is the number being raised to a power, while the exponent is the small number that indicates how many times the base should be multiplied by itself.
How do I evaluate an expression with a negative exponent?
+To evaluate an expression with a negative exponent, take the reciprocal of the base number. For example, 2^(-1) = 1⁄2.
What is the product of powers property?
+The product of powers property states that when multiplying two powers with the same base, add the exponents. For example, 2^2 × 2^3 = 2^(2+3) = 2^5.