Mastering Free-Body Diagrams: Answers and Worksheet Solutions
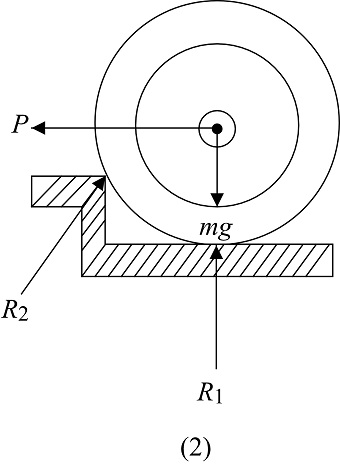
Understanding Free-Body Diagrams
Free-body diagrams are a powerful tool used in physics to visualize and analyze the forces acting on an object. They are a crucial part of solving problems in mechanics, and are used to identify the net force acting on an object, which is essential for determining its motion. In this article, we will delve into the world of free-body diagrams, exploring their importance, how to draw them, and providing solutions to common problems.
The Importance of Free-Body Diagrams
Free-body diagrams are essential in physics because they allow us to:
- Identify the forces acting on an object: By drawing a free-body diagram, we can clearly see all the forces acting on an object, including the direction and magnitude of each force.
- Determine the net force: By adding up all the forces acting on an object, we can determine the net force, which is the force that actually causes the object to accelerate.
- Solve problems in mechanics: Free-body diagrams are used to solve problems in mechanics, such as finding the acceleration of an object, the force required to move an object, and the tension in a rope.
How to Draw a Free-Body Diagram
Drawing a free-body diagram is a straightforward process that involves the following steps:
- Choose a reference frame: Choose a reference frame that is convenient for the problem. This is usually a Cartesian coordinate system with x, y, and z axes.
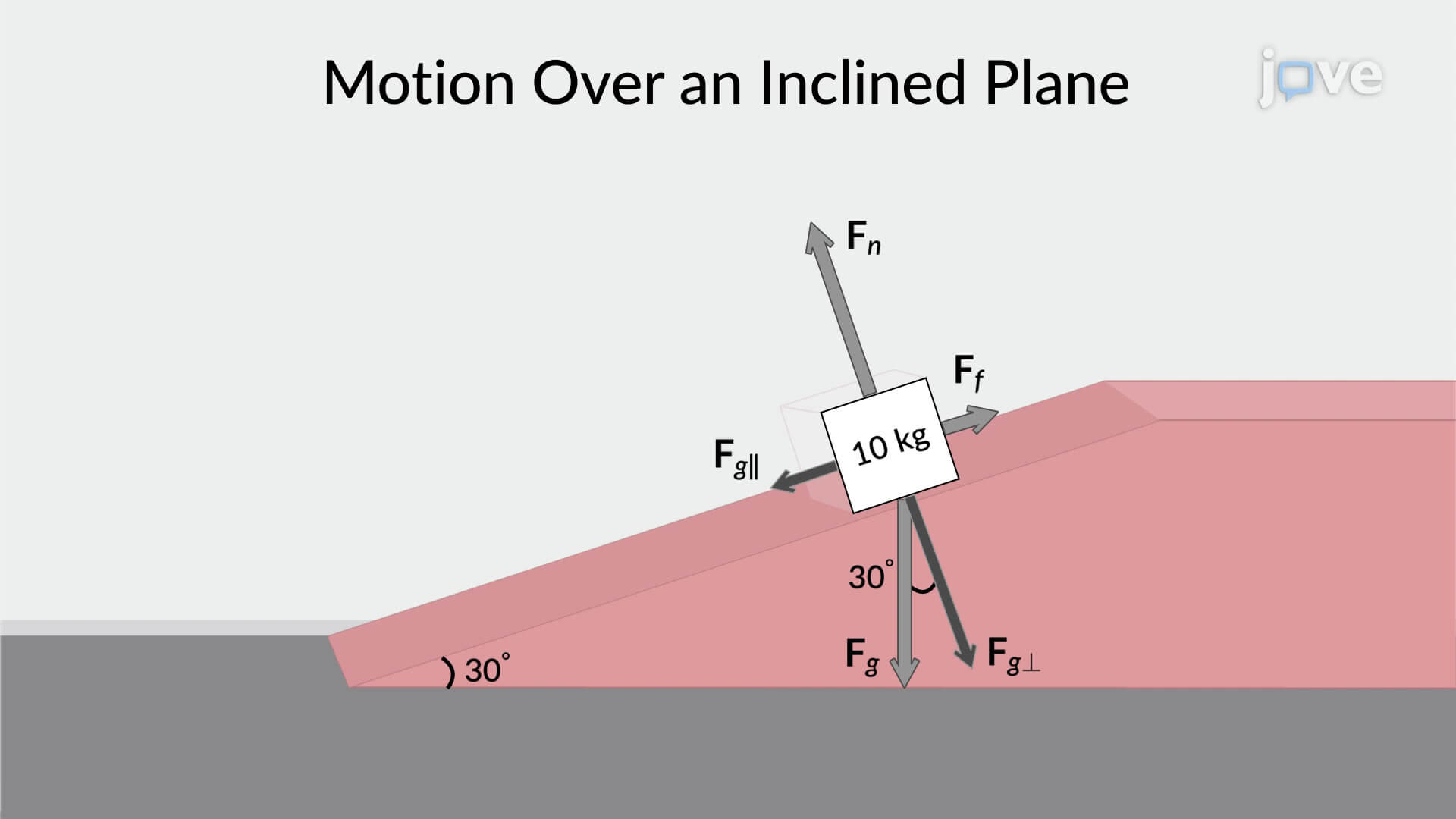
- Identify the forces: Identify all the forces acting on the object, including gravity, normal force, friction, tension, and any other external forces.
- Draw the forces: Draw each force as an arrow, making sure to include the direction and magnitude of each force.
- Label the forces: Label each force with its corresponding symbol, such as Fg for gravity, Fn for normal force, and Ff for friction.
💡 Note: Make sure to include all the forces acting on the object, even if they are not explicitly mentioned in the problem.
Common Forces in Free-Body Diagrams
There are several common forces that appear in free-body diagrams, including:
- Gravity (Fg): The force of gravity acting on an object, which is always downward.
- Normal force (Fn): The force exerted by a surface on an object, which is always perpendicular to the surface.
- Friction (Ff): The force that opposes motion between two surfaces, which can be either static or kinetic.
- Tension (Ft): The force exerted by a rope or string on an object, which is always along the length of the rope.
Solutions to Common Problems
Here are solutions to some common problems involving free-body diagrams:
Problem 1: A block of mass 2 kg is placed on a frictionless surface. What is the net force acting on the block?
Solution: Since the surface is frictionless, there is no friction force acting on the block. The only force acting on the block is gravity, which is downward. Therefore, the net force acting on the block is Fg = 2 kg x 9.8 m/s^2 = 19.6 N downward.
Problem 2: A rope is attached to a block of mass 5 kg. The rope is pulled with a force of 20 N, and the block is accelerated at 2 m/s^2. What is the tension in the rope?
Solution: Since the block is accelerated, there must be a net force acting on it. The net force is the tension in the rope minus the force of gravity. We can calculate the net force as Fnet = ma = 5 kg x 2 m/s^2 = 10 N. Since the tension in the rope is upward, and the force of gravity is downward, we can write Fnet = Ft - Fg. Solving for Ft, we get Ft = Fnet + Fg = 10 N + 49 N = 59 N.
Worksheet Solutions
Here are solutions to some common worksheet problems involving free-body diagrams:
| Problem | Solution |
|---|---|
| A block of mass 3 kg is placed on a surface with a coefficient of friction of 0.2. What is the net force acting on the block? | Fnet = Ff - Fg = 0.2 x 3 kg x 9.8 m/s^2 - 3 kg x 9.8 m/s^2 = 1.96 N - 29.4 N = -27.44 N |
| A rope is attached to a block of mass 10 kg. The rope is pulled with a force of 50 N, and the block is accelerated at 5 m/s^2. What is the tension in the rope? | Fnet = ma = 10 kg x 5 m/s^2 = 50 N. Fnet = Ft - Fg, so Ft = Fnet + Fg = 50 N + 98 N = 148 N |
📝 Note: These solutions are meant to serve as examples only. Make sure to check your work and use the correct units.
What is the purpose of a free-body diagram?
+A free-body diagram is used to identify the forces acting on an object, determine the net force, and solve problems in mechanics.
How do I draw a free-body diagram?
+To draw a free-body diagram, choose a reference frame, identify the forces, draw each force as an arrow, and label each force with its corresponding symbol.
What are some common forces that appear in free-body diagrams?
+Some common forces that appear in free-body diagrams include gravity, normal force, friction, and tension.
In conclusion, free-body diagrams are a powerful tool used in physics to visualize and analyze the forces acting on an object. By understanding how to draw a free-body diagram and identifying the common forces that appear, we can solve problems in mechanics and gain a deeper understanding of the physical world.