Fractions, Decimals, and Percents Worksheets for Math Mastery
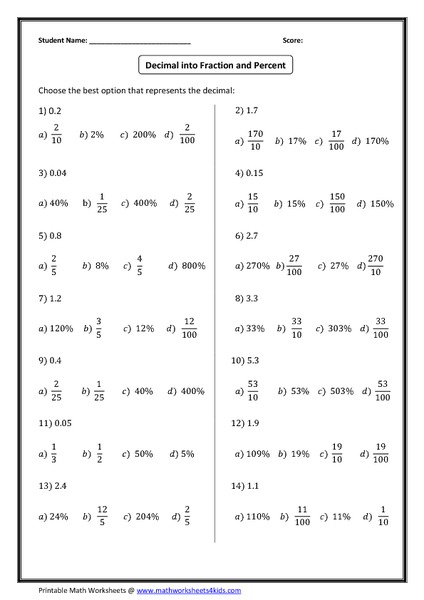
Mastering Fractions, Decimals, and Percents: A Comprehensive Guide
Fractions, decimals, and percents are fundamental concepts in mathematics that often pose a challenge for students. Understanding these concepts is crucial for success in various mathematical operations, problem-solving, and real-world applications. In this article, we will delve into the world of fractions, decimals, and percents, exploring their definitions, conversions, and practical applications. We will also provide you with valuable resources, including worksheets, to help you master these essential math concepts.
Understanding Fractions
A fraction is a way to express a part of a whole as a ratio of two numbers. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator tells us how many equal parts we have, while the denominator tells us how many parts the whole is divided into.
Key Concepts:
- Simplifying Fractions: To simplify a fraction, we need to find the greatest common divisor (GCD) of the numerator and the denominator and divide both numbers by the GCD.
- Equivalent Fractions: Equivalent fractions are fractions that have the same value, but different numerators and denominators.
- Comparing Fractions: To compare fractions, we need to find a common denominator and then compare the numerators.
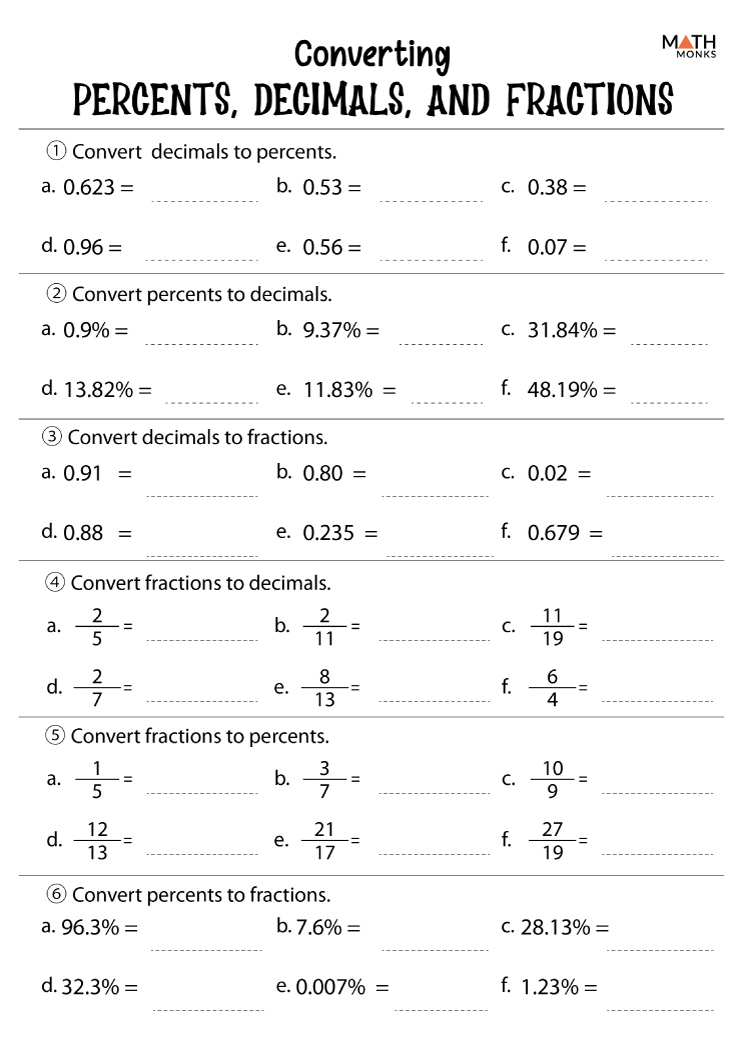
Converting Fractions to Decimals and Percents
Converting fractions to decimals and percents is a crucial skill in mathematics. To convert a fraction to a decimal, we divide the numerator by the denominator. To convert a fraction to a percent, we divide the numerator by the denominator and multiply by 100.
Conversion Table:
| Fraction | Decimal | Percent |
|---|---|---|
| 1/2 | 0.5 | 50% |
| 1/4 | 0.25 | 25% |
| 3/4 | 0.75 | 75% |
Understanding Decimals
A decimal is a way to express a number using a point (.) to separate the whole part from the fractional part. Decimals can be converted to fractions and percents using the same techniques as before.
Key Concepts:
- Rounding Decimals: To round a decimal, we look at the digit to the right of the desired place value and decide whether to round up or down.
- Comparing Decimals: To compare decimals, we need to compare the digits to the right of the decimal point.
- Adding and Subtracting Decimals: To add or subtract decimals, we need to align the decimal points and perform the operation as usual.
Understanding Percents
A percent is a way to express a number as a fraction of 100. Percents can be converted to fractions and decimals using the same techniques as before.
Key Concepts:
- Finding a Percent of a Number: To find a percent of a number, we multiply the number by the percent as a decimal.
- Increasing and Decreasing Percents: To increase or decrease a number by a percent, we multiply the number by the percent as a decimal and add or subtract the result.
- Comparing Percents: To compare percents, we need to compare the decimal equivalents.
Worksheets for Math Mastery
To help you master fractions, decimals, and percents, we have created a set of worksheets that cover various topics, from simplifying fractions to finding percents of numbers.
Worksheets:
- Simplifying Fractions: Simplify fractions to their lowest terms.
- Converting Fractions to Decimals and Percents: Convert fractions to decimals and percents using the conversion table.
- Comparing Fractions: Compare fractions using equivalent fractions and common denominators.
- Rounding Decimals: Round decimals to the nearest tenth, hundredth, or thousandth.
- Finding a Percent of a Number: Find a percent of a number using multiplication.
- Increasing and Decreasing Percents: Increase or decrease a number by a percent using multiplication.
📝 Note: These worksheets are designed to help you practice and reinforce your understanding of fractions, decimals, and percents. Make sure to complete each worksheet carefully and check your answers to ensure accuracy.
Conclusion
Mastering fractions, decimals, and percents is essential for success in mathematics and real-world applications. By understanding the definitions, conversions, and practical applications of these concepts, you will be well-equipped to tackle various math problems and challenges. Remember to practice regularly using the worksheets provided, and don’t hesitate to seek help when needed. With persistence and dedication, you can become a math master and achieve your goals.
What is the difference between a fraction and a decimal?
+A fraction is a way to express a part of a whole as a ratio of two numbers, while a decimal is a way to express a number using a point (.) to separate the whole part from the fractional part.
How do I convert a fraction to a decimal?
+To convert a fraction to a decimal, divide the numerator by the denominator.
What is the concept of equivalent fractions?
+Equivalent fractions are fractions that have the same value, but different numerators and denominators.