5 Ways to Master Fractions as Division

Understanding Fractions as Division
Fractions are a fundamental concept in mathematics, and mastering them is crucial for success in various mathematical operations. One way to think about fractions is as a division operation. In this blog post, we will explore five ways to master fractions as division, making it easier to understand and work with fractions.
1. Visualizing Fractions as Division
To start, it’s essential to visualize fractions as a division operation. Imagine you have a pizza that’s cut into 8 slices, and you eat 2 of them. You can represent this as a fraction, 2⁄8, which can also be thought of as 2 ÷ 8. This visualization helps you understand that fractions are simply a way of showing a part of a whole.
🍕 Note: When visualizing fractions, use real-life examples like pizzas, cakes, or toys to make it more relatable.
2. Simplifying Fractions as Division
Simplifying fractions is an essential skill to master. To simplify a fraction, you need to find the greatest common divisor (GCD) of the numerator and the denominator. For example, to simplify the fraction 4⁄8, you can divide both the numerator and the denominator by 2, resulting in 2⁄4, which can be further simplified to 1⁄2. This process is similar to dividing both numbers by their GCD.
| Fraction | Simplified Fraction |
|---|---|
| 4/8 | 1/2 |
| 6/12 | 1/2 |
3. Adding and Subtracting Fractions as Division
Adding and subtracting fractions can be challenging, but thinking of them as division operations can make it easier. When adding or subtracting fractions, you need to have the same denominator. For example, to add 1⁄4 and 1⁄4, you can think of it as (1 ÷ 4) + (1 ÷ 4) = 2 ÷ 4 = 1⁄2.
- When adding fractions, add the numerators and keep the denominator the same.
- When subtracting fractions, subtract the numerators and keep the denominator the same.
📝 Note: Make sure to have the same denominator when adding or subtracting fractions.
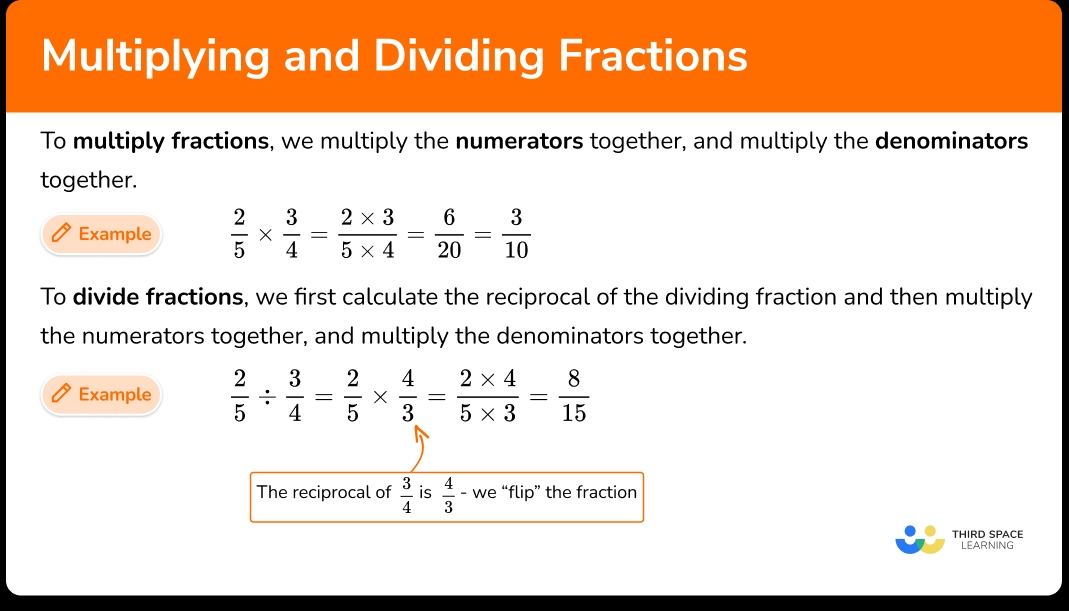
4. Multiplying Fractions as Division
Multiplying fractions can be thought of as multiplying the numerators and denominators separately. For example, to multiply 1⁄2 and 3⁄4, you can think of it as (1 ÷ 2) × (3 ÷ 4) = (1 × 3) ÷ (2 × 4) = 3 ÷ 8 = 3⁄8.
- When multiplying fractions, multiply the numerators and multiply the denominators.
- Simplify the resulting fraction, if possible.
5. Dividing Fractions as Division
Dividing fractions can be thought of as inverting the second fraction and multiplying. For example, to divide 1⁄2 by 3⁄4, you can think of it as (1 ÷ 2) ÷ (3 ÷ 4) = (1 ÷ 2) × (4 ÷ 3) = (1 × 4) ÷ (2 × 3) = 4 ÷ 6 = 2⁄3.
- When dividing fractions, invert the second fraction and multiply.
- Simplify the resulting fraction, if possible.
In conclusion, mastering fractions as division requires practice and patience. By visualizing fractions as division, simplifying fractions, and applying the rules for adding, subtracting, multiplying, and dividing fractions, you can become proficient in working with fractions.
What is the best way to visualize fractions as division?
+Use real-life examples like pizzas, cakes, or toys to visualize fractions as division.
How do you simplify fractions as division?
+To simplify fractions, find the greatest common divisor (GCD) of the numerator and the denominator and divide both numbers by their GCD.
What is the rule for adding and subtracting fractions as division?
+When adding or subtracting fractions, make sure to have the same denominator. Add or subtract the numerators and keep the denominator the same.