5 Essential Fraction Operations to Master
Understanding the Basics of Fraction Operations
Fractions are a fundamental concept in mathematics, and being able to perform operations with them is crucial for success in various mathematical disciplines. In this article, we will explore five essential fraction operations that you need to master in order to tackle more complex mathematical problems.
Addition of Fractions
Adding fractions is one of the most basic operations you can perform with fractions. To add fractions, you need to follow these steps:
- Make sure the denominators are the same. If they are not, find the least common multiple (LCM) of the two denominators.
- Add the numerators (the numbers on top) while keeping the denominator the same.
- Simplify the fraction, if possible.
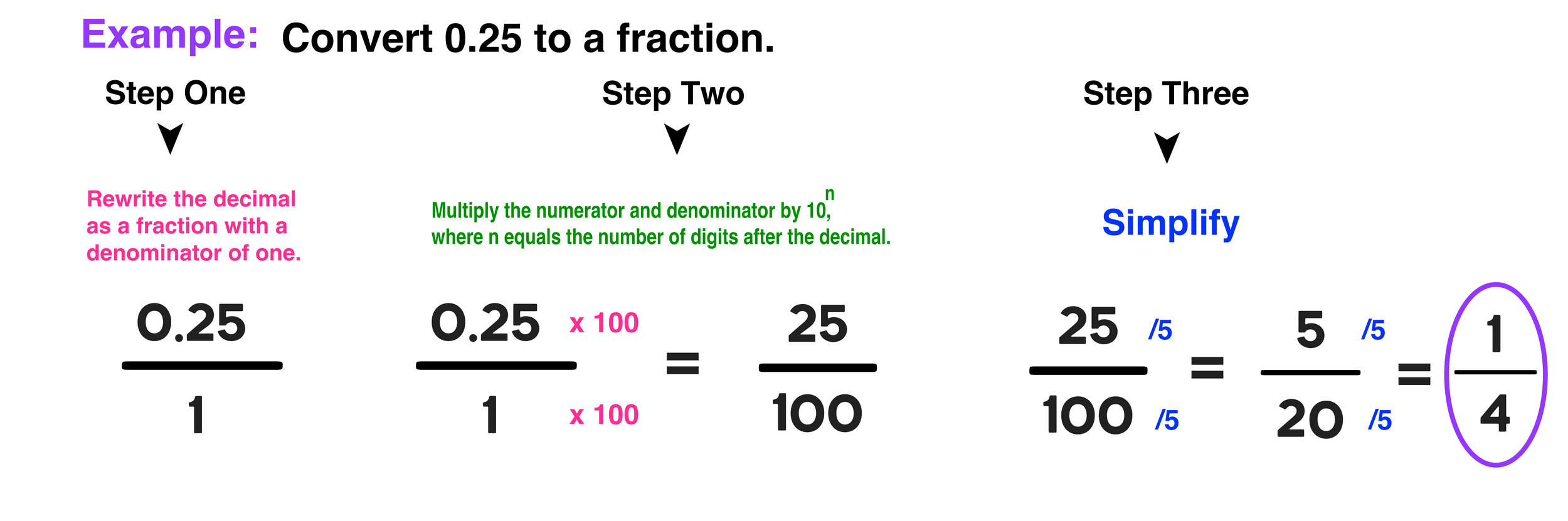
For example, let’s add the fractions 1⁄4 and 1⁄4:
1⁄4 + 1⁄4 = 2⁄4
We can simplify this fraction by dividing both the numerator and denominator by 2:
2⁄4 = 1⁄2
🤔 Note: When adding fractions, make sure to line up the denominators and add the numerators separately.
Subtraction of Fractions
Subtracting fractions is similar to adding fractions, except that you subtract the numerators instead of adding them. To subtract fractions, follow these steps:
- Make sure the denominators are the same. If they are not, find the LCM of the two denominators.
- Subtract the numerators while keeping the denominator the same.
- Simplify the fraction, if possible.
For example, let’s subtract the fractions 3⁄4 and 1⁄4:
3⁄4 - 1⁄4 = 2⁄4
We can simplify this fraction by dividing both the numerator and denominator by 2:
2⁄4 = 1⁄2
🤔 Note: When subtracting fractions, make sure to line up the denominators and subtract the numerators separately.
Multiplication of Fractions
Multiplying fractions is a bit different from adding and subtracting fractions. To multiply fractions, follow these steps:
- Multiply the numerators (the numbers on top) together.
- Multiply the denominators together.
- Simplify the fraction, if possible.
For example, let’s multiply the fractions 1⁄2 and 3⁄4:
(1⁄2) × (3⁄4) = (1 × 3) / (2 × 4) = 3⁄8
🤔 Note: When multiplying fractions, make sure to multiply the numerators and denominators separately.
Division of Fractions
Dividing fractions is similar to multiplying fractions, except that you invert the second fraction (i.e., flip the numerator and denominator) and then multiply. To divide fractions, follow these steps:
- Invert the second fraction (i.e., flip the numerator and denominator).
- Multiply the fractions as usual.
For example, let’s divide the fractions 1⁄2 and 3⁄4:
(1⁄2) ÷ (3⁄4) = (1⁄2) × (4⁄3) = (1 × 4) / (2 × 3) = 4⁄6
We can simplify this fraction by dividing both the numerator and denominator by 2:
4⁄6 = 2⁄3
🤔 Note: When dividing fractions, make sure to invert the second fraction and multiply as usual.
Comparison of Fractions
Comparing fractions is an essential operation that involves determining which fraction is larger or smaller. To compare fractions, follow these steps:
- Make sure the denominators are the same. If they are not, find the LCM of the two denominators.
- Compare the numerators while keeping the denominator the same.
- If the numerators are the same, the fractions are equal.
For example, let’s compare the fractions 3⁄4 and 2⁄4:
Since the denominators are the same, we can compare the numerators:
3 > 2
Therefore, 3⁄4 is greater than 2⁄4.
🤔 Note: When comparing fractions, make sure to line up the denominators and compare the numerators separately.
| Operation | Steps | Example |
|---|---|---|
| Addition | 1. Make sure denominators are the same. 2. Add numerators. 3. Simplify fraction. | 1/4 + 1/4 = 2/4 = 1/2 |
| Subtraction | 1. Make sure denominators are the same. 2. Subtract numerators. 3. Simplify fraction. | 3/4 - 1/4 = 2/4 = 1/2 |
| Multiplication | 1. Multiply numerators. 2. Multiply denominators. 3. Simplify fraction. | (1/2) × (3/4) = 3/8 |
| Division | 1. Invert second fraction. 2. Multiply fractions. 3. Simplify fraction. | (1/2) ÷ (3/4) = (1/2) × (4/3) = 4/6 = 2/3 |
| Comparison | 1. Make sure denominators are the same. 2. Compare numerators. 3. Determine which fraction is larger or smaller. | 3/4 vs. 2/4: 3 > 2, so 3/4 is greater than 2/4 |
In conclusion, mastering fraction operations is a crucial step in becoming proficient in mathematics. By understanding how to add, subtract, multiply, divide, and compare fractions, you will be able to tackle more complex mathematical problems with confidence.
What is the least common multiple (LCM) of two denominators?
+The least common multiple (LCM) of two denominators is the smallest number that both denominators can divide into evenly. For example, the LCM of 4 and 6 is 12.
How do I simplify a fraction?
+To simplify a fraction, divide both the numerator and denominator by the greatest common divisor (GCD). For example, the fraction 4⁄8 can be simplified to 1⁄2 by dividing both the numerator and denominator by 4.
What is the difference between multiplying and dividing fractions?
+Multiplying fractions involves multiplying the numerators and denominators separately, while dividing fractions involves inverting the second fraction and then multiplying. For example, (1⁄2) × (3⁄4) = 3⁄8, while (1⁄2) ÷ (3⁄4) = (1⁄2) × (4⁄3) = 4⁄6 = 2⁄3.