5 Ways to Calculate Percent of a Number
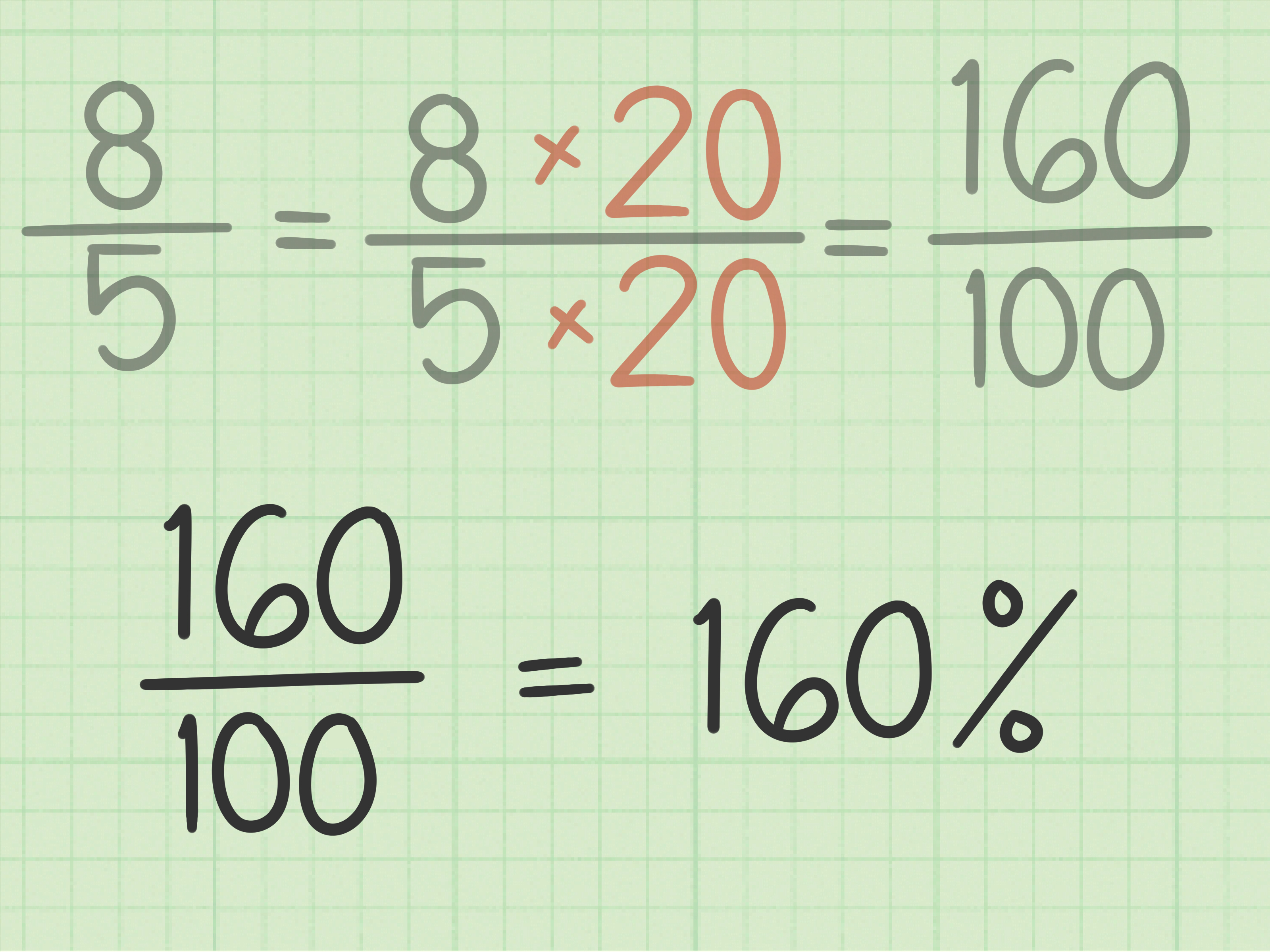
Calculating the percentage of a number is a fundamental mathematical operation that is frequently used in various aspects of life, including finance, science, and everyday problem-solving. There are multiple ways to calculate percentages, each with its own specific application and simplicity. Here are five methods to calculate percentages, each explained with examples to illustrate their use.
1. Basic Percentage Calculation
To find a percentage of a number, you multiply the number by the percentage, after converting the percentage to its decimal form by dividing by 100.
Example: Find 25% of 120.
- Convert 25% to a decimal: 25 ÷ 100 = 0.25
- Multiply 0.25 by 120: 0.25 × 120 = 30
So, 25% of 120 is 30.
2. Percentage Increase
To calculate the result of a percentage increase, you add the percentage value to the original number. This is particularly useful in scenarios where you’re calculating discounts, markups, or any form of increase.
Example: A shirt originally priced at $80 is on sale with a 15% discount. How much will you pay for the shirt?
- Calculate the discount amount: 15% of 80 = 0.15 × 80 = 12
- Subtract the discount from the original price: 80 - 12 = $68
You’ll pay $68 for the shirt.
3. Percentage Decrease
Similar to calculating an increase, but you subtract the percentage value from the original number. This method is used for calculating discounts, depreciation, or any form of decrease.
Example: A car valued at $20,000 depreciates by 10% each year. What’s its value after one year?
- Calculate the depreciation: 10% of 20,000 = 0.10 × 20,000 = 2,000
- Subtract the depreciation from the original value: 20,000 - 2,000 = $18,000
The car’s value after one year is $18,000.
4. Reverse Percentage Calculation
Sometimes, you might need to find the original number when you know the percentage and the result. This is done by dividing the result by the percentage in its decimal form.
Example: You have $36, which is 24% of an unknown number. What is that number?
- Convert 24% to a decimal: 24 ÷ 100 = 0.24
- Divide 36 by 0.24: 36 ÷ 0.24 = $150
The unknown number is $150.
5. Percentage Change Between Two Numbers
To find the percentage change from one number to another, you calculate the difference, divide by the original number, and then convert to a percentage.
Example: A stock price increases from 40 to 56. What’s the percentage increase?
- Calculate the difference: 56 - 40 = $16
- Divide the difference by the original price: 16 ÷ 40 = 0.4
- Convert to a percentage: 0.4 × 100 = 40%
The stock price increased by 40%.
Conclusion
Mastering how to calculate percentages is crucial for a wide range of applications, from personal finance to scientific calculations. Whether you’re dealing with increases, decreases, or simply finding a percentage of a number, understanding these methods can significantly simplify your calculations. By practicing and applying these techniques, you’ll find that calculating percentages becomes second nature, making it easier to tackle more complex problems.
What is the simplest way to calculate a percentage of a number?
+
The simplest way is to multiply the number by the percentage after converting the percentage to its decimal form.
How do you calculate a percentage increase or decrease?
+
For an increase, add the percentage value to the original number. For a decrease, subtract the percentage value from the original number.
What is reverse percentage calculation used for?
+
It’s used to find the original number when you know the percentage and the result. This is done by dividing the result by the percentage in its decimal form.
Related Terms:
- Decimal to percentage worksheet
- Percentage Worksheet Grade 6
- Percentage Worksheet grade 7 PDF
- Percentage word problems worksheet pdf